Write the linear equation such that each point on its graph has an ordinate 3 times its abscissa.
To solve the problem of writing a linear equation such that each point on its graph has an ordinate that is 3 times its abscissa, we can follow these steps:
1. Understanding the Terms:
- The abscissa refers to the x-coordinate of a point on the graph.
- The ordinate refers to the y-coordinate of a point on the graph.
- According to the problem, the ordinate (y) is 3 times the abscissa (x).
2. Setting Up the Equation:
- We can express this relationship mathematically as:
y=3x
3. Verifying with Points:
- Let's verify this equation with some points:
- If x=0, then y=3×0=0 → Point (0, 0)
- If x=1, then y=3×1=3 → Point (1, 3)
- If x=2, then y=3×2=6 → Point (2, 6)
- If x=3, then y=3×3=9 → Point (3, 9)
4. Conclusion:
- The points (0, 0), (1, 3), (2, 6), and (3, 9) all lie on the line represented by the equation y=3x.
- Therefore, the linear equation that satisfies the condition that the ordinate is 3 times the abscissa is:
y=3x
- The abscissa refers to the x-coordinate of a point on the graph.
- The ordinate refers to the y-coordinate of a point on the graph.
- According to the problem, the ordinate (y) is 3 times the abscissa (x).
- We can express this relationship mathematically as:
y=3x
- Let's verify this equation with some points:
- If x=0, then y=3×0=0 → Point (0, 0)
- If x=1, then y=3×1=3 → Point (1, 3)
- If x=2, then y=3×2=6 → Point (2, 6)
- If x=3, then y=3×3=9 → Point (3, 9)
- The points (0, 0), (1, 3), (2, 6), and (3, 9) all lie on the line represented by the equation y=3x.
- Therefore, the linear equation that satisfies the condition that the ordinate is 3 times the abscissa is:
y=3x
Topper's Solved these Questions
- LINEAR EQUATION IN TWO VARIABLESBook:NCERT EXEMPLAR ENGLISHChapter:LINEAR EQUATION IN TWO VARIABLESExercise:Exercise 4.4
Explore 7 Videos
- LINEAR EQUATION IN TWO VARIABLESBook:NCERT EXEMPLAR ENGLISHChapter:LINEAR EQUATION IN TWO VARIABLESExercise:Exercise 4.5
Explore 1 Video
- LINEAR EQUATION IN TWO VARIABLESBook:NCERT EXEMPLAR ENGLISHChapter:LINEAR EQUATION IN TWO VARIABLESExercise:Exercise 4.2
Explore 1 Video
- INTRODUCTION TO EUCLID GEOMETRYBook:NCERT EXEMPLAR ENGLISHChapter:INTRODUCTION TO EUCLID GEOMETRYExercise:Exercise 5.4 Long Answer Type Questions
Explore 5 Videos
- LINES AND ANGLES Book:NCERT EXEMPLAR ENGLISHChapter:LINES AND ANGLES Exercise:MULTIPLE CHOICE QUESTIONS
Explore 34 Videos
Similar Questions
Find the point on the parabola y2=18x at which ordinate is 3 times its abscissa.
View SolutionFind the point on the parabola y2=12x at which ordinate is 3 times its abscissa.
View SolutionA point whose abscissa is −3 and ordinate 2 lies in
View SolutionDetermine the point on the graph of the linear equation 2x+5y=19 whose ordinate is 112 times its abscissa.
View SolutionA point A is at a distance of √10 unit from the point (4,3). Find the co-ordinates of the point A, if its ordinate is twice its abscissa.
View SolutionThe following observed values of x and y are thought to satisfy a linear equation. Write the linear equation
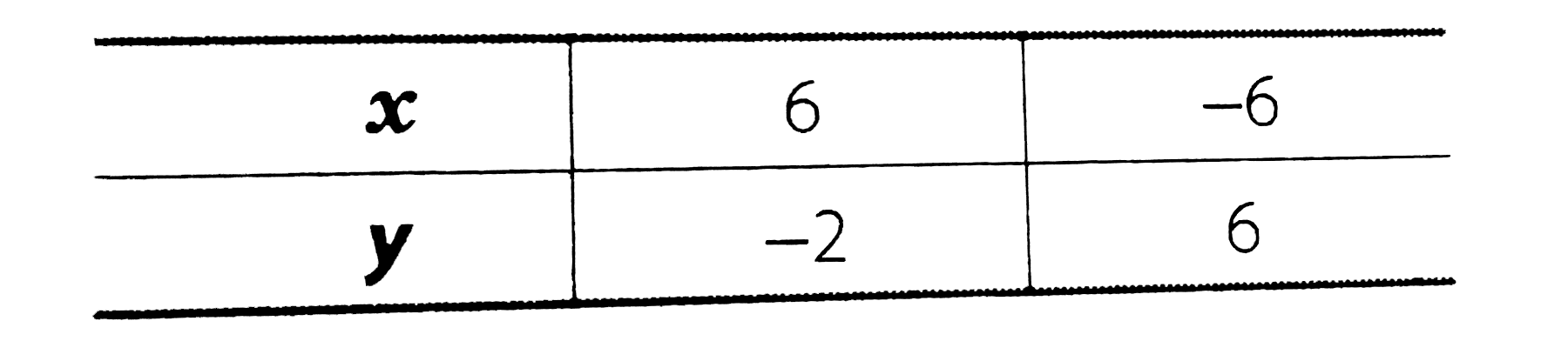
Draw the graph using the values of x, y as given in the above table.
At what points the graph of the linear equation
(i) cuts the X-axis ? (ii) cuts the Y-axis ?
View SolutionThe graph of the linear equation y=x passes through the point
View SolutionThe co-ordinates of a point on the parabola y2=8x whose ordinate is twice of abscissa, is :
View SolutionA curve passes through the point (5,3) and at any point (x,y) on it, the product of its slope and the ordinate is equal to its abscissa. Find the equation of the curve and identify it.
View SolutionUse the graph given alongside, to find the co-ordinate of the point (s) satisfying the given condition:
the ordinate is half of the abscissa.
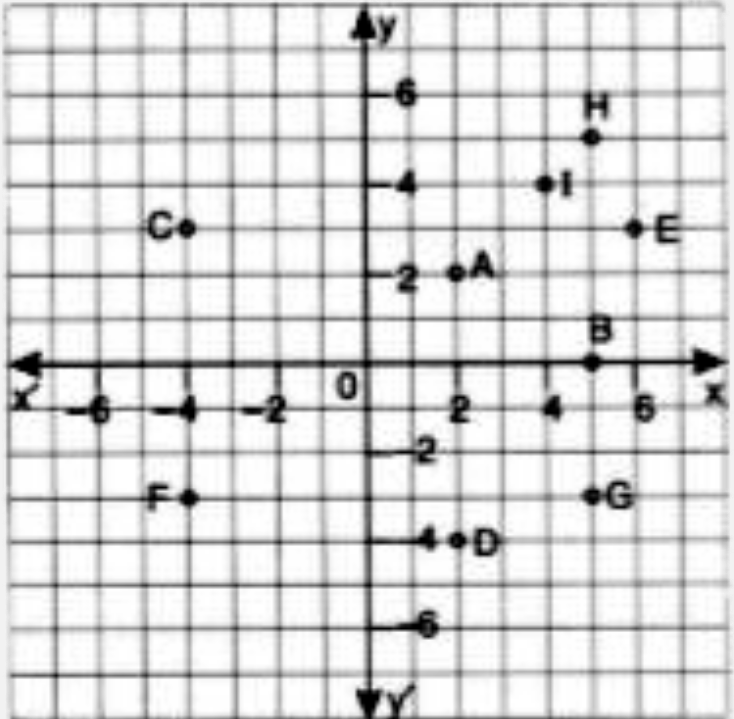
View Solution