Given that ΔH=0 for mixing of two gases. Explain whether the diffusion of these gases into each other in a closed container is a spontaneous process or not ?
To determine whether the diffusion of two gases into each other in a closed container is a spontaneous process given that ΔH = 0 for mixing, we can follow these steps:
1. Understand the Concept of Spontaneity:
- A process is considered spontaneous if it occurs without needing to be driven by an external force. The spontaneity of a process is determined by the change in Gibbs free energy (ΔG).
2. Gibbs Free Energy Equation:
- The relationship between Gibbs free energy, enthalpy, and entropy is given by the equation:
ΔG=ΔH−TΔS
- Here, ΔG is the change in Gibbs free energy, ΔH is the change in enthalpy, T is the absolute temperature, and ΔS is the change in entropy.
3. Substituting Given Values:
- In this case, we are given that ΔH = 0 for the mixing of the two gases. Substituting this into the Gibbs free energy equation gives:
ΔG=0−TΔS
ΔG=−TΔS
4. Analyzing the Temperature:
- The temperature (T) is always a positive value in thermodynamic equations. Therefore, the sign of ΔG will depend on the sign of ΔS.
5. Understanding Entropy (ΔS):
- When two gases mix, the randomness or disorder of the system increases. This increase in disorder corresponds to a positive change in entropy (ΔS > 0).
6. Conclusion on Spontaneity:
- Since ΔS is positive, we can conclude:
ΔG=−TΔS<0
- A negative ΔG indicates that the process is spontaneous.
7. Final Statement:
- Therefore, the diffusion of the two gases into each other in a closed container is indeed a spontaneous process.
- A process is considered spontaneous if it occurs without needing to be driven by an external force. The spontaneity of a process is determined by the change in Gibbs free energy (ΔG).
- The relationship between Gibbs free energy, enthalpy, and entropy is given by the equation:
ΔG=ΔH−TΔS
- Here, ΔG is the change in Gibbs free energy, ΔH is the change in enthalpy, T is the absolute temperature, and ΔS is the change in entropy.
- In this case, we are given that ΔH = 0 for the mixing of the two gases. Substituting this into the Gibbs free energy equation gives:
ΔG=0−TΔS
ΔG=−TΔS
- The temperature (T) is always a positive value in thermodynamic equations. Therefore, the sign of ΔG will depend on the sign of ΔS.
- When two gases mix, the randomness or disorder of the system increases. This increase in disorder corresponds to a positive change in entropy (ΔS > 0).
- Since ΔS is positive, we can conclude:
ΔG=−TΔS<0
- A negative ΔG indicates that the process is spontaneous.
- Therefore, the diffusion of the two gases into each other in a closed container is indeed a spontaneous process.
Topper's Solved these Questions
Similar Questions
The molecules in gases frequently collide with each other and the sides of the container in which they are kept.
View SolutionThere are two closed containers A and B. Container A contains PCl5 gas at 25∘C temperature and 1 bar pressure. Container B contains equilibrium mixture of gases obtained by partial decomposition of PCl5 (g) at the same temperature and pressure. Assuming that initially equal mole of PCl5 (g) at same temperature and pressure . Assuming that initially equal moles of PCl5 were taken into the containers ( before decomposition ), the gases are allowed to effuse through a pinhole in the containers, Which container will show higher rate of effusion ? Justify .
View SolutionThe rates of diffusion of two gases A and B are in the the ratio 1:4 A mixture contains these gases A and B in the ratio 2:3 The ratio of mole fraction of the gases A and B in the mixture is (assume that PA=PB) .
View SolutionP-V plots for two gases during an adiabatic process are given in the figure:
Plot A and plot B should correspond to :
View SolutionTwo bolcks to the same metal having same mass and at temperature T1 and T2 respectively ,are brought in contact with each other and allowed to attain thermal equilibrium at constant pressure .The change in entropy ,ΔS for this process is :
View SolutionTwo bolcks to the same metal having same mass and at temperature T1 and T2 respectively ,are brought in contact with each other and allowed to attain thermal equilibrium at constant pressure .The change in entropy ,ΔS for this process is :
View SolutionState which type of chemical reactions proceed with - a. Evolution of heat energy b. Absorption of heat energy. State in each of the following reactions whether heat is evolved or absorbed - i. Water is added to quicklime ii. Two neutral gases on passage through an electric arc give niric oxide iii. Two neutral gases combine to give - a basic gas.
View SolutionConsider the adjacent figure. A piston divides a cylindrical container into two equal parts. The lets part contains 1 mole of helium gas and the right part contains two moles or oxygen gas. The initial temperatures and pressures of the gases in the left chamber are T0 and p0 and the right chamber are T02 and p0 respectively as shown in the figure. The piston as well as the walls of the container are adiabatic. After removal of the piston, gases mix homoganeously and the final pressure becomes p=4n13p0. Find the value of n.
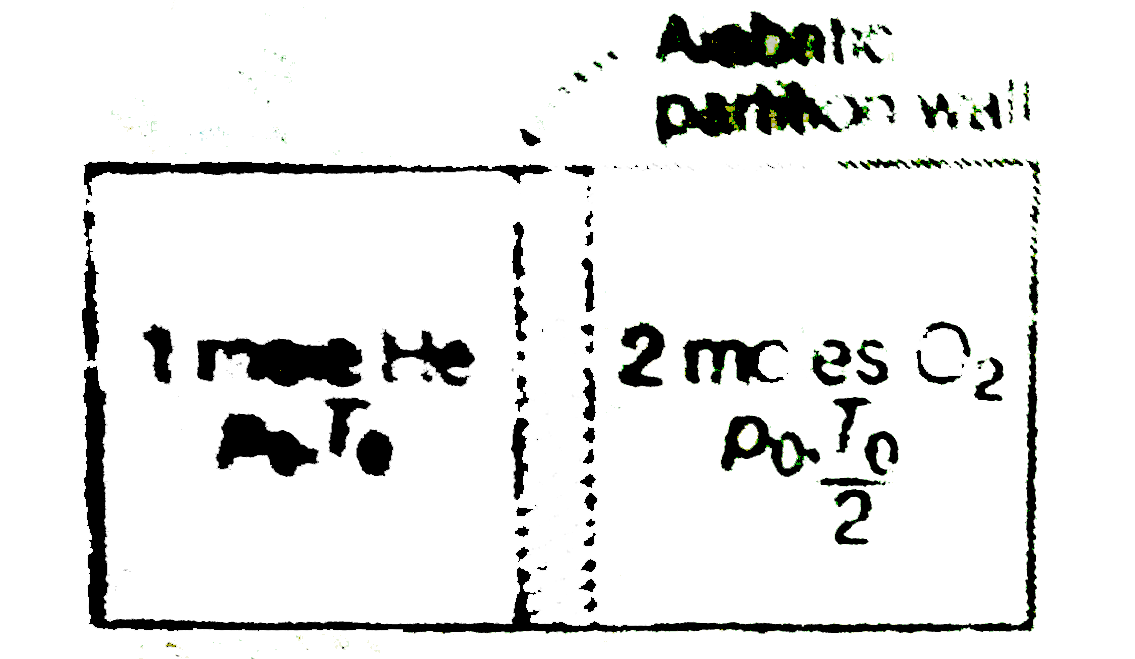
View SolutionTwo gases occupy two containers (A) and (B). The gas in (A) of volume 0.11m3 experts a pressure of 1.38Mpa. The gas in (B) of volume 0.16m3 experts a pressure of 0.69 Mpa. Two containers are united by a tube of negligible volume and the gases are allowed to intermingle. What is the final pressure in the container if the temperature remains constant ?
View SolutionOne of the exterior angles of a triangle is 800 , and the interior opposite angles are equal to each other. What is the measures of each of these two angles?
View Solution
