Give examples of a one-dimensional motion where
(a) the particle moving along positive x-direction comes to rest periodically and forward.
(b) the particle moving along positive x-direction comes to rest periodically and moves backward..
Step-by-Step Text Solution
Part (a): Example of One-Dimensional Motion Where the Particle Comes to Rest Periodically and Moves Forward
1. Define the Position Function:
We can define the position of the particle as a function of time, x(t)=t−sin(t).
2. Find the Velocity:
The velocity v(t) is the derivative of the position function with respect to time:
v(t)=dxdt=1−cos(t)
3. Determine When the Particle Comes to Rest:
The particle comes to rest when the velocity is zero:
1−cos(t)=0⟹cos(t)=1
This occurs at t=2nπ where n is an integer.
4. Check the Position at Rest Points:
- At t=0:
x(0)=0−sin(0)=0
- At t=2π:
x(2π)=2π−sin(2π)=2π
This shows that the particle moves forward along the positive x-direction and comes to rest periodically.
Part (b): Example of One-Dimensional Motion Where the Particle Comes to Rest Periodically and Moves Backward
1. Define the Position Function:
We can define the position of the particle as x(t)=sin(t).
2. Find the Velocity:
The velocity v(t) is the derivative of the position function:
v(t)=dxdt=cos(t)
3. Determine When the Particle Comes to Rest:
The particle comes to rest when the velocity is zero:
cos(t)=0
This occurs at t=π2+nπ where n is an integer.
4. Check the Acceleration at Rest Points:
The acceleration a(t) is the derivative of the velocity:
a(t)=−sin(t)
- At t=π2:
a(π2)=−sin(π2)=−1(negative acceleration)
This indicates that after coming to rest, the particle moves backward.
We can define the position of the particle as a function of time, x(t)=t−sin(t).
2. Find the Velocity:
The velocity v(t) is the derivative of the position function with respect to time:
v(t)=dxdt=1−cos(t)
The particle comes to rest when the velocity is zero:
1−cos(t)=0⟹cos(t)=1
This occurs at t=2nπ where n is an integer.
- At t=0:
x(0)=0−sin(0)=0
- At t=2π:
x(2π)=2π−sin(2π)=2π
This shows that the particle moves forward along the positive x-direction and comes to rest periodically.
We can define the position of the particle as x(t)=sin(t).
The velocity v(t) is the derivative of the position function:
v(t)=dxdt=cos(t)
The particle comes to rest when the velocity is zero:
cos(t)=0
This occurs at t=π2+nπ where n is an integer.
The acceleration a(t) is the derivative of the velocity:
a(t)=−sin(t)
- At t=π2:
a(π2)=−sin(π2)=−1(negative acceleration)
This indicates that after coming to rest, the particle moves backward.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - Multiple Choice Question (More Than One Qns)
6 Videos
- MOTION IN A PLANEBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Multiple Choice Questions
37 Videos
- OSCILLATIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - OSCILLATIONSEXERCISE - All Questions
36 Videos
Similar Questions
The position of a particle moving along x-axis varies eith time t as x=4t−t2+1. Find the time interval(s) during which the particle is moving along positive x-direction.
View SolutionPosition-time graph for a particle moving along x− direction is as shown in the figure. Average speed of the particle from t=0 to t=4 is :

View SolutionA particle of mass m moving with speed v in position x - direction collides perfectly inelastically with another identical particle moving with same speed in positive y - direction . Find final velocity of the combination.
View SolutionA particle located at position x=0, at time t=0, starts moving along the positive x-direction with a velocity v2=αx (where alpha is a positive constant). The displacement of particle is proportional to
View SolutionA positively charged particle moving along x-axis with a certain velocity enters a uniform electric field directed along positive y-axis. Its
View SolutionAcceleration vs time graph is shown in the figure for a particle moving along a straight line. The particle is initially at rest. Find the time instant(s) when the particle comes to rest?
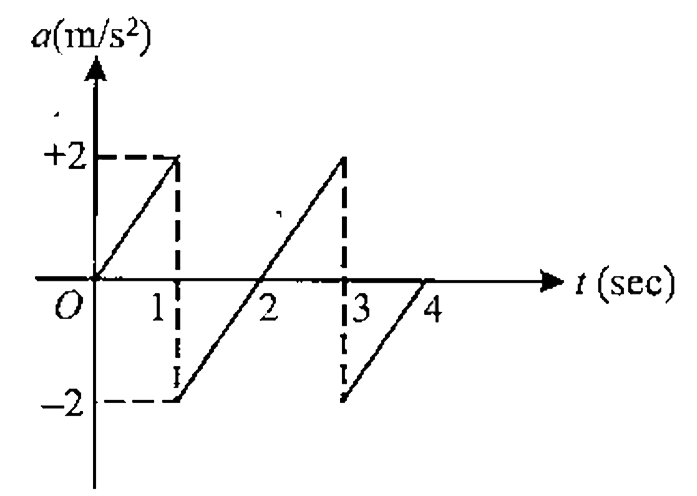
View SolutionA particle is moving along the curve x=at2+bt+c. If ac=b2, then particle would be moving with uniform
View SolutionThe velocity of a particle moving in the positive direction of the X-axis varies as V=K√S where K is a positive constant. Draw V-t graph.
View SolutionThe velocity of a particle moving in the positive direction of x-axis veries as v=10√x. Assuming that at t=0, particle was at x=0
View SolutionThe velocity –position graph of a particle moving along a straight line is shown. Then acceleration of the particle at s = 15 m is :
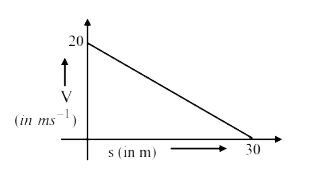
View Solution
