The human eye has an approximate angular resolution of ϕ=5.8×104 rad and a typical photo printer prints a minimum of 300 dpi (dots per inch, 1 inch = 2.54 cm). At what minimal distance z should a printed page be held so that one does not see the individual dots.Solution in Gujrati
( S1 and S2 are two consecutive dots on the paper)
As shown in the figure, suppose images of two consecutive dots S1 and S2 (on he paper at distance Z from the eye) can be seen clear and just separated by an eye. Here distance dm between S2 and S2 is called "linear limit of resolution" of an eye and angle αmin=ϕ is called "angular limit of resolution" of an eye.
Now as per the definition of measurement of angle in radian,
angle =arcradius
∴αmin=dmR
or ϕ=dmZ
∴Z=dmϕ
∴Z=(2.54cm300)5.8×10−4
∴Z=2543×5.8=14.6cm
If the paper is kept at a distance greater than 14.6 cm then images of S1 and S2 can not be seen clear and separated. Hence required minimum distance is Z = 14.6 cm.
Topper's Solved these Questions
- WAVE OPTICSBOOK - KUMAR PRAKASHANCHAPTER - WAVE OPTICSEXERCISE - SECTION-C NCERT Exemplar Solution (Short Answer Type Questions )
3 Videos
- WAVE OPTICSBOOK - KUMAR PRAKASHANCHAPTER - WAVE OPTICSEXERCISE - SECTION-C NCERT Exemplar Solution (Long Answer Type Questions)
5 Videos
- WAVE OPTICSBOOK - KUMAR PRAKASHANCHAPTER - WAVE OPTICSEXERCISE - SECTION-C NCERT Exemplar Solution (Multiple Choice Questions (More than One Options) )
4 Videos
- SEMICONDUCTOR ELECTRONICS : MATERIALS, DEVICES AND SIMPLE CIRCUITS BOOK - KUMAR PRAKASHANCHAPTER - SEMICONDUCTOR ELECTRONICS : MATERIALS, DEVICES AND SIMPLE CIRCUITS EXERCISE - Section-D : Multiple Choice Questions (MCQs) (MCQs asked in Competitive Exams)
129 Videos
Similar Questions
Can reflection result in plane polarised light if the light is incident on the interface from the side with higher refractive index ?
View Solutionif the intensity of the principal maximum in the single slit Fraunhoffer diffraction pattern, then the intensity when the slit width is double will be .....
View Solution
Truth table for the given circuit (See figure) is
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionThe human eye has an approximate angular resolution of ϕ=5.8×10−4 rad and a typical photo printer prints a minimum of 300 dpi (dots per inch, =2.54cm). Aminimum distance 'z' should a printed page be held so that one doesnot see the indivdual dots is ______ .
View Solution1 फुट ( foot) को मीटर (m ) में व्यक्त करे । ( 1 इंच = 2.54 cm)
View SolutionA typical 3-D printer creates object by laying down layers of ink one on top of another. The average thckness of layer depends on the caliber of the printer. For one particular 3-D printer, the average thickness of a layer is 102μm (micrometers). When creating a 1-inch cube, the printer takes 6 second to lay down each layer of ink. Approximately how long should it take this printer to create a 1-inch cube? (There are 1,000,000 micrometers in a meter, and 1 inch is approximately equal to 0.0254 meters.)
View Solution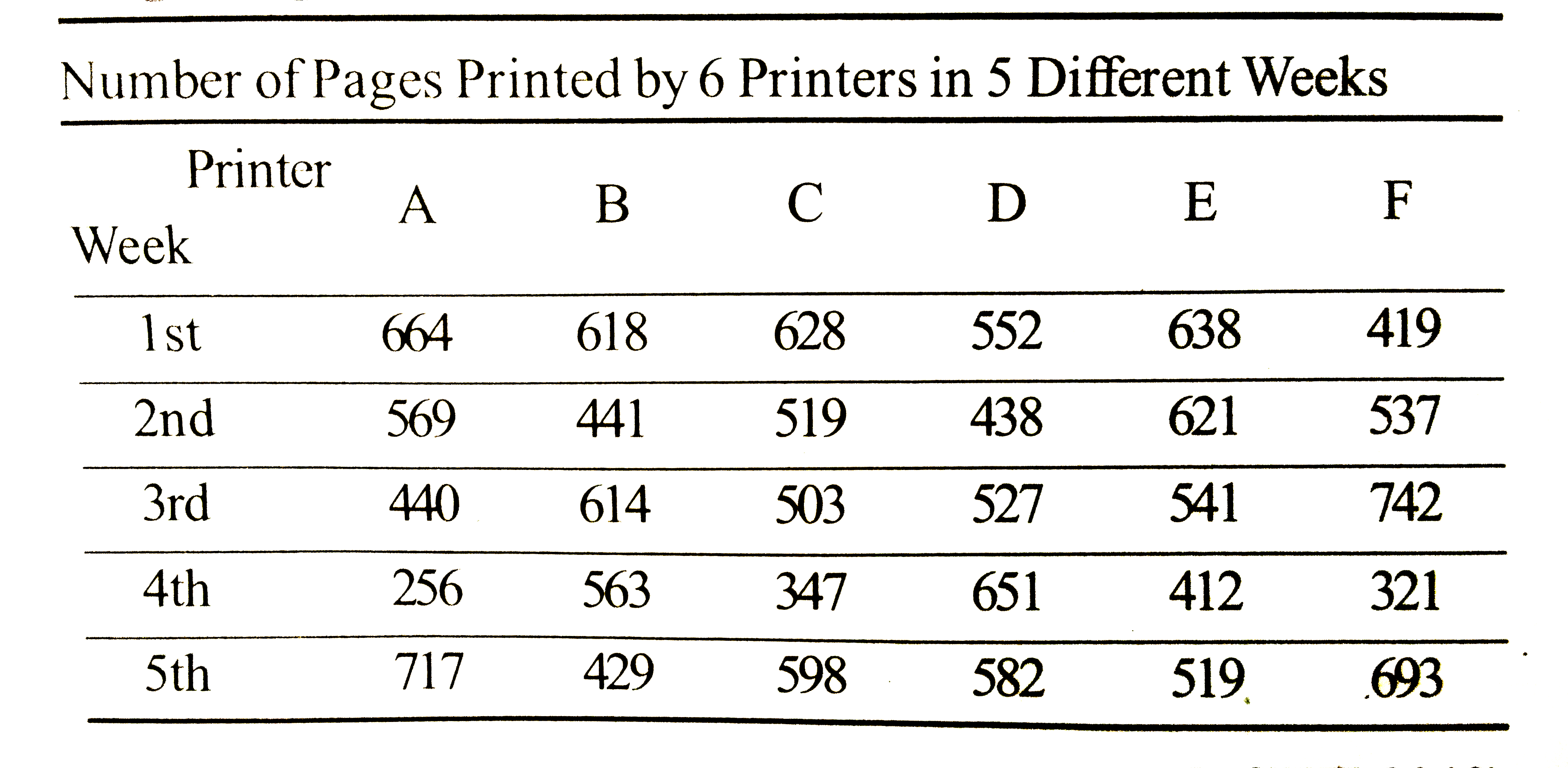
What is the respective ratio between the number of pages printed by Printer B in 2nd week and the number pages printed by Printer F in 5th week ?
View Solution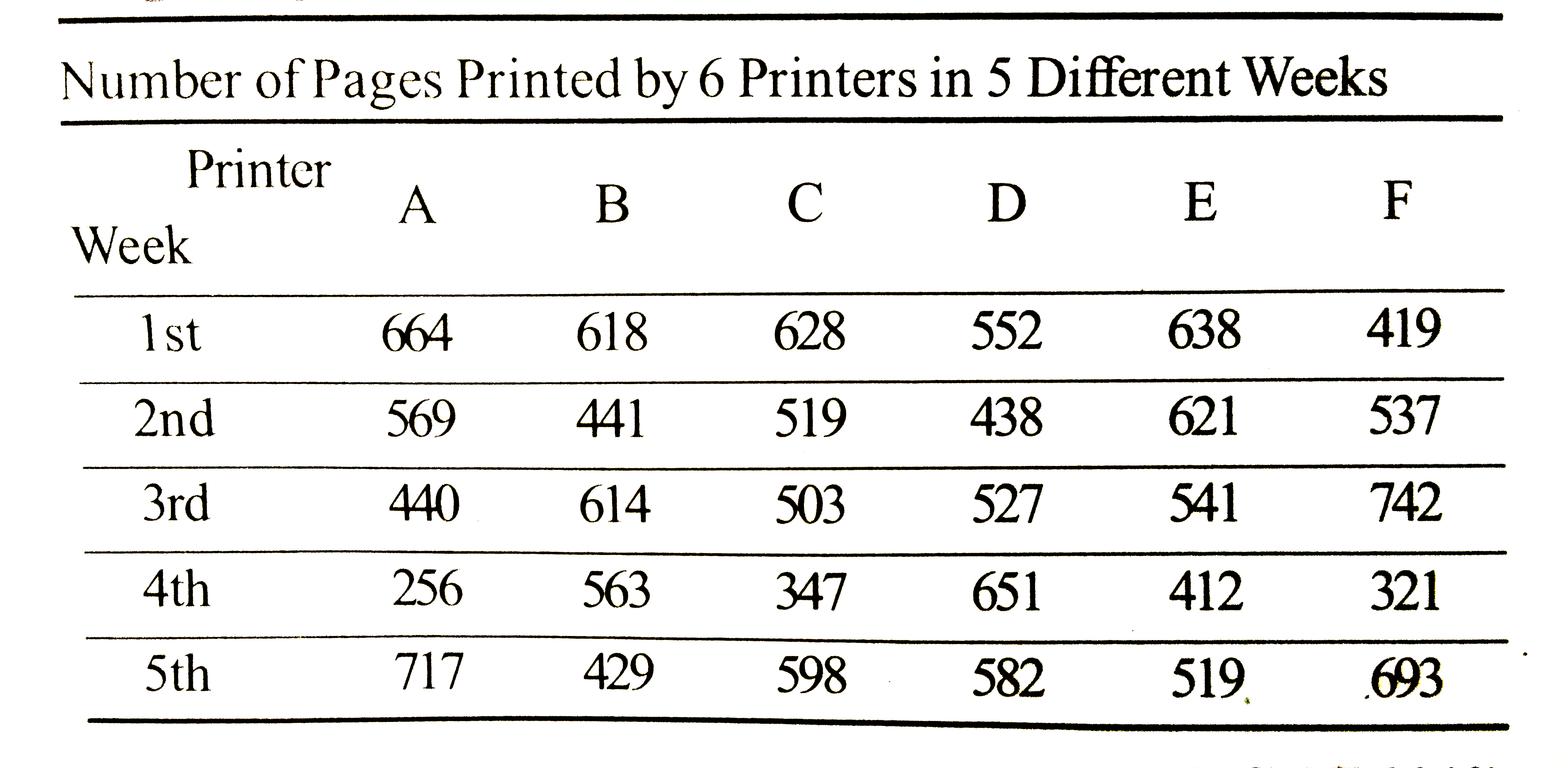
What is the avrage number of pages printed by all the given printers in 4th week?
View SolutionA rectangular page is to contain 24cm2 of print. The margins at the top and bottom of the page are 1.5 cm and the margins at other sides of the page is 1 cm. What should be the dimensions of the page so that the area of the paper used is minimum.
View SolutionThe height of a child is 4 feet and 2 inches. This height may be noted in cm as ________ . ( 1 inch = 2.54 cm)
View SolutionThe human eye has an approximate angular resolution of ϕ=5.8×10−4 rad and a typical photprinter prints a minimum of 300 dpi (dots per inch = 2.54 cm). At what minimal distance z should a printed page be held so that one does not see the individual dots ?
View Solutionदो तारो के कोणीय पृथकन का न्यूनतम मान निकाले जिन्हे 200cm द्वारक के दूरदर्शक द्वारा विभेदित किया जा सकता है। यदि मनुष्य की आँख का द्वारक 2mm हो और नैतृका की फोकस दुरी 1 इंच हो, तो अभिदृश्यक की न्यूनतम फोकस दुरी कितनी होनी चाहिए कि दूरदर्शक की पूर्ण विभेदन क्षमता प्रयुक्त हो सके ? λ=5500Å ले।
View Solutionमानव आँख का कोणीय विभेदन (Angular resolution) लगभग ϕ=5.8×10−4 rad है तथा एक सामान्य फोटोप्रिन्टर न्यूनतम 300 dpi (dots per inch, 1 इंच = 2.54cm) छापता है। किस न्यूतनम दूरी z पर छापे हुए पृष्ठ को रखना चाहिए ताकि अलग-अलग बिन्दुएं दिखाई न पड़े।
View SolutionThe human eye has an approximate angular resolution of ϕ=5.8×10−4 rad and a typical photo printer prints a minimum of 300 dpi (dots per inch, =2.54cm). Aminimum distance 'z' should a printed page be held so that one doesnot see the indivdual dots is ______ .
View Solution
