Prove Bernoulli 's Principle . OR Obtain Bernoulli's equation for steady incompressible irrotational and viscous liquid.Solution in Gujrati

Video Solution

Text Solution

Verified by Experts

A pipe is shown in figure.
At point B of left end of pipe Fluid speed $= v_{1}$
Cross sectional area $= A_{1}$ .
Pressure exerted on fluid $P_{1} = \frac{F_{1}}{A_{1}}$ .

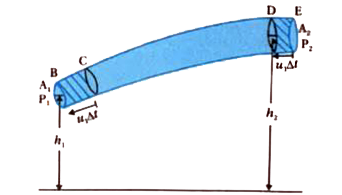
At point D , of right end of pipe ,
Fluid speed $= v_{2}$
Cross esctional area $= A_{2}$
Pressure exerted on the fluid $P_{2} = \frac{F_{2}}{A_{2}}$ .
Force $F_{1} = P_{1} A_{1}$ exerted on fluid at point B, covers distance $v_{1} Δ t$ and reaches at point C. the work done on fluid
$W_{1} =$ (Force)(displacement)
$W_{1} = P_{1} A_{1} v_{1} Δ t$
$∴ W_{1} = P_{1} (Δ V_{1})$ ....(1)
where $Δ V = A_{1} v_{1} Δ t =$ volume of fluid)
Fluid at point d, covers distance $v_{2} Δ t$ and reaches at point E, the work done by the fluid.
$W_{2} = - P_{2} A_{2} v_{2} Δ t$
$∴ W_{2} = - P_{2} Δ V$ ....(2)
where $Δ V = A_{2} v_{2} Δ t$ = volume of fluid)
According to equation of continuity both fluids have same volume.
Total work done on the fluid
$W = W_{1} + W_{2}$
$W = P_{1} Δ V - P_{2} Δ V$
$W = (P_{1} - P_{2}) Δ V$ ....(3)
Part of this work goes into changing the kinetic energy of the fluid and part goes into changing the gravitational potential energy.
$W = Δ K + Δ U$ .... (4)
The mass flowing fluid of density $ρ$ at time $Δ t$ .
$Δ m = Volume \times Density$
$Δ m = ρ (Δ V)$ ...(5)
This liquid when moves from B to D its velocity becomes $v_{1}$ to $v_{2}$ . Hence change in its kinetic energy
$Δ K = \frac{1}{2} m (v_{2}^{2} - v_{1}^{2})$
$Δ K = \frac{1}{2} ρ Δ V (v_{2}^{2} - v_{1}^{2})$ .....(6)
When fluid of mass $Δ m$ move from B to D , it posses height from $h_{1}$ to $h_{2} (h_{2} > h_{1})$ . Hence its potential energy is botained as below
$Δ U = m g (h_{2}) - m g (h_{1})$
$Δ U = m g (h_{2} - h_{1})$
$Δ U = (ρ Δ V) g (h_{2} - h_{1})$ ....(7)
The values of W , $Δ K, Δ U$ in equation (4) from equation (3),(6),(7)
$(P_{1} - P_{2}) Δ V = \frac{1}{2} ρ Δ V (v_{2}^{2} - v_{1}^{2}) + (ρ Δ V) g (h_{2} - h_{1})$
Dividing each by $Δ V$
$P_{1} - P_{2} = \frac{1}{2} ρ (v_{2}^{2} - v_{1}^{2}) + ρ g h_{2} - h_{1})$
$∴ P_{1} - P_{2} = \frac{1}{2} ρ v_{2}^{2} - \frac{1}{2} ρ v_{1}^{2} + ρ g h_{2} - ρ g h_{1}$
$∴ P_{1} + \frac{1}{2} ρ_{1}^{2} + ρ g h_{1} = P_{2} + \frac{1}{2} ρ v_{2}^{2} + ρ g h_{2}$ ....(8)
Equation (8) is called Bernoulli.s equation In general
$P + \frac{1}{2} ρ v^{2} + ρ g h =$ constant ....(9)
In words the Bernoulli.s equation may be stated as follows :
As moving along a streamline the sum of the pressure (P) , the kinetic energy per unit volume
$(\frac{1}{2} ρ v^{2})$ and the potential energy per unit volume $(ρ g h)$ remains a constant.

|

Updated on:21/07/2023

Class 11 PHYSICS PROPERTIES OF SOLIDS AND FLUIDS

Topper's Solved these Questions

Similar Questions

KUMAR PRAKASHAN-MECHANICAL PROPERTIES OF FLUIDS -QUESTION PAPER (SECTION -C)

Home
Ask Doubt
Profile