State and prove Pascal's law.Solution in Gujrati
Law : The pressure in a fluid at rest is the same at all points
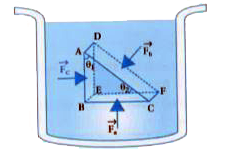
Proof of Pascal.s law. ABC-DEF is an element of the interior of a fluid at rest , This element is in the form of a right angled prism . The element is small so that the effect of gravity can be ignored, but it has been enlarged for the sake of clarity .
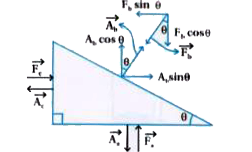
Figure shows an element in the interior of a fluid at rest . This element ABC- DEF is in the form of a right - angled prism.
Area of surface ADFC is Ab. The force on this surface is Fb and hence pressure is Pb.
Area of surface BEFC is Aa . The perpendicular force on this surface is Fa and hence pressure is Pa.
Area of surface ABCD is Ac .The perpendicular force on this surface is Fc and hence pressure is Pc
Fa=Fbcosθ (For equilibrium position)
Aa=Abcosθ (by geometry )and
Fc=Fbsinθ (for equilibrium position)
Ac=Absinθ (by geometry)
The pressures on rectangular surfaces are as below as shown in figure.
Pb=FbAb ....(1)
Pa=FaAa=FbcosθAbcosθ=FbAb ....(2)
Pc=FcAc=FbsinθAbsinθ=FbAb ....(3)
It is clear from equation 1,2 and 3
Pa=Pb=Pc .... (4)
Equation (4) shows that pressure exerted is same in all directions is a fluid at rest .
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDS BOOK - KUMAR PRAKASHANCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - SECTION-A Try Yourself (VSQs)
132 Videos
- MECHANICAL PROPERTIES OF FLUIDS BOOK - KUMAR PRAKASHANCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - SECTION - B Numericals
29 Videos
- MACHANICAL PROPERTIES OF SOLIDS BOOK - KUMAR PRAKASHANCHAPTER - MACHANICAL PROPERTIES OF SOLIDS EXERCISE - QUESTION PAPER
11 Videos
- MOTION IN A STRAIGHT LINE BOOK - KUMAR PRAKASHANCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - QUESTION PAPER
11 Videos
Similar Questions
What is fluid ?
View SolutionA 14.5 kg mass, fastened to the end of a steel wire of unstretched 1.0 m, is whirled in a vertical circle with an angular velocity of 2 rev/s at the bottom of the circle. The cross- sectional area of the wire is 0.065 cm2. Calculate the elongation of the wire when the mass is at the lowest point of its path.
View SolutionWhat is called brittle material ?
View SolutionDefine frame of reference and give its types.
View SolutionState Pascal's law. How can it be demonstrated?
View SolutionState the law of transmission of pressure (Pascal's Law) in fluids.
View SolutionState Pascal's law and verify it with the help of an experiment.
View SolutionState and prove Pascal's law in fluids.
View SolutionState Pascal's law.
View SolutionState Pascal's Law .
View SolutionState Pascal's law in fluids.
View SolutionState and prove Pascal's law in fluids.
View SolutionState Pascal's lae in fluids.
View SolutionState and prove Pascal's law in fluids.
View SolutionState Pascal's lae in fluids.
View Solution
