Area under power-time curve gives total work done
by the body only
on the body only
Both (a) and (b)
neither (a) nor (b)
The correct Answer is:C
To solve the question "Area under power-time curve gives total work done," we will analyze the relationship between power, work, and the area under the curve in a step-by-step manner.
1. Understanding Power and Work:
- Power (P) is defined as the rate at which work (W) is done. Mathematically, this is expressed as:
P=dWdt
- This means that the instantaneous power is the derivative of work with respect to time.
2. Relating Power to Work:
- Rearranging the equation gives us:
dW=P⋅dt
- This indicates that an infinitesimal amount of work done (dW) over an infinitesimal time interval (dt) is equal to the power multiplied by that time interval.
3. Integrating to Find Total Work:
- To find the total work done over a time interval, we integrate the power with respect to time:
W=∫Pdt
- The result of this integration gives us the total work done over the specified time interval.
4. Interpreting the Area Under the Curve:
- In a power-time graph, the area under the curve represents the integral of power with respect to time. Thus:
Area under the power-time curve=W
- Therefore, the area under the power-time curve indeed gives us the total work done.
5. Determining the Type of Work Done:
- The question further asks whether the work done is "by the body" or "on the body."
- If the area under the curve is positive, it indicates that work is done on the body (meaning energy is supplied to the body).
- If the area is negative, it indicates that work is done by the body (meaning the body is doing work against an external force).
6. Conclusion:
- Since the area under the power-time curve can be either positive or negative depending on the situation, the correct answer to the question is that the area can represent both types of work depending on its sign.
- Therefore, the answer is option 3: both a and b.
- Power (P) is defined as the rate at which work (W) is done. Mathematically, this is expressed as:
P=dWdt
- This means that the instantaneous power is the derivative of work with respect to time.
- Rearranging the equation gives us:
dW=P⋅dt
- This indicates that an infinitesimal amount of work done (dW) over an infinitesimal time interval (dt) is equal to the power multiplied by that time interval.
- To find the total work done over a time interval, we integrate the power with respect to time:
W=∫Pdt
- The result of this integration gives us the total work done over the specified time interval.
- In a power-time graph, the area under the curve represents the integral of power with respect to time. Thus:
Area under the power-time curve=W
- Therefore, the area under the power-time curve indeed gives us the total work done.
- The question further asks whether the work done is "by the body" or "on the body."
- If the area under the curve is positive, it indicates that work is done on the body (meaning energy is supplied to the body).
- If the area is negative, it indicates that work is done by the body (meaning the body is doing work against an external force).
- Since the area under the power-time curve can be either positive or negative depending on the situation, the correct answer to the question is that the area can represent both types of work depending on its sign.
- Therefore, the answer is option 3: both a and b.
Topper's Solved these Questions
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - MISCELLANEOUS QUESTIONSEXERCISE - PART-B99videos
MISCELLANEOUS QUESTIONS
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - MEASUREMENTEXERCISE - ELEMENTARY QUESTIONS (HIGHER ORDER THINKING QUESTIONS)13videos
MEASUREMENT
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - MOTIONEXERCISE - HIGHER ORDER THINKING QUESTIONS48videos
MOTION
Similar Questions
Knowledge Check
- Question 1 - Select One
Area under speed-time curve gives
AdistanceBvelocityCaccelerationDnone of these - Question 2 - Select One
The area under the given curve is
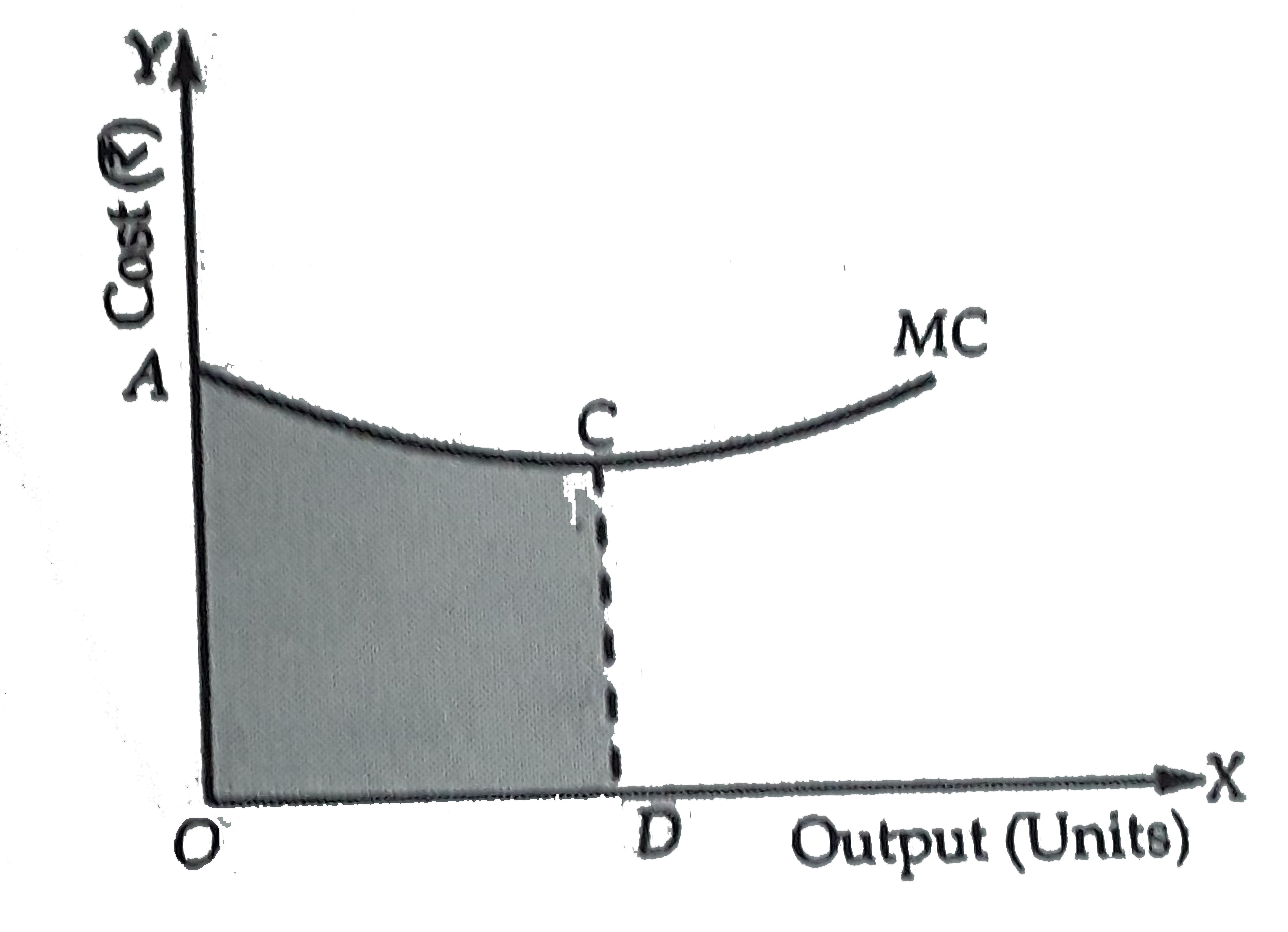 ATCBTVCCACDAVC
ATCBTVCCACDAVC - Question 3 - Select One
Area under marginal cost curve is
ATVCBTFCCAFCDAVC