If f is focal length of the lens, then the power of a lens is equal to
100f(cm)
10f(cm)
100f(m)
1100f(cm)
The correct Answer is:A
To find the power of a lens given its focal length (f), we can follow these steps:
1. Understand the Relationship: The power (P) of a lens is defined as the reciprocal of its focal length (f) measured in meters. The formula is:
P=1f(in meters)
2. Convert Focal Length to Meters: If the focal length is given in centimeters, we need to convert it to meters since the power is defined in diopters (D), where 1 D = 1/m. The conversion is:
f(in meters)=f(in cm)100
3. Substitute in the Power Formula: Now, substituting the converted focal length into the power formula:
P=1(f(in cm)100)=100f(in cm)
4. Conclusion: Therefore, the power of the lens in terms of its focal length in centimeters is:
P=100f(in cm)
P=1f(in meters)
f(in meters)=f(in cm)100
P=1(f(in cm)100)=100f(in cm)
P=100f(in cm)
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - MCGROW HILL PUBLICATIONCHAPTER - REFRACTION OF LIGHTEXERCISE - HIGHER ORDER THINKING QUESTIONS
37 Videos
- REFLECTION OF LIGHT BOOK - MCGROW HILL PUBLICATIONCHAPTER - REFLECTION OF LIGHT EXERCISE - HIGHER ORDER THINKING QUESTIONS
10 Videos
- SOURCES OF ENERGY BOOK - MCGROW HILL PUBLICATIONCHAPTER - SOURCES OF ENERGY EXERCISE - ELEMENTARY QUESTIONS
82 Videos
Similar Questions
Knowledge Check
An object is placed in front of a converging lens at a distance equal to twice the focal length f1 of the lens. On the other side of the lens is a concave mirror of focal length f2 separated from the lens is a concave mirror of focal length f2 separated from the lens by a distance 2(f1+f2). Light from the object passes rightward through the lens, reflects from the mirror, passes leftward throught the lens, and forms a final image of the object.
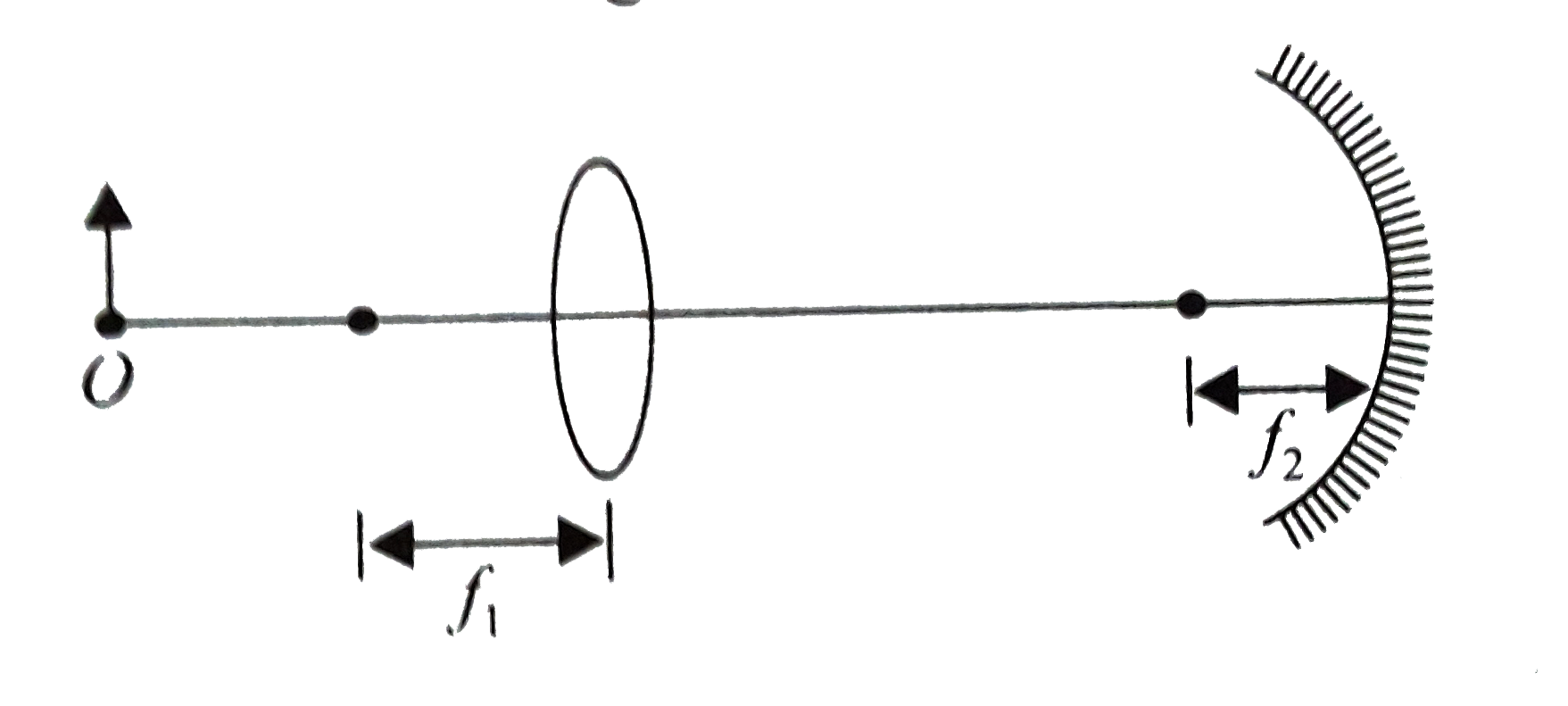 AThe distance between the lens and the final image is equal to 2f1.BThe distance between the lens and the final image is equal to 2(f1+f2)CThe final image is real, inverted and of same size as that fo the object.DThe final image is real, erect and of same size as that of the object.
AThe distance between the lens and the final image is equal to 2f1.BThe distance between the lens and the final image is equal to 2(f1+f2)CThe final image is real, inverted and of same size as that fo the object.DThe final image is real, erect and of same size as that of the object.If the focal length of the biconvex lens is 25 cm, then the
power of the lens will beAa + 4 dioptreBb - 4 dioptreCc +0.04 dioptreDd -0.04 dioptreA focal length of a lens 10cm. What is power of a lens in dipotre?
A0.1DB10DC15DD1D
