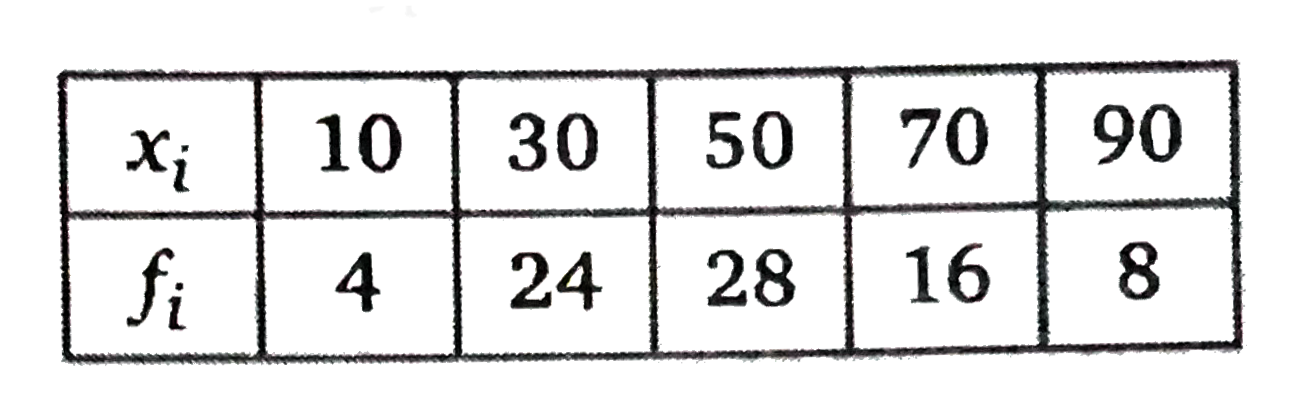
Let the data, 4, 10, x, y, 27 be in the increasing order. If the median of the data is 18 and its mean deviation about mean is 7.6, then the mean of this data is:
17
16
16.5
15.5
The correct Answer is:C
To solve the problem step by step, we need to find the mean of the data set 4,10,x,y,27 given that the median is 18 and the mean deviation about the mean is 7.6.
Step 1: Determine the value of x
Since the data is in increasing order and consists of 5 terms, the median will be the third term. Given that the median is 18, we can conclude that:
x=18
Thus, the data set becomes:
4,10,18,y,27
Step 2: Calculate the mean of the data
The mean M of the data set is calculated as follows:
M=4+10+18+y+275
Calculating the sum:
4+10+18+27=59
So,
M=59+y5
Step 3: Set up the equation for mean deviation
The mean deviation (MD) about the mean is given by the formula:
MD=1nn∑i=1|xi−M|
For our data set, this becomes:
MD=15(|4−M|+|10−M|+|18−M|+|y−M|+|27−M|)
We know that MD=7.6, so:
15(|4−M|+|10−M|+|18−M|+|y−M|+|27−M|)=7.6
Multiplying both sides by 5 gives:
|4−M|+|10−M|+|18−M|+|y−M|+|27−M|=38
Step 4: Substitute M into the mean deviation equation
Substituting M=59+y5 into the mean deviation equation:
|4−59+y5|+|10−59+y5|+|18−59+y5|+|y−59+y5|+|27−59+y5|=38
Step 5: Simplify each absolute value term
Let M=59+y5. We will analyze each term:
1. |4−M|=|4−59+y5|=∣∣∣20−59−y5∣∣∣=|20−59−y|5=|y+39|5
2. |10−M|=|10−59+y5|=∣∣∣50−59−y5∣∣∣=|y+9|5
3. |18−M|=|18−59+y5|=∣∣∣90−59−y5∣∣∣=|y+31|5
4. |y−M|=|y−59+y5|=∣∣∣5y−59−y5∣∣∣=|4y−59|5
5. |27−M|=|27−59+y5|=∣∣∣135−59−y5∣∣∣=|y+76|5
Step 6: Combine the terms
Combining all the terms, we have:
|y+39|+|y+9|+|y+31|+|4y−59|+|y+76|5=38
Multiplying through by 5:
|y+39|+|y+9|+|y+31|+|4y−59|+|y+76|=190
Step 7: Solve for y
This equation can be solved by considering different cases for y based on the values of the absolute terms. After solving, we find:
y=23.5
Step 8: Calculate the mean
Now substituting y back into the mean formula:
M=59+23.55=82.55=16.5
Final Answer
Thus, the mean of the data is:
16.5
Since the data is in increasing order and consists of 5 terms, the median will be the third term. Given that the median is 18, we can conclude that:
x=18
Thus, the data set becomes:
4,10,18,y,27
The mean M of the data set is calculated as follows:
M=4+10+18+y+275
Calculating the sum:
4+10+18+27=59
So,
M=59+y5
The mean deviation (MD) about the mean is given by the formula:
MD=1nn∑i=1|xi−M|
For our data set, this becomes:
MD=15(|4−M|+|10−M|+|18−M|+|y−M|+|27−M|)
We know that MD=7.6, so:
15(|4−M|+|10−M|+|18−M|+|y−M|+|27−M|)=7.6
Multiplying both sides by 5 gives:
|4−M|+|10−M|+|18−M|+|y−M|+|27−M|=38
Substituting M=59+y5 into the mean deviation equation:
|4−59+y5|+|10−59+y5|+|18−59+y5|+|y−59+y5|+|27−59+y5|=38
Let M=59+y5. We will analyze each term:
1. |4−M|=|4−59+y5|=∣∣∣20−59−y5∣∣∣=|20−59−y|5=|y+39|5
2. |10−M|=|10−59+y5|=∣∣∣50−59−y5∣∣∣=|y+9|5
3. |18−M|=|18−59+y5|=∣∣∣90−59−y5∣∣∣=|y+31|5
4. |y−M|=|y−59+y5|=∣∣∣5y−59−y5∣∣∣=|4y−59|5
5. |27−M|=|27−59+y5|=∣∣∣135−59−y5∣∣∣=|y+76|5
Combining all the terms, we have:
|y+39|+|y+9|+|y+31|+|4y−59|+|y+76|5=38
Multiplying through by 5:
|y+39|+|y+9|+|y+31|+|4y−59|+|y+76|=190
This equation can be solved by considering different cases for y based on the values of the absolute terms. After solving, we find:
y=23.5
Now substituting y back into the mean formula:
M=59+23.55=82.55=16.5
Thus, the mean of the data is:
16.5
Topper's Solved these Questions
- JEE (MAIN) 2020 QUESTIONS ( 9TH JAN-AFTERNOON) BOOK - MCGROW HILL PUBLICATIONCHAPTER - JEE (MAIN) 2020 QUESTIONS ( 9TH JAN-AFTERNOON) EXERCISE - JEE (Main) 2020 Questions with Solutions Mathematics (9th Jan - Afternoon)
25 Videos
- JEE (MAIN) QUESTIONS WITH SOLUTIONS MATHEMATICS (7 TH JAN-MORNING )BOOK - MCGROW HILL PUBLICATIONCHAPTER - JEE (MAIN) QUESTIONS WITH SOLUTIONS MATHEMATICS (7 TH JAN-MORNING )EXERCISE - QUESTIONS
25 Videos
Similar Questions
Knowledge Check
If the mode of the data is 18 and the mean is 24, then median is
A18B24C22D21If the mean of a data is 27 and its median is 33 then the mode is
A30B43C45D47
Find the mean deviation about the mean for the data in Question:

View SolutionFind the mean deviation about the mean for the data in Question:

View SolutionFind the mean deviation about the mean for the following data :
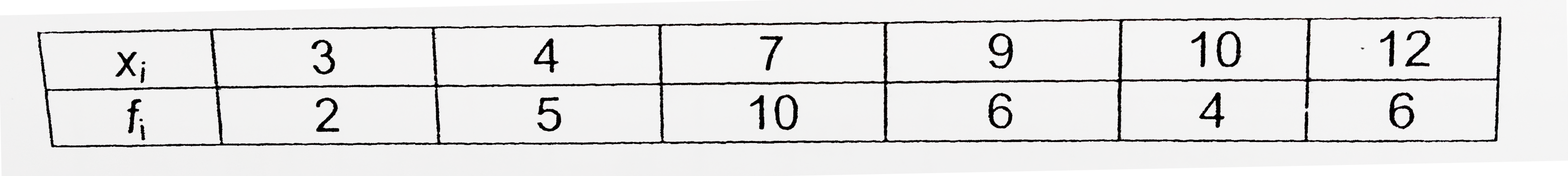
View SolutionFind the mean deviation about the mean for the following data :
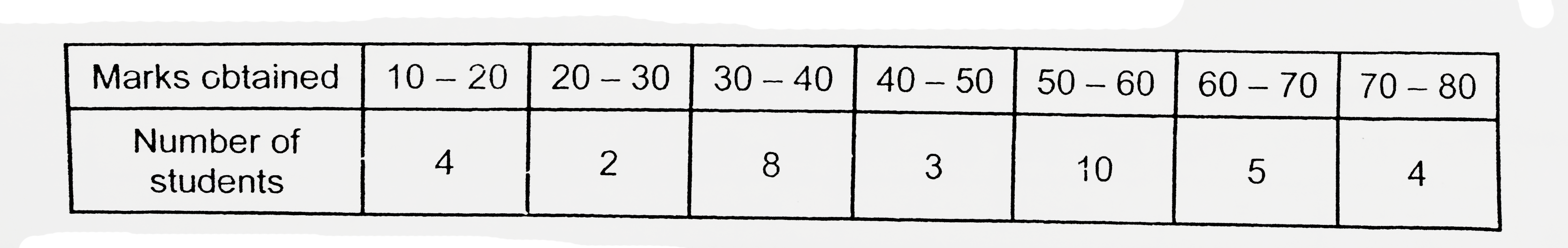
View SolutionFind the mean deviation about the median for the following data:
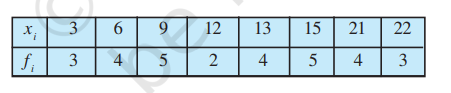
View SolutionFind the mean deviation about the median for the data in Question

View Solution