The point of inflection of $y = x^{3} - 5 x^{2} + 3 x - 5$ is

A

$\frac{1}{2}$

B

$\frac{3}{4}$

C

$\frac{7}{4}$

D

$\frac{5}{3}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:D

To find the point of inflection of the function $y = x^{3} - 5 x^{2} + 3 x - 5$ , we need to follow these steps:
Step 1: Find the first derivative $y^{'}$
The first derivative of $y$ is calculated using the power rule of differentiation.
$y^{'} = \frac{d}{d x} (x^{3}) - \frac{d}{d x} (5 x^{2}) + \frac{d}{d x} (3 x) - \frac{d}{d x} (5)$
Calculating each term:
- The derivative of $x^{3}$ is $3 x^{2}$ .
- The derivative of $5 x^{2}$ is $10 x$ .
- The derivative of $3 x$ is $3$ .
- The derivative of a constant $- 5$ is $0$ .
Putting it all together:
$y^{'} = 3 x^{2} - 10 x + 3$
Step 2: Find the second derivative $y^{''}$
Now, we differentiate $y^{'}$ to find the second derivative.
$y^{''} = \frac{d}{d x} (3 x^{2}) - \frac{d}{d x} (10 x) + \frac{d}{d x} (3)$
Calculating each term:
- The derivative of $3 x^{2}$ is $6 x$ .
- The derivative of $10 x$ is $10$ .
- The derivative of a constant $3$ is $0$ .
Putting it all together:
$y^{''} = 6 x - 10$
Step 3: Set the second derivative equal to zero
To find the points of inflection, we set the second derivative equal to zero:
$6 x - 10 = 0$
Step 4: Solve for $x$
Now, we solve for $x$ :
$6 x = 10 x = \frac{10}{6} = \frac{5}{3}$
Step 5: Find the corresponding $y$ value
Now we substitute $x = \frac{5}{3}$ back into the original function $y$ to find the corresponding $y$ value.
$y = {(\frac{5}{3})}^{3} - 5 {(\frac{5}{3})}^{2} + 3 (\frac{5}{3}) - 5$
Calculating each term:
- ${(\frac{5}{3})}^{3} = \frac{125}{27}$
- $5 {(\frac{5}{3})}^{2} = 5 \cdot \frac{25}{9} = \frac{125}{9}$
- $3 (\frac{5}{3}) = 5$
- $- 5 = - 5$
Now substituting these values into the equation:
$y = \frac{125}{27} - \frac{125}{9} + 5 - 5$
To combine these fractions, we need a common denominator, which is 27:
$y = \frac{125}{27} - \frac{375}{27} + \frac{135}{27} - \frac{135}{27} y = \frac{125 - 375 + 135 - 135}{27} = \frac{- 250}{27}$
Final Result
The point of inflection is:
$(\frac{5}{3}, - \frac{250}{27})$

Updated on:7/8/2024

|

Class 12 MATHS APPLICATION OF DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

The slope of the tangent at the point of inflection of $y = x^{3} - 3 x^{2} + 6 x + 2009$ is equal to :
A2
B3
C1
D4
Concavity and convexity :
if $f'' (x) > 0 \forall x \in (a, b)$ then the curve y=f(x) is concave up ( or convex down) in (a,b) and $if f'' (x) < 0 \forall x \in (a, b)$ then the curve y=f(x) is concave down (or convex up ) in (a,b)

Inflection point :
The point where concavity of the curve changes is known as point of inflection (at inflection point $f'' (x)$ is equal to 0 or undefined)

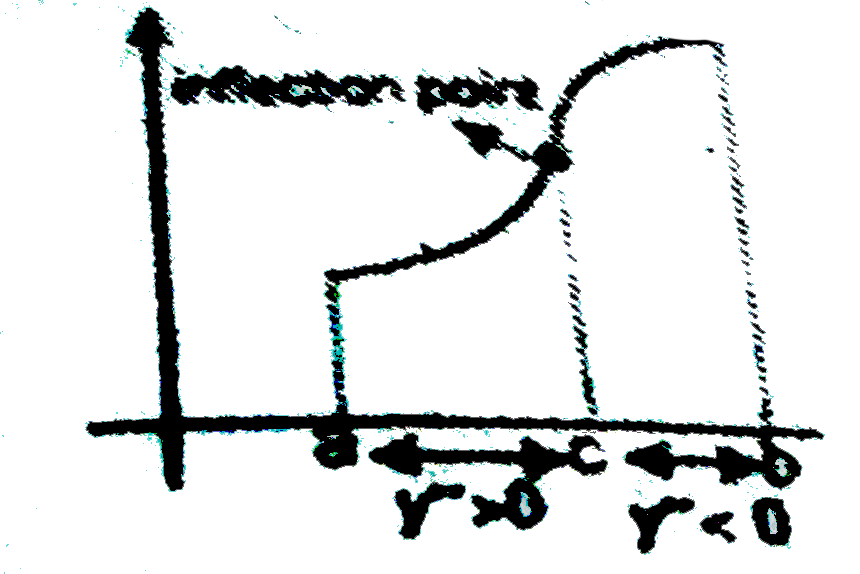
Number of point of inflection for $f (x) = {(x - 1)}^{3} {(x - 2)}^{2}$ is :
A $1$
B $2$
C $3$
D $4$

MCGROW HILL PUBLICATION-APPLICATIONS OF DERIVATIVES-Solved Examples ( CONCEPT - BASED SINGLE CORRECT ANSWER TYPE QUESTIONS )

Home
Ask Doubt
Profile