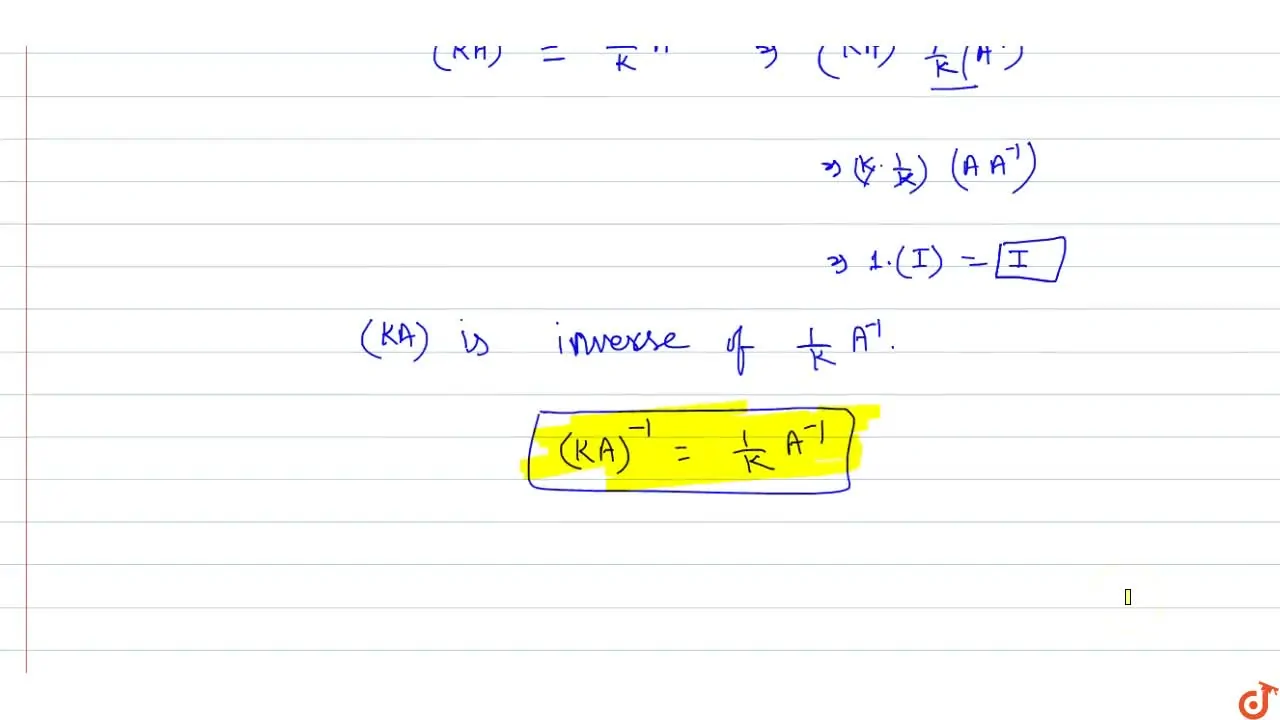
If A is 3×3 invertible matrix, then show that for any scalar k (non-zero),kA is invertible and (kA)−1=1kA−1I
k.A−1
1k.A−1
−k.A−1
none of these
The correct Answer is:B
(kA)−1=1k.A−1 is true
Related Playlists
- BOOK - RS AGGARWALCHAPTER - SYSTEM OF LINEAR EQUATIONSEXERCISE - EXERCISE 8A (VALUE BASED QUESTIONS)4videos
SYSTEM OF LINEAR EQUATIONS
- BOOK - RS AGGARWALCHAPTER - STRAIGHT LINE IN SPACEEXERCISE - Objective Questions19videos
STRAIGHT LINE IN SPACE
- BOOK - RS AGGARWALCHAPTER - THE PLANEEXERCISE - Objective Questions35videos
THE PLANE
Similar Questions
If A is 3×3 invertible matrix, then show that for any scalar k (non-zero),kA is invertible and (kA)−1=1kA−1I
View SolutionIf A is an invertible square matrix then ∣∣A−1∣∣=?
View SolutionIf A is an invertible matrix, then prove that (A1)−1=(A−1)1
View SolutionIf A is a 3×3 invertible matrix, then what will be the value of k if det(A–1)=(detA)k .
View SolutionIf A is an invertible matrix and A−1=[3556] then A=?
View SolutionIf A is an invertible square matrix; then AT is also invertible and (AT)−1=(A−1)T
View SolutionIf A is invertible matrix of order 3×3, then ∣∣A−1∣∣ is equal to…………
View SolutionIf A is an invertible matrix of order n and k is any positive real number, then the value of [det(kA)]1detA is
View SolutionIf A is an invertible matrix of order n and k is any positive real number. Then the value of [∂(kA)]−1 det A is :
View SolutionIf A is a skew-symmetric, then kA is a...........(where, k is any scalar) .
View Solution