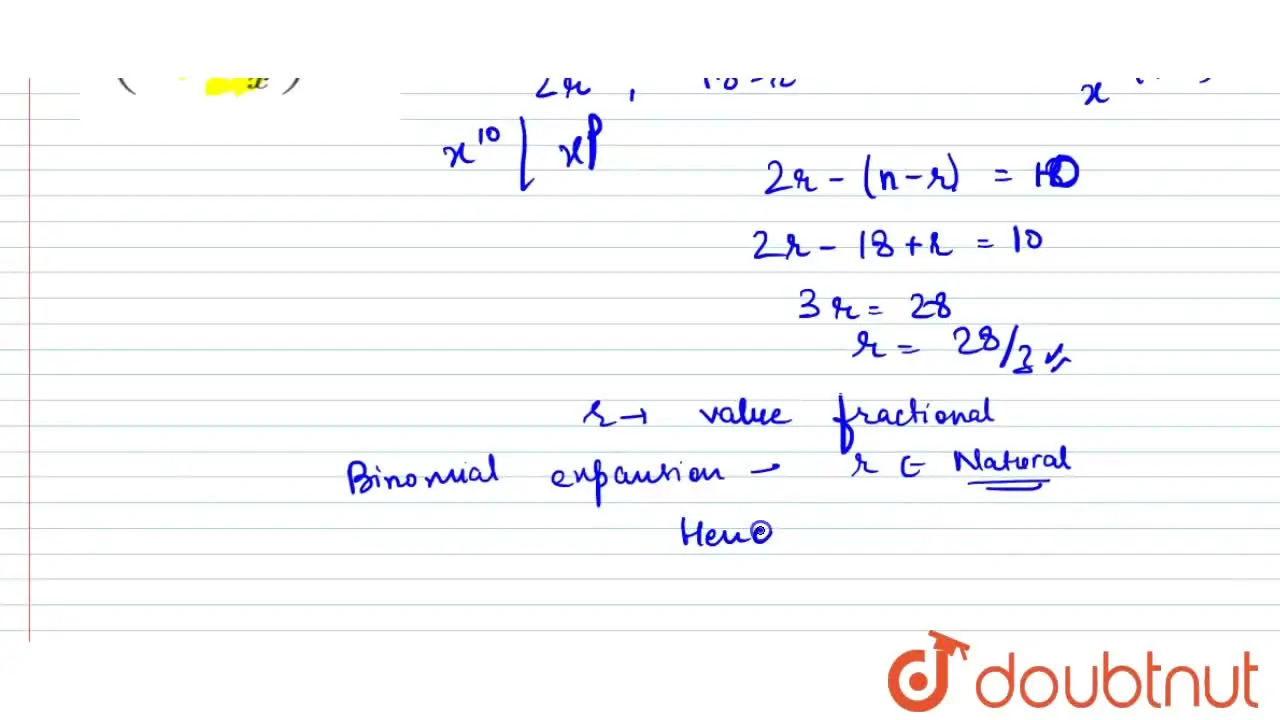
Prove that there is no term containing $x^{10}$ in the expansion of ${(x^{2} - \frac{2}{x})}^{18} .$

Video Solution

Text Solution

Verified by Experts

The general term in the given expansion is given by
$T_{r + 1} = {(- 1)}^{r} \times .^{18} C_{r} \times {(x^{2})}^{(18 - r)} \times {(\frac{2}{x})}^{r}$
$\Rightarrow T_{(r + 1)} = {(- 1)}^{r} \times .^{18} C_{r} \times 2^{r} \times x^{(36 - 3 r)}$ .
Let $T_{r + 1} contain x^{10}$ . Then,
$36 - 3 r = 10 \Rightarrow 3 r = 26 \Rightarrow r = \frac{26}{3}$
Since the value of r cannot be a fraction , so there is no term containing $x^{10}$ .

Updated on:21/07/2023

|

Class 11 MATHS BINOMIAL THEOREM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Which term contains $x^{3}$ in the expansion of ${(3 x - \frac{1}{2 x})}^{8}$ ?
A $2^{n d}$
B $3^{r d}$
C $5^{t h}$
DNone of these
The coefficient of terms containing $x^{5}$ in the
expansion of ${(2 - x + 3 x^{2})}^{2}$ , is
A3
B4
C5
Dnone of these
The term independent of x in the expansion of ${(x - \frac{3}{x^{2}})}^{18}$ is
A $.^{18} c_{6} 0$
B $.^{18} c_{6} 3^{6}$
C $.^{18} c_{12}$
D $.^{18} c_{6} 3^{12}$

RS AGGARWAL-BINOMIAL THEOREM-EXERCISE 10B

Home
Ask Doubt
Profile