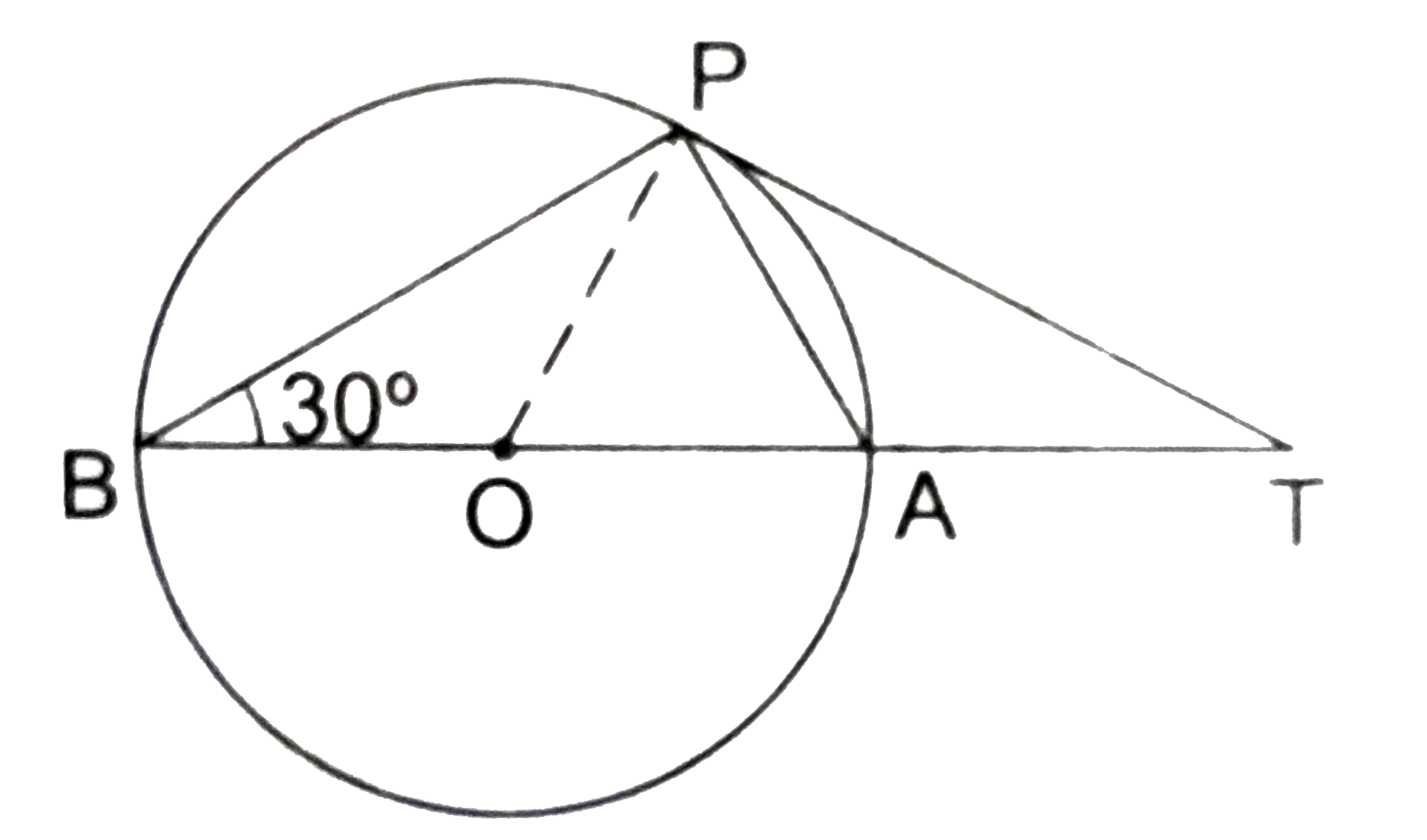
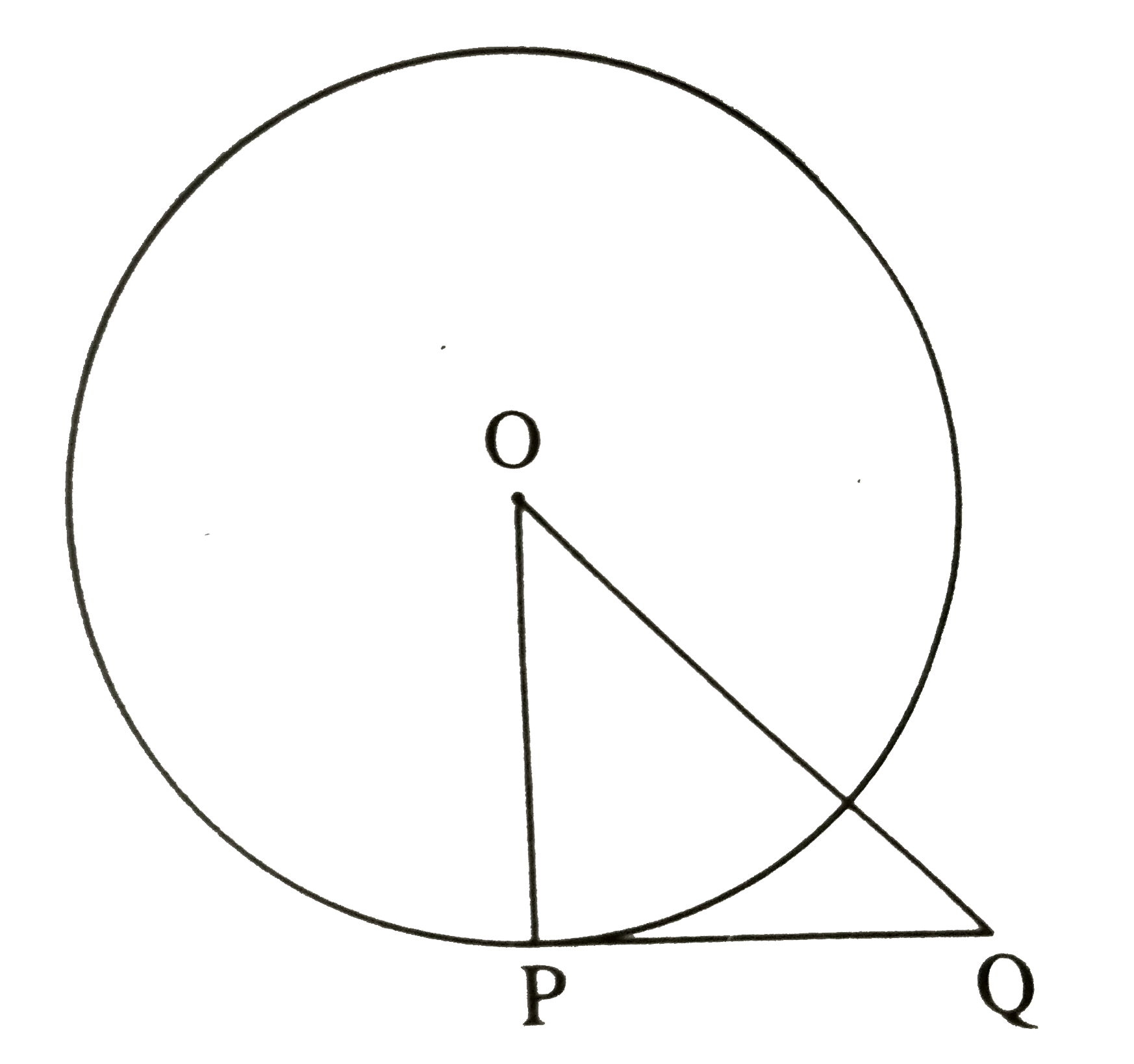
In the given figure, O is the centre of a circle, BOA is its diameter and the tangent at the point P meets BA extended at T. If ∠PBO=30∘ then ∠PTA=?
A
60∘
B
30∘
C
15∘
D
45∘
Video Solution
Text Solution
The correct Answer is:B
∠APB=90∘ [angle in a semicircle]
∴∠PAB=90∘−∠PBA=90∘−30∘=60∘
∠PAT+∠PAB=180∘[ linear pair ]
⇒∠PAT=180∘−∠PAB=180∘−60∘=120∘
∠APT=PBA=30∘ [angles an alternate segments].
Now, ∠APT+∠PAT+∠PTA=180∘ [in ΔPAT]
⇒∠PTA=180∘−(30∘+120∘)=30∘.
|
Topper's Solved these Questions
- CIRCLESBOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Assertion-and-Reason
1 Video
- CIRCLESBOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Test Yourself
20 Videos
- CIRCLESBOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Multiple Choice Questions (Mcq)
15 Videos
- ARITHMETIC PROGRESSIONBOOK - RS AGGARWALCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Multiple Choice Questions (Mcq)
30 Videos
- CONSTRUCTIONSBOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - Test Yourself
10 Videos
Similar Questions
Knowledge Check
In the given figure, O is the centre of a circle, BOA is its diameter and the tangent at the point P meets BA extended at T. If ∠PBO=30∘,then ∠PTA is equal to
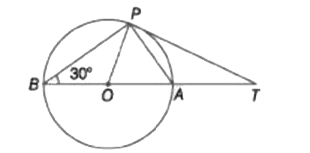 A60∘B30∘C15∘D45∘
A60∘B30∘C15∘D45∘In figure , BOA is a diameter of circle and the tangent at point P meets BA produced at. T if ∠PBO=30∘ then ∠PTA=
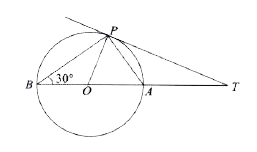 A30∘B60∘C90∘D45∘
A30∘B60∘C90∘D45∘In the figure, O is the centre of a circle and AT is a tangent at point A. The measure of ∠BAT is:
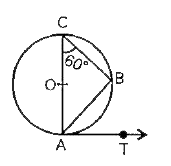 A30∘B60∘C75∘D105∘
A30∘B60∘C75∘D105∘
In the given figure, O is the centre of the circle and TP is the tangent to the circle from an external point T. If ∠PBT=30∘, prove that BA:AT =2:1
View SolutionIn the given figure, O is the centre of the circle.

Name a chord, which is not the diameter of the circle.
View SolutionIn the figure , point P is the centre of the circle and AB is the diameter . The co-ordinates of A are
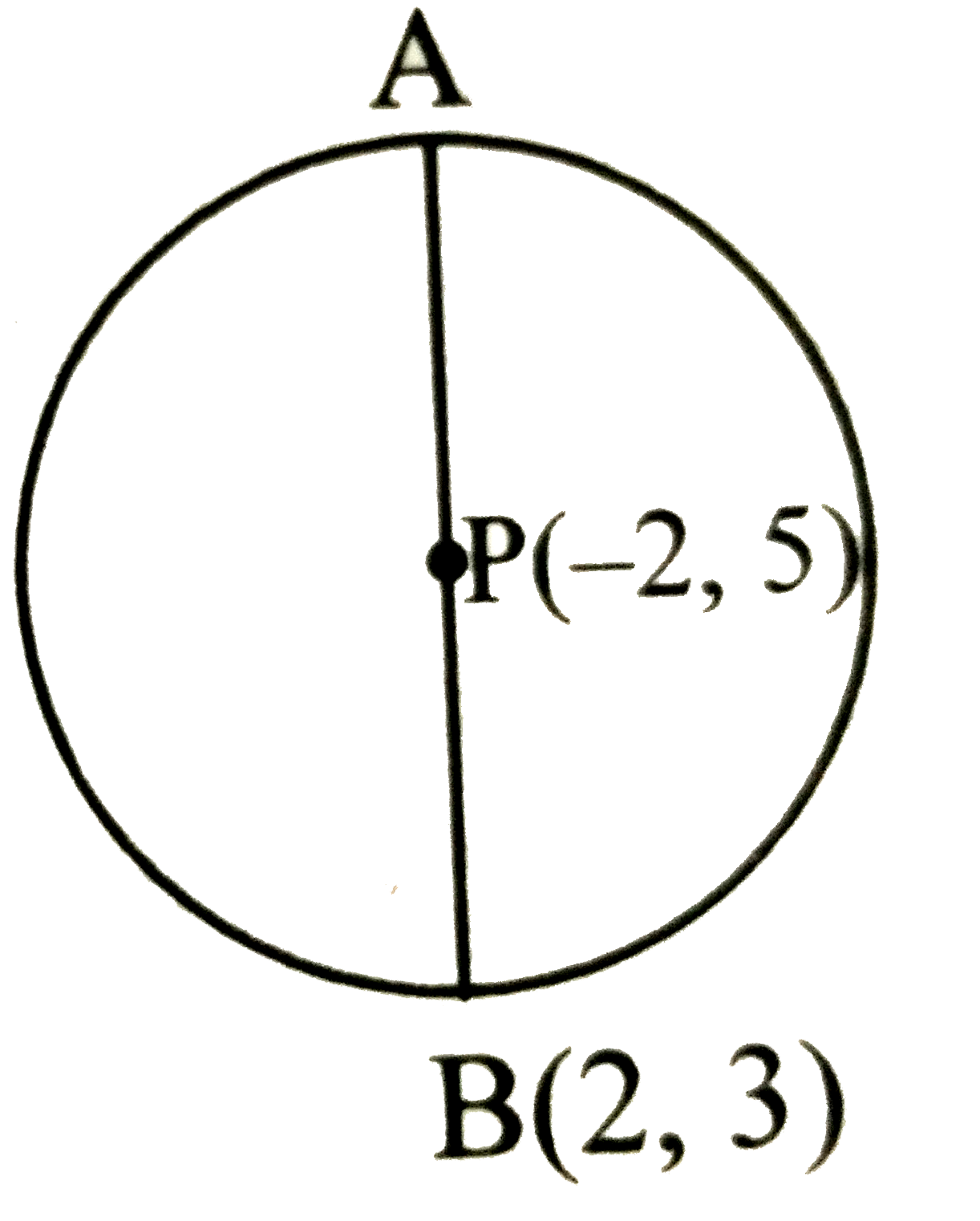
View SolutionIn the giben figure, AB is the diameter of the circle, with centre O and AT is the tangent. Calculate the calue of x.
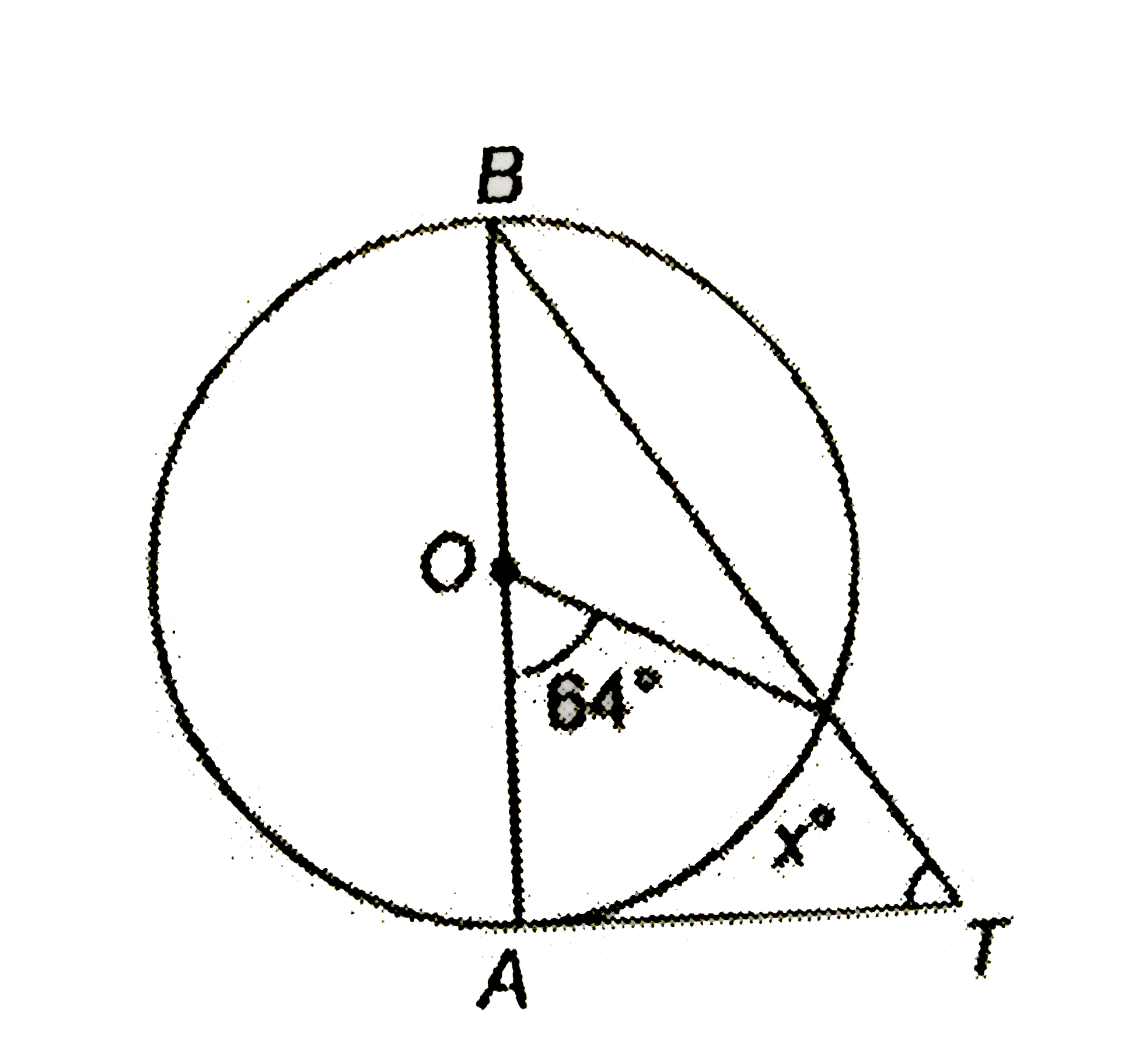
View SolutionIn the figure,O is the centre of the circle PQ is the tangent at point P then what is the measure of ∠OPQ ?Why ?
View Solution