In the given figure, the incircle of $Δ A B C$ touches the sides $B C$ , $C A$ and $A B$ at $P$ , $Q$ and $R$ respectively. Prove that
$(A R + B P + C Q) = (A Q + B R + C P)$
$= \frac{1}{2} (perimeter of Δ A B C)$ .

Video Solution

Text Solution

Verified by Experts

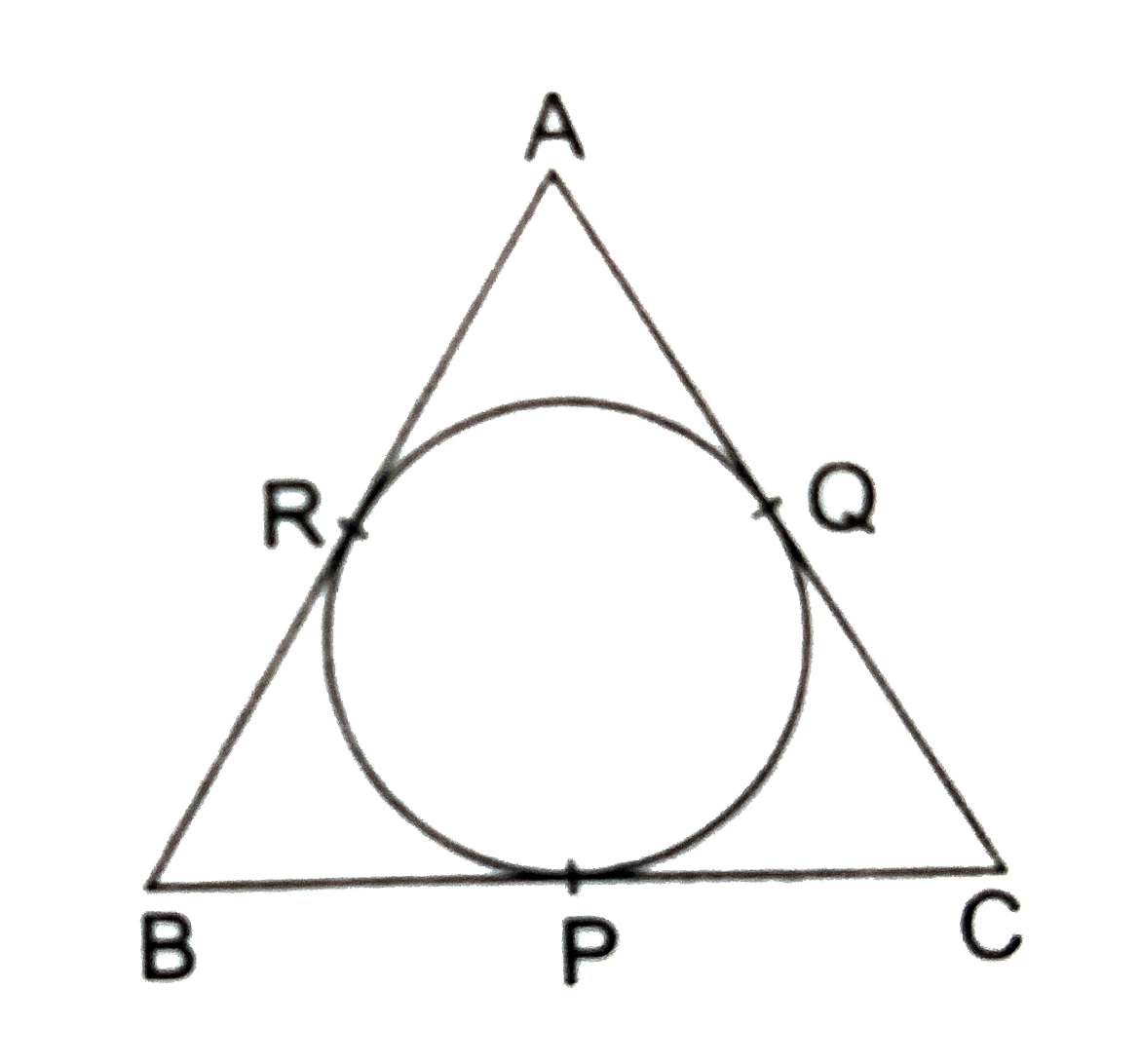
We know that the lengths of tangents from an exterior point to a circle are equal.
$∴ A R = A Q$ , ………. $(i)$ [tangents from $A$ ]
$B P = B R$ , ……… $(i i)$ [tangents from $B$ ]
$C Q = C P$ …………. $(i i i)$ [tangents from $C$ ]
$∴ (A R + B P + C Q) = (A Q + B R + C P) = k$ (say).
Perimeter of $Δ A B C = (A B + B C + C A)$
$= (A R + B R) + (B P + C P) + (C Q + A Q)$
$= (A R + B P + C Q) + (A Q + B R + C P)$
$= (k + k) = 2 k$
$\Rightarrow k = \frac{1}{2} (perimeter of Δ A B C)$ .
$∴ (A R + B P + C Q) = (A Q + B R + C P)$
$= \frac{1}{2} (perimeter of Δ A B C)$ .

|

Updated on:21/07/2023

Class 10 MATHS CIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Incircle of $Δ A B C$ touches the sides BC, AC and AB at D, E and F, respectively. Then answer the following question
$∠ D E F$ is equal to
A $\frac{π - B}{2}$
B $π - 2 B$
C $A - C$
Dnone of these
Question 2 - Select One
Incircle of $Δ A B C$ touches the sides BC, AC and AB at D, E and F, respectively. Then answer the following question
Area of $Δ D E F$ is
A $2 r^{2} sin (2 A) sin (2 B) sin (2 C)$
B $2 r^{2} cos . \frac{A}{2} cos . \frac{B}{2} cos . \frac{C}{2}$
C $2 r^{2} sin (A - B) sin (B - C) sin (C - A)$
Dnone of these

RS AGGARWAL-CIRCLES-Test Yourself

Home
Ask Doubt
Profile