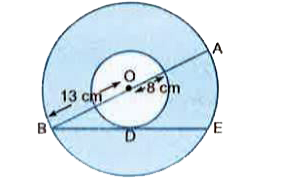
The radii of two concentric circle are 13 cm and 8 cm. AB is a diameter of the bigger circle and BD is a tangent to the smaller circle touching it at D and the bigger circle at E. Point A is joined to D. Find the length of AD.
We have ∠AEB=90∘ [angle in a semicircle]
Also, OD⊥BEand OD bisects BE.
In right ΔOBD, we have
OB2=OD2+BD2 [by Pythagoras' theorem]
⇒BD=√OB2−OD2=√132−82cm [∵OB=13cm, OD=8cm]
∴BE=2BD=2√105cm [∵D is the midpoint of BE]
In right ΔAEB, we have
AB2=AE2+BE2 [by Pythagoras' theorem]
⇒AE=√AB2−BE2=√262−(2√1052)cm=√256cm=16cm
[∵AB= diameter =2×OB=2×13cm=26cm]
In right ΔAED, we have
AD2=AE2+DE2 [by Pythagoras' theorem]
⇒AD=√AE2+DE2=√162+(√105)2cm
=19cm [∵DE=BD=√105cm].
[Note We can also find AE by using midpoint theorem, since in ΔABE, O is the midpoint of AB and D is the midpoint of BE and so OD∣∣AE and AE=2×OD=16cm]
Topper's Solved these Questions
- CIRCLESBOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Exercise 8A
16 Videos
- CIRCLESBOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Exercise 8B
15 Videos
- ARITHMETIC PROGRESSIONBOOK - RS AGGARWALCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Multiple Choice Questions (Mcq)
30 Videos
- CONSTRUCTIONSBOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - Test Yourself
10 Videos
Similar Questions
The radii of two concentric circles are 13cm and 8cm, AB is a diameter of bigger circle. BD is a tangent to the smaller circle touching it at D. Find the length AD.
View SolutionIn Fig., the radii of two concentric circles are 13 cm and 8 cm. AB is diameter of the bigger circle. BD is the tangent to the smaller circle touching it at D. Find the length of AD.
View Solution
Knowledge Check
The radii of two concentric circle are 13 cm and 8 cm. AB is a diameter of the bigger circle and BD is a tangent to the smaller circle touching it at D and the bigger circle at E. Point A is joined to D. Find the length of AD.
A20 cmB19 cmC18 cmD17 cmThe radius of two concentric circles are 9 cm and 15 cm. If the chord of the greater circle be a tangent to the smaller circle, then the length of that chord is
A24 cmB12 cmC30 cmD18 cmIf radii of two concentric circles are 4 cm and 5 cm Then the length of the chord of one circle which is tangent to the other circle is
A3 cmB6 cmC9 cmD1 cm
The radii of two co-centric circles are 13 cm and 8 cm respectively. AB is diameter of outer circle and BE is tangent to the inner circles which touches the inner circle at point D. Then find the length of AD.
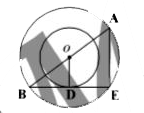
View SolutionThe radii of two concentric circle are 13 cm and 8 cm. AB is a diameter of the bigger circle and BD is a tangent to the smaller circle touching it at D and the bigger circle at E. Point A is joined to D. Find the length of AD.
View SolutionTwo concentric circle of radii 13cm and 12 cm. What is the length of the chord of the larger circle which is tangent to the smaller circle?
View SolutionIf radii of two concentric circles are 12 cm and 13 cm, find the length of each chord of one circle which is tangent to the other circle.
View SolutionIf radii of two concentric circles are 4 cm and 5 cm, then length of each chord of one circle which is tangent to the other circle, is
View Solution