Question

Draw the graph of the equation $2x+3y=6$. From the graph, find the value of y, when
(i) $x=\frac{3}{2}$ (ii) $x=-3$.

Explanation

We have
`2x+3y=6 rArr 3y = 6-2x rArry=(6-2x)/3." "` …(i)
Putting`x=0 " in (i) , we get " y= (6-(2 xx 0))/3 = (6-0)/3 = 6/3 =2.`
Putting `y=0 " in (i) , we get "(6-2x) = 3 xx 0 = 0 rArr 2x = 6 rArr x = 3.`
Putting `x=6" in (i), we get "y=((6-2 xx6))/3= ((6-12))/3=(-6)/3=-2.`
Thus, we have the following table:
`{:(x,0,3," "6),(y,2,0,-2):}`
On the graph paper, draw lines X'OX and YOY' as the x-axis and y-axis respectively.
Plot the points `A(0,2), B(3,0) and C(6,-2)` on this graph paper.
Join AB and BC to get the line ABC.Extend it in both the directions to get the required graph.

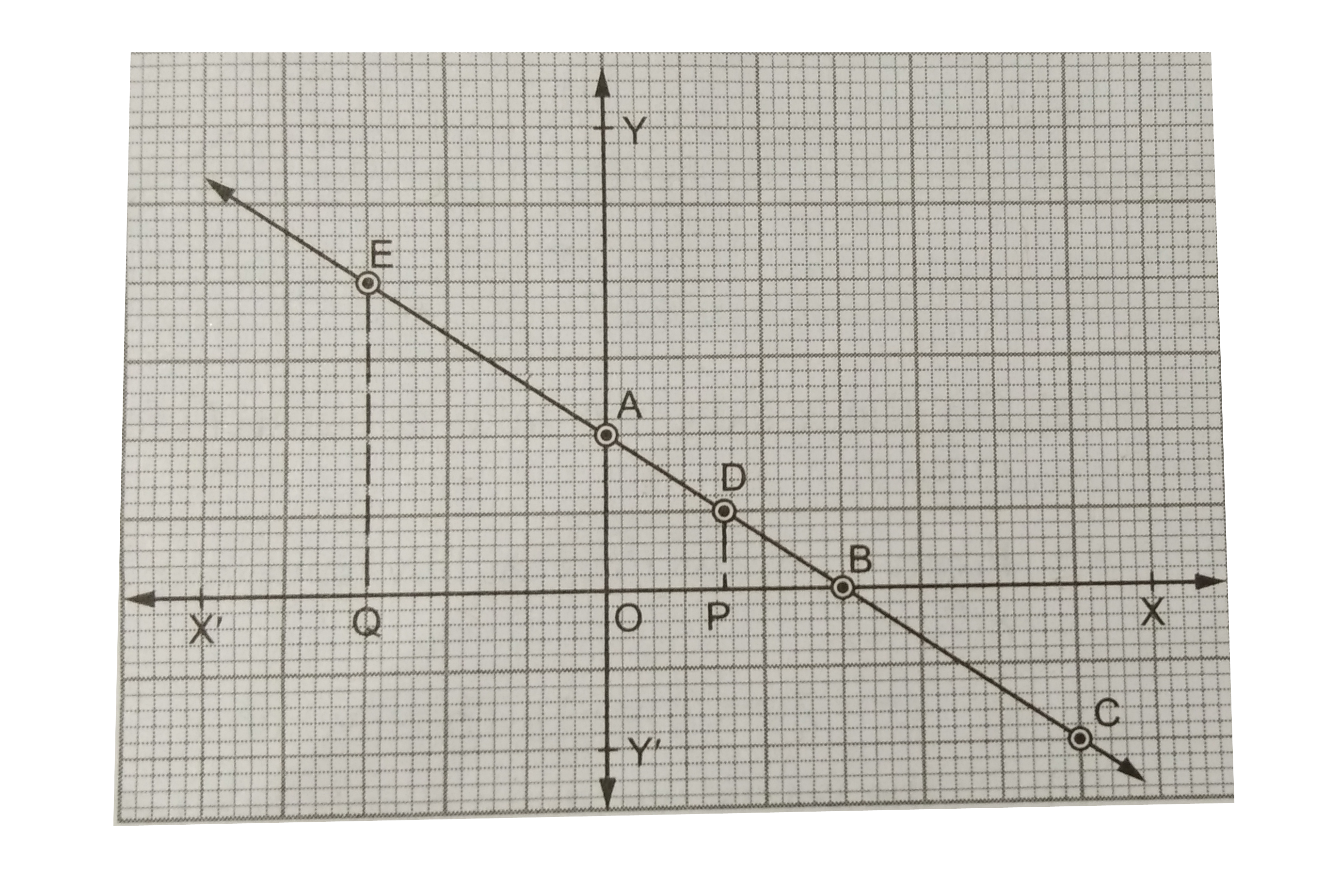
(a) On the x-axis, take a point P such that `OP=x=3/2.`
Draw `PD bot X'OX`, meeting the graph line at `D(3/2,1).`
Thus, `(x=3/2 rArr y=1)`. Thus, when `x=3/2`, then y=1.
(b) On the x-axis , take a point Q such that `OQ=x=-3.`
Draw `QE bot X'OX` , meeting the graph line at `E(-3,4).`
Thus,`(x=-3 rArr y=4)`, i.e., when x=-3, then y=4.