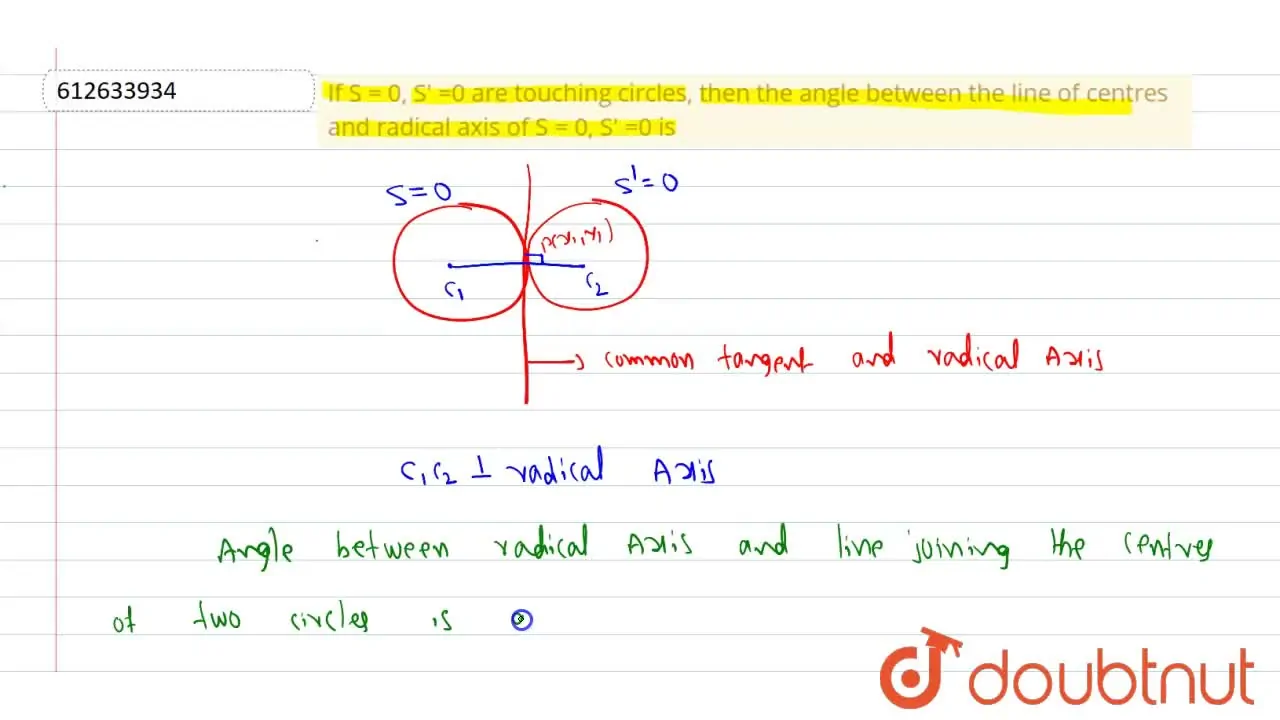
If S = 0, S' =0 are touching circles, then the angle between the line of centres and radical axis of S = 0, S' =0 isSolution in Telugu
90∘
30∘
0∘
60∘
The correct Answer is:A
Step by step video, text & image solution for If S = 0, S' =0 are touching circles, then the angle between the line of centres and radical axis of S = 0, S' =0 is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - AAKASH SERIESCHAPTER - SYSTEM OF CIRCLESEXERCISE - EXERCISE -2.123videos
SYSTEM OF CIRCLES
- BOOK - AAKASH SERIESCHAPTER - SYSTEM OF CIRCLESEXERCISE - EXERCISE 2.222videos
SYSTEM OF CIRCLES
- BOOK - AAKASH SERIESCHAPTER - SYSTEM OF CIRCLESEXERCISE - Exercise-II (Class work)45videos
SYSTEM OF CIRCLES
- BOOK - AAKASH SERIESCHAPTER - STRAIGHT LINES (3D)EXERCISE - PRACTICE EXERCISE28videos
STRAIGHT LINES (3D)
- BOOK - AAKASH SERIESCHAPTER - THEORY OF EQUATIONSEXERCISE - EXERCISE-III46videos
THEORY OF EQUATIONS
Similar Questions
Find the acute angle or angle or intersection of the following circles.
a. x2+y2−12x−6y+41=0,x2+y2+4x+6y−59=0View SolutionFind the equation of the radical axis of the following circles
x2+y2−3x−4y+5=0,3(x2+y2)−7x+8y−11=007:31View SolutionIf the angle between the two equal circles with centres (-2,0),(2,3) is 120∘ then the radius of the circle is
03:22View SolutionThe value of k for which the lines
x+1−3=y+52k=z−42 and x−33k=y−21=z+17 are perpendicular isView SolutionThe quotient obtained when 3x4−x3+2x2−2x−4 is divided by x2−4 is
01:49View SolutionIf the equation fo two circles whose radii are a, a ' are S = 0 and S′=0, then show that circles Sa+S′a′=0andSa−S′a'=0 intersect orthogonally.
02:46View SolutionA circle S=0 with radius √2 touches the line x+y-2=0 at (1,1). Then the length of the tangent drawn from the point (1,2) to S=0 is
04:49View Solutionउन वृतों के समीकरण ज्ञात कीजिए जो x- अक्ष , y- अक्ष और सरल रेखा x=a,(a>0) को स्पर्श करते है ।
02:47View SolutionThe lines represented by 5x2−xy−5x+y=0 are normals to a circle S=0. If this circle touches the circle
S′≡x2+y2−2x+2y−7=0 externally, then the equation of the chord of contact of centre of s′=0 with respect to S=0 is06:25View SolutionIf S=0,S'=0 are any two non- concentric circles then its radical axis is
02:13View SolutionIf the circle S=0 touches X -axis then
04:47View SolutionLet L=0 is a variable straight line passing through the point A(1,1) and S=0 is a variable circle with centre O(0,0) ,L=0 touches the circle S=0 at point P . Line segment PQ is diameter of circle S=0.If maximum value of AQ is k√2 then k is
02:53View SolutionIf two circles S=0 and s'=0 are touch each other externally then
06:25View SolutionIf the equations of two circles whose radii are a and a' are S=0 and S'=0 , then show that the circles Sa+S'a'=0 and Sa−S'a'=0 intersect orthogonally.
09:42View SolutionThe radical axis of two circles S=0, S'=0 does not exist if
View Solution