घन पूर्णांक n1 व n2 के लिए व्यंजक
(1+i)n1+(1+i3)n1+(1−i5)n2+(1+i7)n2, जहाँ i=√−1, का मान वास्तविक होगा, यदि और केवल यदि
n1=n2+1
n1=n2−1
n1=n2
n1>0,n2>0
The correct Answer is:D
Step by step video, text & image solution for घन पूर्णांक n_(1) व n_(2) के लिए व्यंजक (1+i)^(n_(1))+(1+i^(3))^(n_(1))+(1-i^(5))^(n_(2))+(1+i^(7))^(n_(2)), जहाँ i=sqrt(-1), का मान वास्तविक होगा, यदि और केवल यदि by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- सम्मिश्र संख्यायेंBOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Jee Advanced (More than one correct Answers)
23 Videos
- सम्मिश्र संख्यायेंBOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Jee Advanced (Reasoning)
6 Videos
- सम्मिश्र संख्यायेंBOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Solved Multiple Choice Questions (डी मोयवर प्रमेय तथा इकाई के मूल )
59 Videos
- समुच्चय सिद्धान्त तथा सम्बन्ध BOOK - ERRORLESS HINDICHAPTER - समुच्चय सिद्धान्त तथा सम्बन्ध EXERCISE - JEE Advanced (Reasoning type questions)
3 Videos
- सरल रेखा BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)
4 Videos
Similar Questions
माना z1 तथा z2 दो सम्मिश्र संख्याएँ इस प्रकार है कि z1≠z2 तथा |z1|=|z2| . यदि z1 धनात्मक वास्तविक भाग तथा z2 ऋणात्मक काल्पनिक भाग है, तब z1+z2z1−z2 होगा
View Solutionकथन-1: यदि n एक विषम पूर्णांक 3 से बडा है लेकिन 3 का गुणज नहीं है, तब (x+1)n−xn−1,x3+x2+x से विभाज्य है।
कथन-2: यदि n एक विषम पूर्णांक 3 से बड़ा है लेकिन 3 का गुणज नहीं है हम लेते हैं 1+ωn+ω2n=3
View Solution(cosθ+isinθ)4(sinθ+icosθ)5=
View Solutionमाना R वास्तविक संख्याओं का समुच्चय है
कथन-1 : A={(x,y)∈R×R:y−x एक पूर्णांक है} R पर एक तुल्यता संबंध है |
कथन-2 : B={(x,y)∈R×R:x=αy किसी परिमेय संख्या α के लिए} R पर एक तुल्यता संबंध है |
View Solution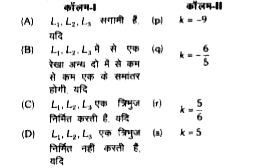
View SolutionIf n_(1),n_(2) are positive integers,then (1+i)^(n_(1))+(1+i^(3))^(n_(1))+(1+i_(5))^(n_(2))+(1+i^(7))^(n_(2)) is real if and only if :
View SolutionLet n1<n2<n3<n4<n5 be positive integers such that n1+n2+n3+n4+n5=20. then the number of distinct arrangements n1,n2,n3,n4,n5 is
View SolutionIf cos36^(@)+cot(7(1)/(2)@)=sqrt(n_(1))+sqrt(n_(2))+sqrt(n_(3))+sqrt(n_(1))+sqrt(n_(5))+sqrt(n_(6)) then the value of sum_(i=1)^(6)n_(i)^(2) must be
View SolutionIf n1+n2+n3+n4=20 such that ni≥i+1∀iε{1,2,3,4} and niεI then number of solutions are
View SolutionIf l2i+m2i+n2i=1, (i=1,2,3) and lilj+mimj+ninj=0,(i≠j,i,j=1,2,3) and Δ=∣∣ ∣∣l1m1n1l2m2n2l3m3n3∣∣ ∣∣ then
View SolutionIf 4cos36∘+cot(71∘2)=√n1+√n2+√n3+√n4+√n5+√n6, then the value of (Σ6i=1n2i10) is equal to
View Solutionघन पूर्णांक n1 व n2 के लिए व्यंजक
(1+i)n1+(1+i3)n1+(1−i5)n2+(1+i7)n2, जहाँ i=√−1, का मान वास्तविक होगा, यदि और केवल यदि
View SolutionIf 4cos36∘+cot(71∘2)=√n1+√n2+√n3+√n4+√n5+√n6 then the product of the digits in 6∑i=1n2i =
View SolutionIf A =⎡⎢⎣l1m1n1l2m2n2l3m3n3⎤⎥⎦ then Find A+I
View SolutionIf l2i+m2i+n2i=1, (i=1,2,3) and lilj+mimj+ninj=0,(i≠j,i,j=1,2,3) and Δ=∣∣ ∣∣l1m1n1l2m2n2l3m3n3∣∣ ∣∣ then
View SolutionFor positive integers n1 and n2 the value of the expression
(1+i)n1+(1+i3)n1+(1+i5)n2+(1+i7)n2 is a real number if and only if
View Solution
