माना की सम्मिश्र संख्याएँ α तथा 1α क्रमशः वृत्त (x−x0)2+(y−y0)2=r2 तथा (x−x0)2+(y+y0)2=4r2 पर स्थित हैं। यदि z0=x0+iy0 समीकरण 2|z0|2=r2+2 को सन्तुष्ट करता है, तब |α| =
1√2
1/2
1/√7
1/3
The correct Answer is:C
Step by step video, text & image solution for माना की सम्मिश्र संख्याएँ alpha तथा 1/alpha क्रमशः वृत्त (x - x_0)^2 + (y - y_0)^2 = r^2 तथा (x - x_0)^2 + (y + y_0)^2 = 4r^2 पर स्थित हैं। यदि z_0 = x_0 + iy_0 समीकरण 2|z_0|^2 = r^2 + 2 को सन्तुष्ट करता है, तब |alpha| = by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Solved Multiple Choice Questions (डी मोयवर प्रमेय तथा इकाई के मूल )59videos
सम्मिश्र संख्यायें
- BOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Exercise117videos
सम्मिश्र संख्यायें
- BOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Solved Multiple Choice Questions (सम्मिश्र संख्याओं का वर्गमूल, निरूपण और लघुगणक)9videos
सम्मिश्र संख्यायें
- BOOK - ERRORLESS HINDICHAPTER - समुच्चय सिद्धान्त तथा सम्बन्ध EXERCISE - JEE Advanced (Reasoning type questions)3videos
समुच्चय सिद्धान्त तथा सम्बन्ध
- BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)4videos
सरल रेखा
Similar Questions
(cosθ+isinθ)4(sinθ+icosθ)5=
05:30View Solutionयदि z≠0 एक सम्मिश्र संख्या हो, तो
07:39View Solutionयदि (−7−24i)1/2=x−iy, तो x2+y2=
03:10View Solutionमाना R वास्तविक संख्याओं का समुच्चय है
कथन-1 : A={(x,y)∈R×R:y−x एक पूर्णांक है} R पर एक तुल्यता संबंध है |
कथन-2 : B={(x,y)∈R×R:x=αy किसी परिमेय संख्या α के लिए} R पर एक तुल्यता संबंध है |12:09View Solution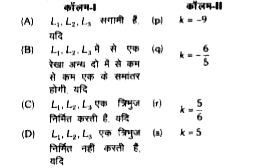
07:40View SolutionLet Z = 18 + 26i where Z0=x0+iy0(x0,y0∈ R) is the cube root of Z having least positive argument. Find the value of x0y0(x0+y0).
01:57View SolutionLet complex numbers alpha and 1/alpha lies on circle (x-x_0)^2+(y-y_0)^2=r^2 and (x-x_0)^2+(y-y_0)^2=4r^2 respectively. If z_0=x_0+iy_0 satisfies the equation 2|z_0|^2=r^2+2 then |alpha| is equal to
11:05View SolutionFind the equation of the normal to the curve x2a2−y2b2=1 at (x0,y0)
05:23View Solutionमाना की सम्मिश्र संख्याएँ α तथा 1α क्रमशः वृत्त (x−x0)2+(y−y0)2=r2 तथा (x−x0)2+(y+y0)2=4r2 पर स्थित हैं। यदि z0=x0+iy0 समीकरण 2|z0|2=r2+2 को सन्तुष्ट करता है, तब |α| =
12:25View Solutionमाना Z= 18 + 26i, जहाँ Z0=x0+iy0(x0,y0∈R) का घनमूल है जिसका कोणांक न्यूनतम धनात्मक है तो (x0,y0∈R) का मान ज्ञात कीजिए।
08:19View SolutionFill in the blanks in the distance of the point P(x0,y0,z0)from z axis is :
[√x20+y20,√y20+z20,√x20+z20,
√(x−x0)2+(y−y0)2]09:57View SolutionLet complex numbers alpha and 1/alpha^- lie on circles (x-x_0)^2+(y-y_0)^2=r^2 and (x-x_0)^2+(y-y_0)^2=4r^2 respectively if z_0=x_0+iy_0 satisfies the equation 2absz_0^2=r^2+2 then absalpha=
12:39View SolutionFind the equations of the tangent and normal to the hyperbola x2a2−y2b2=1. at the point (x0,y0)
08:09View SolutionLet complex numbers alpha and 1/alpha lies on circle (x-x_0)^2(y-y_0)^2=r^2 and (x-x_0)^2+(y-y_0)^2=4r^2 respectively. If z_0=x_0+iy_0 satisfies the equation 2|z_0|^2=r^2+2 then |alpha| is equal to (a) 1/sqrt2 (b) 1/2 (c) 1/sqrt7 (d) 1/3
08:09View SolutionLet complex numbers alpha and 1/alpha lies on circle (x-x_0)^2+(y-y_0)^2=r^2 and (x-x_0)^2+(y-y_0)^2=4r^2 respectively. If z_0=x_0+iy_0 satisfies the equation 2|z_0|^2=r^2+2 then |alpha| is equal to (a) 1/sqrt2 (b) 1/2 (c) 1/sqrt7 (d) 1/3
03:24View Solution