D, E and F are the mid-points of sides of ΔABC. P, Q, R are the mid-points of sides DEF. This process of marking the mid-points and forming a new triangle is continued. How are the areas of these triangles related?Solution in Kannada
The correct Answer is:1:4
Step by step video, text & image solution for D, E and F are the mid-points of sides of Delta ABC. P, Q, R are the mid-points of sides DEF. This process of marking the mid-points and forming a new triangle is continued. How are the areas of these triangles related? by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBOOK - OSWAAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - TOPIC-3 AREA OF SIMILAR TRIANGLES (LONG ANSWER TYPE QUESTIONS-(II))
2 Videos
- TRIANGLESBOOK - OSWAAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - TOPIC-4 PYTHAGOREAN TRIPLETS (MULTIPLE CHOICE QUESTIONS)
16 Videos
- TRIANGLESBOOK - OSWAAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - TOPIC-3 AREA OF SIMILAR TRIANGLES (SHORT ANSWER TYPE QUESTIONS)
8 Videos
- SURFACE AREA AND VOLUMESBOOK - OSWAAL PUBLICATIONCHAPTER - SURFACE AREA AND VOLUMESEXERCISE - EXERCISE 15.5
5 Videos
Similar Questions
Prove that “the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides”.
View SolutionIn ΔPQR, ∠PQR=90∘, The correct relation with respect in ΔPQR is :
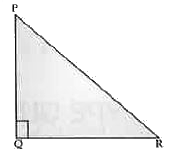
View SolutionIf the area of the similar triangles are equal, then they are congruent. Prove.
View SolutionA copper wire, 3mm in diameter , is wound about a cylinder whose length is 12cm, and diameter 10cm, so as to cover the curved surface of the cylinder . Find the length and mass of the wire, assuming the density of copper to be 8.88gpercm3
View SolutionD, E and F are respectively the mid-points of sides AB, BC and CA of ΔABC . Find the ratio of the areas of ΔDEFandΔABC .
View SolutionIn ΔABC,D,E,F are respectively the mid points of the sides AB, BC and AC. Find ratio of the area of ΔDEF and area of ΔABC.
View SolutionD, E, F are mid points of sides BC, CA, AB of ΔABC. Find the ratio of areas of ΔDEFandΔABC.
View SolutionD, E, F are mid points of sides BC, CA, AB of ΔABC. Find the ratio of areas of ΔDEFandΔABC.
View SolutionIn trigle ABC, the points D, E, F are the midpoints of the sides BC,CA, and AB respee. Tively. Using vector methed ,show that the area of Δ DEF is equal to 14 (area of ABC).
View SolutionD, E, F are mid points of sides BC, CA, AB of ΔABC. Find the ratio of areas of ΔDEFandΔABC.
View SolutionIn the adjoining figure D, E and F are the mid-points of the sides BC, AC and AB respectively. triangle DEF is congruent to triangle :
View SolutionD,E,F are the mid points of the sides BC, CA and AB respectively of a △ABC. Determine the ratio of the areas of △DEF and △ABC.
View SolutionD,E and F are the mid points of the sides BC, CA and AB respectivley of f△ABC then the ratio of the areas of △DEF and ABC =………..
View SolutionIf D, E, f are the mid-point of the sides of triangle ABC, prove that : ar(△DEF)=14ar(△ABC).
View SolutionIf D,E,F are mid-points of the sides of triangle ABC , prove (by vectors) that area of triangle DEF= 1/4 area of triangle ABC .
View Solution
