Prove that opposites of a quadrilaterial circumscribing a circle subtend supplementary angles at the centre of the circle. Solution in Kannada
The correct Answer is:180∘
Step by step video, text & image solution for Prove that opposites of a quadrilaterial circumscribing a circle subtend supplementary angles at the centre of the circle. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLES BOOK - OSWAAL PUBLICATIONCHAPTER - CIRCLES EXERCISE - TEXTBOOK CORNER (EXERCISE 4.1)
6 Videos
- ARITHMETIC PROGRESSIONSBOOK - OSWAAL PUBLICATIONCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - TEXTBOOK CORNER EXERCISE 1.4
5 Videos
- CO-ORDINATE GEOMETRYBOOK - OSWAAL PUBLICATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - TEXTBOOK CORNER (EXERCISE 7.4)
7 Videos
Similar Questions
How many tangents can a circle have ?
View SolutionWhich term of the AP: 121,117,113……. is its first negative term?
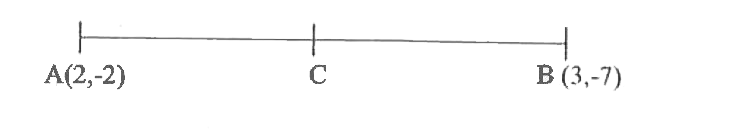
View SolutionDetermine the ratio in which the line 2x + y - 4 = 0 divides the line segment joining the points A(2, -2) and B(3, 7).
View SolutionProve that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the centre.
View SolutionProve that the angle between two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segments joining the points of contact at the centre.
View SolutionProve that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segments joining the points of contact at the centre.
View SolutionProve that for a quadrilateral circumscribed about a circle, the angles subtended by any two opposite sides at the centres are supplementary to each other.
View SolutionProve that opposites of a quadrilaterial circumscribing a circle subtend supplementary angles at the centre of the circle.
View SolutionProve that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the centre.
View SolutionProve that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line - segment joining the points of contact at the centre.
View SolutionProve that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line - segment joining the points of contact at the centre.
View SolutionProve that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line - segment joining the points of contact at the centre.
View SolutionProve that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the centre.
View SolutionProve that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segment joining the points of contact at the centre.
View SolutionProve that the angle between two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segments joining the points of contact at the centre.
View SolutionA circle touches the sides of a quadrilateral ABCD at P, Q, R, S respectively. Show that the angles subtended at the centre by a pair of opposite sides are supplementary.
View Solution
