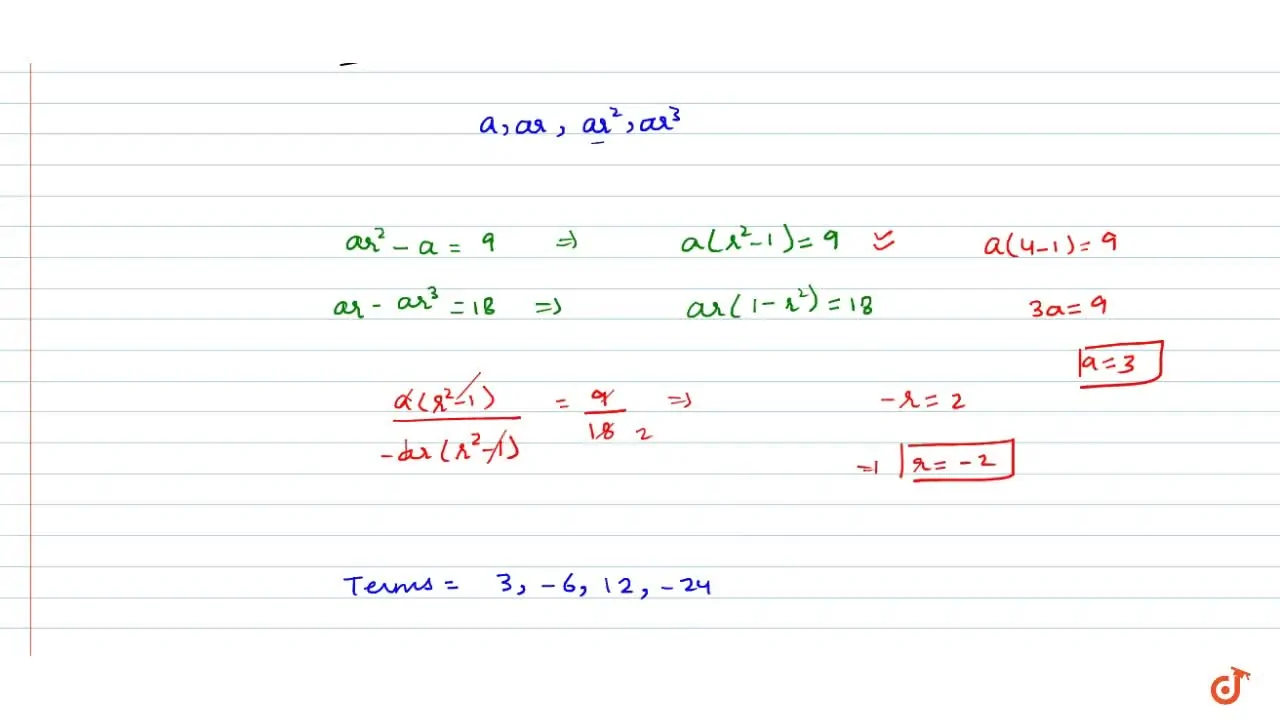
Find four numbers forming a geometric progression in which the third term is greater than the first term by 9, and the second term is greater than the 4thby 18.
Let a be the first term and r be the common ratio of G.P.
We know that,
nth term of G.P. =arn−1
i.e. an=arn−1
It is given that, third term is greater than the first term by 9
i.e. a3=a1+9
ar3−1=a+9
ar2=a+9 .....(1)
Also given that, and the second term is greater than the 4thby 18
i.e. a2=a4+18
ar2−1=ar4−1+18
ar=ar3+18 .....(2)
We need to calculate a and r
Multiplying (1) by r
r(ar2)=r(a+9)
ar3=ar+9r ....(3)
Putting ar3=ar+9r in (2)
ar=(ar+9r)18
ar−ar=9r+18
0=9r+18
−9r=18
r=−2
Put r=−2 in (1)
ar2=a+9
a(−2)2=a+9
a×4=a+9
4a−a=9
3a=9
a=3
Hence, a=3 and r=−2
We know that,
nth term of G.P.=arn−1
1st term of G.P.=a=3
2nd term of G.P.=ar2−1
a2=3×(−2)2−1=3×(−2)=−6
3rd term of G.P.=ar3−1
a3=3×(−2)3−1=3×(−2)2=12
4th term of G.P.=ar4−1
a4=3×(−2)4−1=3×(−2)3=−24
Hence G.P. is 3,−6,12,−24.....
Related Playlists
- BOOK - NCERTCHAPTER - SEQUENCES AND SERIESEXERCISE - EXERCISE 9.114videos
SEQUENCES AND SERIES
- BOOK - NCERTCHAPTER - SEQUENCES AND SERIESEXERCISE - EXERCISE 9.56videos
SEQUENCES AND SERIES
- BOOK - NCERTCHAPTER - SEQUENCES AND SERIESEXERCISE - EXERCISE 9.218videos
SEQUENCES AND SERIES
- BOOK - NCERTCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - EXERCISE 2.35videos
RELATIONS AND FUNCTIONS
- BOOK - NCERTCHAPTER - SETSEXERCISE - EXERCISE 1.57videos
SETS
Similar Questions
Find four numbers forming a geometric progression in which the third term is greater than the first term by 9, and the second term is greater than the 4thby 18.
View SolutionFind four numbers forming a geometric progression in which the third term is greater than the first term by 9, and the second term is greater than the 4th by 18.
View SolutionFind four numbers forming a geometric progression in which the third term is greater than the first terms by 9, and second term is greater than the 4th by 18.
View SolutionFind the 4 terms in G .P. in which 3rd term is 9 more than the first term and 2nd term is 18 more than the 4th term.
View SolutionIn an A.P. the third term is four times the first term, and the sixth term is 17 , find the series.
View SolutionThe third term of a geometric progression is 4. Then find the product of the first five terms.
View SolutionThe first term of a geometric progression is equal to b−2, the third term is b+6, and the arithmetic mean of the first and third term to the second term is in the ratio 5:3. Find the positive integral value of b.
View SolutionA number is greater than 3 but less than 9 also it is greater than 6 but less than 9.
View SolutionFind the sum of the following geometric progression: 2,6,18… to 7 terms
View SolutionFor a series in geometric progression, the first term is a and the second term is 3a. The common ratio of the series is _______.
View Solution