The scalar and vector products of two vectors are 48√3 and 144 respectively. What is angle between the two vectors?Solution in Telugu
θ=60∘
Topper's Solved these Questions
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - NUMERICAL EXXERCISE (LEVEL -2)
21 Videos
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
9 Videos
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
12 Videos
- ELECTROSTATICSBOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
4 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
6 Videos
Similar Questions
The width of a river is 3√3 km. A boat has a velocity of √3 km/s and starts to cross the river in a direction perpendicular to the bank of the river. If the river is flowing with a velocity 2km/sec, find the distance drifted by the boat.
View SolutionFind the components of vector ¯¯¯A=2ˆi+3ˆj along the directions of ˆi+ˆjandˆi−ˆj
View SolutionIf the vectors 2ˆi+2ˆj−ˆkand3ˆi−6ˆj+nˆk are mutually perpendicular, find the value of n ?
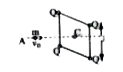
View SolutionFour identical point charges are fixed at the four corners of a square of a side length l. Another charged particle of mass m and charge +q is projected towards centre of square from a large, distance along the line perpendicular to plane of square. The minimum value of initial velocity V0 in m/s required to cross the square is? (m=1gm.l=4√2m,Q=1μc,q=0.5μc)
View SolutionA fish is at a depth of 12 cm in water is viewed by an observer on the bank of a lake. To what height the image of the fish is raised in cm ?
View SolutionThe magnitude of scalar and vector products of two vectors are 48√3 and 144 respectively. What is the angle between the two vectors?
View SolutionThe magnitude of scalar and vector products of two vectors are 144 and 48sqrt(3) respectivley. What is the angle between the two vectors?
View SolutionThe modulus of the vector product of two vector is (1)/(sqrt(3) times their scalar product . The angle between vectors is
View SolutionThe magnitude of the vector product of two vectors is √3 times their scalar product. The angle between the two vectors is
View SolutionRepresent the angle between two vectors in terms of the scalar product of those vectors.
View SolutionIf the scalar and vector products of two vectors vecA and vecB are equal in magnitude, then the angle between the two vectors is
View SolutionThe magnitude of the vector product of two vectors is 4. The magnitude of their scalar product is 4sqrt(3) . The angle between the two vectors is :
View SolutionThe magnitude of the vector product of two vectors is sqrt(3) times their scalar product. The angle between the vectors is :
View SolutionThe magnitude of the vector product of two vectors is 4. The magnitude of their scalar product is 4sqrt3 . The angle between the two vectors is :
View SolutionThe magnitude of scalar and vector products of two vector are 48 sqrt(3) and 144 respectively. What is the angle between the two vectors ?
View Solution
