An enemy plane is flying horizontally at an altitude of 2 km with a speed of An army man with an anti-aircraft gun on the ground sights hit enemy plane when it is directly overhead and fires a shell with a muzzle speed of . Ar what angle with the vertical should the gun be fired so as to hit the plane ?Solution in Telugu
Let G be the position of the gun and E that of the enemy plane flying horizontally with speed
, when the shell is fired with a speed is
Let the shell hit the plane a point P and let t be the time taken for the shell to hit the plane. It is clear that the shell will hit the plane, if the horizontal distance EP travelled by the plane in time t = the distance travelled by the shell in the horizontal direction in the same time,
i.e. or
or
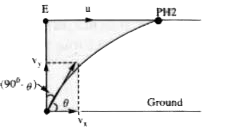
or
Therefore, angle with the vertical
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - SHORT ANSWER TYPE QUESTIONS
6 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
10 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Circular Motion)
7 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
Similar Questions
Show that the trajectory of an object thrown at certain angle with the horizontal is a parabola .
View SolutionWhat is the acceleration of a projectile at the top of its trajectory ?
View SolutionA particle ‘P is moving in a circle of radius "r" with uniform speed v. AB is the diameter of circle and 'C' is the centre. The angular velocity of P about A and C are in the ratio.
View SolutionGive two examples of the motion of big objects where the object can be treated as a particle and where it cannot be?
View SolutionAn enemy plane is flying horizontally at an altitude of 2km with a speed of 300ms−1.An army man with an anti-aircraft gun on the ground sights enemy plane when it is directly overhead and fires a shell with a muzzle speed of 600ms−1.At what angle with the vertical should the gun be fired so as to hit the plane?
View SolutionA fighter plane flying horizontally at an altitude of 2km with speed of 540 kmph passes directly over head an anti aircraft gun.If the gun can fire a bullet at the muzzle speed of 500ms−1,at what angle with the vertical the gun should fire the bullet so that the bullet hits the plane?
View SolutionAn enemy air plane is flying horizontally at an altitude of 2.7km with a speed of 250ms−1 .An army man on the ground observed it when it is directly over his head.He fired an anti-aircraft gun with a muzzle speed of 500ms−1 ,such that the shell hits the plane.At what angle with the horizontal,gun should be fired?
View SolutionA fighter plane flying horizontally at an altitude of 1.5 km with speed 720 km/h passes directly overhead an anti - aircraft gun . At what angle from the vertical should the gun be fired for the shell with muzzle speed 600"ms"^(-1) to hit the plane ? At what minimum altitude should the pilot fly the plane to avoid being hit ? (Take g= 10 " ms "^(-2)) .
View SolutionA fighter plane flying horizontally at an altitude of 1.5 km with speed 720 km/h passes directly overhead an anti - aircraft gun . At what angle from the vertical should the gun be fired for the shell with muzzle speed 600ms−1 to hit the plane ? At what minimum altitude should the pilot fly the plane to avoid being hit ? (Take g=10 ms −2).
View SolutionA fighter plane flying horizontally at an altitude of 1.5 km with speed 720 km/h passes directly overhead an anti - aircraft gun . At what angle from the vertical should the gun be fired for the shell with muzzle speed 600"ms"^(-1) to hit the plane ? At what minimum altitude should the pilot fly the plane to avoid being hit ? (Take g= 10 " ms "^(-2)) .
View SolutionA fighter plane flying horizontally at an altitude of 1.5 km with speed 720 km/h passes directly overhead an anti - aircraft gun . At what angle from the vertical should the gun be fired for the shell with muzzle speed 600ms−1 to hit the plane ? At what minimum altitude should the pilot fly the plane to avoid being hit ? (Take g=10 ms −2).
View SolutionA fighter plane flying horizontally at an altitude of 1.5 km with speed 720 km h−1 passes diretly overhead an anti-aircraft gun. At what angle from the vertical should the gun he fired for the shell with muzzle speed 600 m s−1 to hit the plane. (Take g = 10 m s−1)
View SolutionAn enemy plane is flying horizontally at an altitude of 2 km with a speed of 300 ms^(-1) An army man with an anti-aircraft gun on the ground sights hit enemy plane when it is directly overhead and fires a shell with a muzzle speed of 600 ms^(-1) . Ar what angle with the vertical should the gun be fired so as to hit the plane ?
View SolutionA fighter plane flying horizontally at an altitude of 1.5 km with speed 720 km/h passes directly overhead an antiaircraft gun. At what angle from the vertical should the gun be fired for the shell with muzzle speed 600 ms to hit the plane? At what minimum altitude should the pilot fly the plane to avoid being hit ? (Take g=10ms−2).
View SolutionAn enemy plane is flying horizontally at an altitude of 2km with a speed of 300 ms^(-1) . An army man with an anti-aircraft gun on the ground sights hit enemy plane when it is directly overhead and fires a shell with a muzzle speed of 600 ms^(-1) . At what angle with the vertical should the gun be fired so as to hit the plane ?
View Solution
