Question

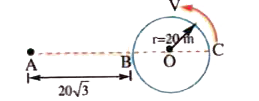
A particle is moving along a vertical circle of radius r = 20 m with a constnat vertical circle of radius r = 20 m with a constnat speed v = 31.4m/s as shown is figure . Straight line ABC is horizontal and passes throught the centre of the circle . A shell if fired from point A at the instant when the particle is at C. If distance AB is $20\sqrt{3}$ m and the shell collide with the particle at B , then prove $\tan \theta =\frac{{(2n-1)}^2}{\sqrt{3}}$.where n is an interger. Further , show that smallest value of $\theta$ is ${30}^{\circ }$.

Explanation

As at the time of firing of th shell , the particle was at C and the shell collides with it at B, therefore the number of the revolution completed by the particle is odd multiple of half i.e., (2n-1)/2, where n is an integer .
Let the time period of the particle , then
`T = (2pir)/(v) = (2xx3014 xx 20)/(31.4) = 4 ` second.
IF the time of the flight of the shell , then t = time of `[(2n-1)//2]` revolutions of the particle
` = ((2-1))/(2) xx 4 = 2(2n -1)` second .
for a projectile , the time of flight is given by ` t = (2u sin theta)/(g)`
Hence `(2 u sin theta)/(g) = 2(2n-1)" ".......(i)`
The range of the projectile is given by `R = (u^(2) sin 2 theta)/(g)`
Hence `(u^(2) sin 2 theta)/(g) = 20 sqrt(3)" "........(ii)`
From equation (i) and (ii) `tan theta = ((2n-1)^(2))/(sqrt(3))`
For `theta` to be smallest , n =1 so `tan theta =((2n-1))/(sqrt(3))`