A stone is projected vertically up from the ground with velocity . The interval of time between the two instants at which the stone is at a height of 60 m above the ground is Solution in Telugu
4 s
6 s
8 s
12 s
The correct Answer is:A
Step by step video, text & image solution for A stone is projected vertically up from the ground with velocity 40 ms^(-1) . The interval of time between the two instants at which the stone is at a height of 60 m above the ground is (g = 10 "ms"^(-2)) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - PRACTICE EXERCISE
58 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - problems
47 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - EXERCISE -I
58 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III
45 Videos
Similar Questions
If a cyclist takes two minutes to compete half revolution on a circular path of 120 m radius. What is the average speed?
View SolutionFind the covered by the particles,in the following figures,(i) and (ii) while a particle travels from A to B as shown by the arrows.
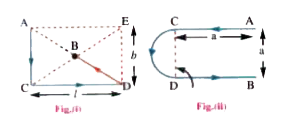
View SolutionThe ratio of the numerical values of the average velocity and average speed of a body is always
View SolutionA particle is projected over a triangle from one end of a horizontal base and garzing the vertex falls on the other end of the base . If α and β the base angles and θ the angle of projection then show that tanθ=tanα+tanβ.
View SolutionA proton, a deuteron and an a particle having same momentum enter a uniform magnetic field at right angles to the field. Then the ratio of their angular momenta during their motion in the magnetic field is
View SolutionA stone is thrown horizontally with velocity g ms^(-1) from the top of a tower of height g metre. The velocity with which it hits the ground is ("in" ms^(-1))
View SolutionA stone is dropped from a height of 100m .At the same instant another stone is projected vertically upwards with velocity of 50ms^(-1) .If g=10ms^(-2) ,the height from the ground at which the 2 stones meet is
View SolutionA stone is projected from a point on the ground in such a way so as to hit a bird at height 3h and could attain a maximum height 4h above the ground. If at the instant of projection, the bird flies away horizontally with speed 10 ms^(-1) and the stone still hits the bird while descending, then the horizontal velocity of stone is
View SolutionA balloon is going vertically upward with a velocity of 15 ms−1. When it is at a height of 50 m above the ground a stone is gently dropped from it. The stone will reach the ground in time (take g = 10 ms−2):
View SolutionA balloon is rising vertically up with a velocity of 29 ms−1. A stone is dropped from it and it reaches the ground in 10 seconds. The height of the balloon when the stone was dropped from it is
View SolutionA stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100 ms^(-1) . Find when and where the two stones meet ?
View SolutionA stone is thrown horizontally with velocity g ms^(-1) from the top of a tower of height g metre. The velocity with which it hits the ground is (in ms^(-1) )
View SolutionA stone is projected vertically up from the ground with velocity 40 ms^(-1) .The interval of time between the two instants at which the stone is at a height of 60 m above the ground is
View SolutionA stone is projected vertically up from the ground with velocity 40ms−1 . The interval of time between the two instants at which the stone is at a height of 60 m above the ground is (g=10ms−2)
View SolutionA stone is thrown horizontally with velocity g ms^(-1) from the top of a tower of height g metre. The velocity with which it hits the ground is (in ms^(-1) )
View Solution
