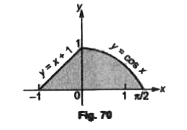
The area bounded by $y = x + 1 and y = cos x$ and the x - axis, is

A

1 sq. units

B

$\frac{3}{2} sq. units$

C

$\frac{1}{4} sq. units$

D

$\frac{1}{8} sq. units$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:B

To find the area bounded by the curves $y = x + 1$ , $y = cos x$ , and the x-axis, we will follow these steps:
Step 1: Identify the points of intersection
We need to find the points where the curves $y = x + 1$ and $y = cos x$ intersect. To do this, we set them equal to each other:
$x + 1 = cos x$
This equation cannot be solved algebraically, so we will find the points of intersection graphically or numerically.
Step 2: Graph the functions
We can sketch the graphs of $y = x + 1$ and $y = cos x$ to identify the points of intersection. The graph of $y = cos x$ oscillates between -1 and 1, while the line $y = x + 1$ is a straight line with a slope of 1.
From the graph, we can observe that the curves intersect at approximately $x = - 1$ and $x = 0$ .
Step 3: Set up the integral for the area
The area $A$ between the curves from $x = - 1$ to $x = 0$ can be calculated using the integral:
$A = \int_{- 1}^{0} (cos x - (x + 1)) d x$
Step 4: Simplify the integrand
We simplify the integrand:
$cos x - (x + 1) = cos x - x - 1$
Step 5: Evaluate the integral
Now we will compute the integral:
$A = \int_{- 1}^{0} (cos x - x - 1) d x$
This can be split into three separate integrals:
$A = \int_{- 1}^{0} cos x d x - \int_{- 1}^{0} x d x - \int_{- 1}^{0} 1 d x$
Calculating each integral separately:
1. Integral of $cos x$ :
$\int cos x d x = sin x so {(sin x)}_{- 1}^{0} = sin (0) - sin (- 1) = 0 - (- sin (1)) = sin (1)$
2. Integral of $x$ :
$\int x d x = \frac{x^{2}}{2} so {(\frac{x^{2}}{2})}_{- 1}^{0} = 0 - \frac{(- 1)^{2}}{2} = - \frac{1}{2}$
3. Integral of 1:
$\int 1 d x = x so {(x)}_{- 1}^{0} = 0 - (- 1) = 1$
Putting it all together:
$A = sin (1) - (- \frac{1}{2}) - 1$
$A = sin (1) + \frac{1}{2} - 1 = sin (1) - \frac{1}{2}$
Final Step: Calculate the area
Thus, the area bounded by the curves and the x-axis is:
$A = sin (1) - \frac{1}{2}$

Updated on:18/9/2024

|

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

If $A_{1}$ is the area bounded by the curve y = cos x and $A_{2}$ is the area bounded by y = cos 2 x along with x - axis from x = 0 and $x = \frac{π}{0}$ , then
A $A_{1} = \frac{\sqrt{3}}{2}, A_{2} = \frac{\sqrt{3}}{4}$
B $A_{1} = \frac{\sqrt{3}}{2}, A_{2} = \frac{1}{2}$
C $\frac{A_{1}}{A_{2}} = \frac{2}{1}$
D $\frac{A_{1}}{A_{2}} = \frac{\sqrt{3}}{1}$
The area bounded by $y = - x^{2} + 1$ and the x-axis is
A $\frac{1}{3}$
B $\frac{2}{3}$
C $\frac{4}{3}$
D $\frac{8}{3}$
The area bounded by $y = max (x^{2}, x^{4}), y = 1$ and the y - axis from $x = 0 to x = 1$ is
A3 sq. units
B $\frac{3}{2}$ sq. units
C $\frac{2}{3}$ sq. units
D $\frac{1}{2}$ sq. units

MTG-WBJEE-APPLICATION OF INTEGRALS-WE JEE PREVIOUS YEARS QUESTIONS (CATEGORY 3 : ONE OR MORE THAN ONE OPTION CORRECT TYPE)

Home
Ask Doubt
Profile