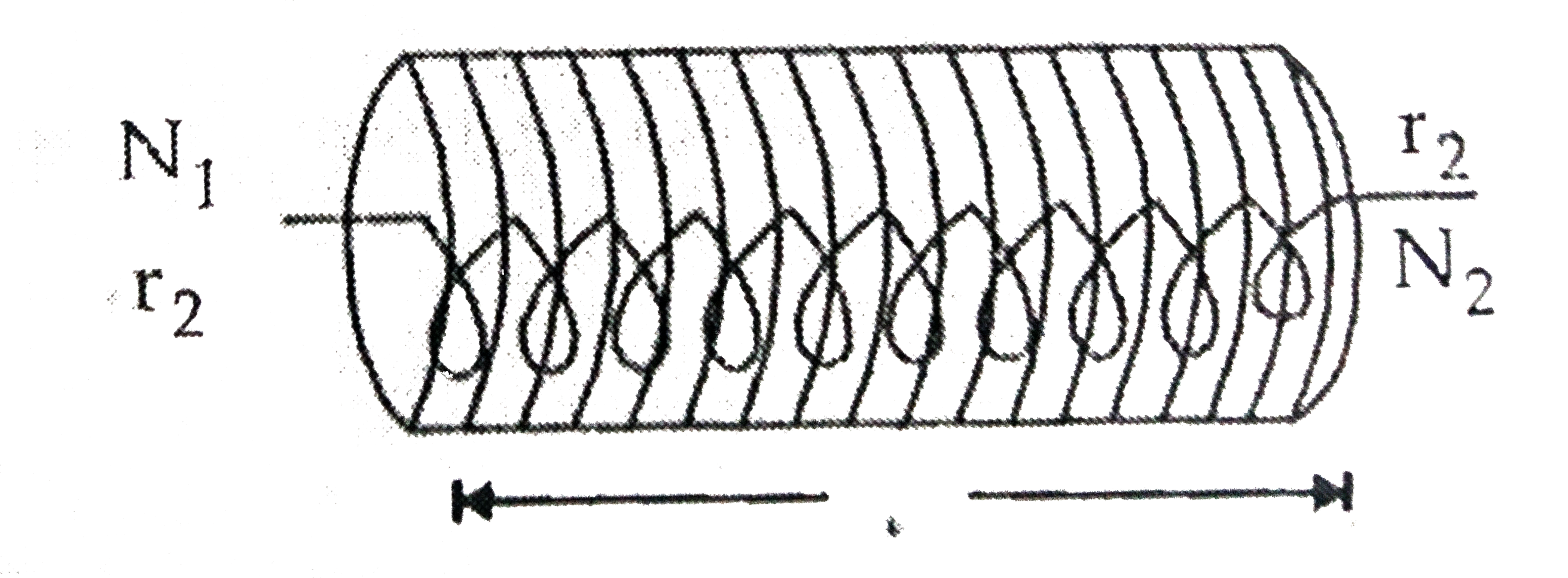
Define mutual inductance between two long coaxial solenoids. Find out the expression for the mutual of inner solenoid of length 1 having the radius $r_{1}$ and the number of turns $n_{1}$ per unit length due to the second other solenoid of same length and $n_{2}$ number of turns per unit length.

Video Solution

Text Solution

Verified by Experts

Let l be the length of each solenoid $S_{1} and S_{2} . N_{1} and N_{2}$ be the total number of turn os $S_{1} and S_{2}$ .
Magnetic field in $S_{1} = B_{1} = \frac{μ_{0} N_{1} N_{1}}{l}$
Magnetic flux linked with each turn of $S_{2} = B_{1} \times$ area of each turn $= B_{1} π r_{1}^{2}$
Total magnetic flux linked with $S_{2} = B_{1} π r_{1}^{2} N_{2}$
$∴ ϕ_{2} (\frac{μ_{0} N_{l} I_{l}}{l}) π r_{1}^{2} N_{2} = \frac{μ_{0} N_{1} N_{2} π r_{1}^{2} I_{l}}{l}$
But magnetic flux linked with $S_{2}$ is due to $I_{1}$
$∴ ϕ_{2} \propto I_{1} or ϕ_{2} = M I_{1}$
Where M is the mutual inductance of $S_{2} and S_{1}$
$∴ M I_{1} = \frac{μ_{0} N_{1} N_{2} π r_{1}^{2} I_{1}}{l} ∴ M = μ_{0} n_{1} n_{2} π r_{1}^{2} I$ .

Updated on:21/07/2023

The expression for magnetic induction inside a solenoid of length $L$ carrying a current $I$ and having $N$ number of turns is
A $\frac{μ_{0}}{4 π} \frac{N}{L I}$
B $μ_{0} N I$
C $\frac{μ_{0}}{4 π} N L I$
D $μ_{0} \frac{N}{L} I$
The self inductance L of a solenoid depends on the number of turns per unit length 'n' as
A $L \infty n$
B $L \infty n^{2}$
C $L \infty n^{- 1}$
D $L \infty n^{- 2}$
Find the mutual inductance of two long concentric solenoids for a length I. The area of cross-section of inner solenoid is A and number of turns per unit length are $n_{1}$ and $n_{2}$ .
A $μ_{0} π b^{2} n_{1} n_{2} L$
B $μ_{0} π a^{2} n_{1} n_{2} L^{2}$
C $μ_{0} π a^{2} n_{1} n_{2} L$
D $μ_{0} b^{2} n_{1} n_{2} L^{2}$