Two point charges, q1=10×10−8C and q2=−2×10−8C are separated by a distance of 60 cm in air.
(i) Find at what distance from the 1st charge, q1, would the electric potential be zero.
(ii) Also calculate the electrostatic potential energy of the system.
Let's solve the given problem step by step.
Given:
- q1=10×10−8C
- q2=−2×10−8C
- Distance between the charges d=60cm=0.6m
Part (i): Finding the distance from q1 where the electric potential is zero.
1. Step 1: Set up the equation for electric potential.
The electric potential V at a point due to a charge q is given by:
V=kqr
where k is Coulomb's constant (9×109Nm2/C2) and r is the distance from the charge.
2. Step 2: Define the distances.
Let the point where the potential is zero be at a distance x from q1. Then, the distance from q2 to this point is 0.6−x.
3. Step 3: Write the equation for zero potential.
The total potential at the point is the sum of the potentials due to q1 and q2:
V=kq1x+kq20.6−x=0
4. Step 4: Substitute the values and solve for x.
9×109×10×10−8x+9×109×(−2)×10−80.6−x=0
Simplifying:
10x−20.6−x=0
10x=20.6−x
Cross-multiplying:
10(0.6−x)=2x
6−10x=2x
6=12x
x=612=0.5m
So, the distance from the first charge q1 where the electric potential is zero is 0.5m.
Part (ii): Calculating the electrostatic potential energy of the system.
1. Step 1: Use the formula for electrostatic potential energy.
The electrostatic potential energy U of a system of two point charges is given by:
U=kq1q2r
where r is the distance between the charges.
2. Step 2: Substitute the values.
U=9×109×10×10−8×(−2)×10−80.6
Simplifying:
U=9×109×10×(−2)×10−160.6
U=−180×10−70.6
U=−30×10−7J
U=−3×10−6J
So, the electrostatic potential energy of the system is −3×10−6J.
Summary:
1. The distance from the first charge q1 where the electric potential is zero is 0.5m.
2. The electrostatic potential energy of the system is −3×10−6J.
- q1=10×10−8C
- q2=−2×10−8C
- Distance between the charges d=60cm=0.6m
The electric potential V at a point due to a charge q is given by:
V=kqr
where k is Coulomb's constant (9×109Nm2/C2) and r is the distance from the charge.
Let the point where the potential is zero be at a distance x from q1. Then, the distance from q2 to this point is 0.6−x.
The total potential at the point is the sum of the potentials due to q1 and q2:
V=kq1x+kq20.6−x=0
9×109×10×10−8x+9×109×(−2)×10−80.6−x=0
Simplifying:
10x−20.6−x=0
10x=20.6−x
Cross-multiplying:
10(0.6−x)=2x
6−10x=2x
6=12x
x=612=0.5m
The electrostatic potential energy U of a system of two point charges is given by:
U=kq1q2r
where r is the distance between the charges.
U=9×109×10×10−8×(−2)×10−80.6
Simplifying:
U=9×109×10×(−2)×10−160.6
U=−180×10−70.6
U=−30×10−7J
U=−3×10−6J
1. The distance from the first charge q1 where the electric potential is zero is 0.5m.
2. The electrostatic potential energy of the system is −3×10−6J.
Topper's Solved these Questions
Similar Questions
Two point charges, q1=10×10−8Candq2=−2×10−8C, are separated by a distance of 60 cm in air.
Find at what distance from the 1st charge q1, would the electric potential be zero.
View SolutionTwo point charges 20×10−6Cand−4×10−6C are separated by a distance of 50cm in air, Find (i) the point on line joining the charges, where the electrostatic potential is zero (ii) Also, Calculate the electrostatic potential energy of the system.
View SolutionTwo point charges, q1=10×10−8Candq2=−2×10−8C, are separated by a distance of 60 cm in air.
Also calculate the electrostatic potential energy of the system.
View SolutionTwo point charges q1=2×10−3C and q2=−3×10−6C are separated by a distance x=10 cm. Find the magnitude and nature of the force between the two charges.
View SolutionTwo point charges 4μCand−2μC are separated by a distance of 1m in air. Calculate at what point on the line joining the two charges is the electric potential zero ?
View SolutionTwo point charges +4μCand−2μC are separated by a distance of 1 m in air. Calculate at what point on the line joining the two charges is the electric potential zero.
View SolutionTwo point charges +5×10−19Cand+20×10−19C are separated by a distance of 2m. The electric field intensity will be zero at a distance d=………. From the charges of 5×1019C.
View SolutionTwo charge _q and -3q are placed at a distance of 1 m apart.Find out the points on the line joing two charges, where electric potential is zero.
View SolutionTwo point charge q1=−10×10−6C and q2=15×10−6 are 40 cm apart as shown in figure. Find the potential difference between the point P and Q.
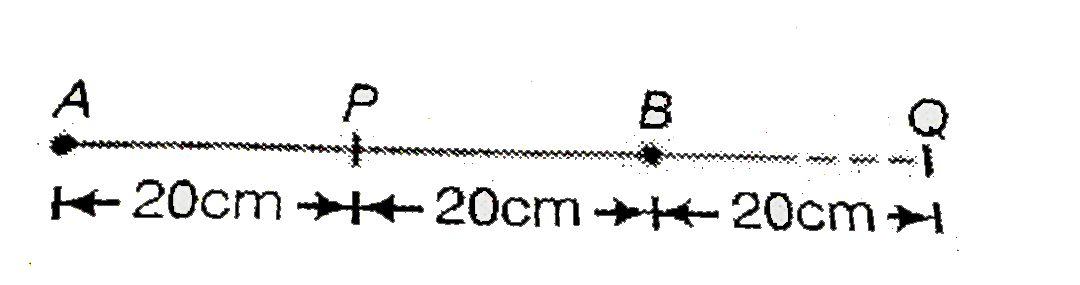
View SolutionPotential energy of a system of charges q1 and q2 which are separated by a distance r is ?
View Solution
