In a car lift , compressed air with a gauge pressure of 4.0×105 Pa is used to raise a piston with a circular cross-sectional area . If the radius of the piston is 0.17 m , what is the maximum mass that can be raised using this piston ?
530 kg
3700 kg
9800 kg
22,000 kg
The correct Answer is:B
To solve the problem of determining the maximum mass that can be raised using a piston in a car lift, we can follow these steps:
Step 1: Understand the relationship between pressure, force, and area
According to Pascal's law, the pressure exerted on a confined fluid is transmitted undiminished throughout the fluid. The relationship between pressure (P), force (F), and area (A) is given by the formula:
P=FA
Where:
- P is the pressure in Pascals (Pa)
- F is the force in Newtons (N)
- A is the area in square meters (m²)
Step 2: Identify the given values
From the problem, we have:
- Gauge pressure Pg=4.0×105Pa
- Radius of the piston r=0.17m
Step 3: Calculate the area of the piston
The area A of a circular piston can be calculated using the formula:
A=πr2
Substituting the value of r:
A=π(0.17)2
A≈3.14×0.0289
A≈0.049m2
Step 4: Relate the force to mass
The force F exerted by the piston can also be expressed in terms of mass m and gravitational acceleration g:
F=mg
Where g≈9.8m/s2.
Step 5: Set up the equation using pressure
Using the relationship between pressure, force, and area, we can substitute F with mg:
Pg=mgA
Rearranging this gives:
m=Pg⋅Ag
Step 6: Substitute the values into the equation
Now we can substitute Pg, A, and g into the equation:
m=(4.0×105)⋅(0.049)9.8
Calculating this step-by-step:
1. Calculate Pg⋅A:
Pg⋅A=4.0×105×0.049≈19600N
2. Now divide by g:
m≈196009.8≈2000kg
Step 7: Final calculation
After calculating, we find:
m≈2000kg
Conclusion
The maximum mass that can be raised using the piston is approximately 2000 kg.
---
According to Pascal's law, the pressure exerted on a confined fluid is transmitted undiminished throughout the fluid. The relationship between pressure (P), force (F), and area (A) is given by the formula:
- P is the pressure in Pascals (Pa)
- F is the force in Newtons (N)
- A is the area in square meters (m²)
From the problem, we have:
- Gauge pressure Pg=4.0×105Pa
- Radius of the piston r=0.17m
The area A of a circular piston can be calculated using the formula:
A≈3.14×0.0289
A≈0.049m2
The force F exerted by the piston can also be expressed in terms of mass m and gravitational acceleration g:
Using the relationship between pressure, force, and area, we can substitute F with mg:
Now we can substitute Pg, A, and g into the equation:
Pg⋅A=4.0×105×0.049≈19600N
m≈196009.8≈2000kg
After calculating, we find:
The maximum mass that can be raised using the piston is approximately 2000 kg.
Topper's Solved these Questions
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - PRACTICE QUESTIONS (More than One Correct Choice Type )
10 Videos
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - PRACTICE QUESTIONS (Linked Comprehension )
9 Videos
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - PROBLEMS
68 Videos
- ELECTROMAGNETIC WAVES BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC WAVES EXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
Similar Questions
The pressure of a gas contained in a cylinder with a movable piston is 300 Pa. The area of the piston is 0.5m2. Calculate the force that is exerted on the piston.
View SolutionIn a car lift, compressed air exerts a force F1 on a small piston having a radius of 0.5cm. This pressure is transmitted to a second piston of radius 10.0 cm . If the mass of the car to be lifted is 1350 kg. calculate F1. What is the pressure necessary to ac complish this task?
View Solution
Knowledge Check
A piston of cross-sectional area 100cm2 is used in a hydraulic pressure to exert a force of 107 dyne on the water. The cross-sectional area of the other piston which support a truck of mass 2000 kg is
A9.8×102cm2B9.8×103cm2C1.96×103cm2D1.96×104cm2A piston of cross-sectional area 100cm2 is used in a hydraulic pressure to exert a force of 107 dyne on the water. The cross-sectional area of the other piston which support a truck of mass 2000 kg is
A100cm2B109cm2C1.96×104cm2D2×1010cm2
In car lift compressed air exerts a force F1 on a small piston having a radius of 5 cm. This pressure is transmitted to a second piston of radius 15 cm. If the mass of the car to be lifted is 1350 kg, what is F1? What is the pressure necessary to ac complish this task ?
View SolutionIn A double -acting steam engine, the avearge pressure of steam is 8×104Nm−2. The length of the stroke is 0.8 m and area of cross-section of the piston is 0.18m2. If the piston makes 6 revolution per second, calculate the horse power of the engine.
View SolutionIn the figure shown below, cylinder A has pump piston, whereas B and C cylinder have lift pistons. If the maximum weight that can be placed on the pump piston is 50 kgwt, what is the maximum weight that can be lifted by piston in the cylinder B? Find the total mechanical adventage.
(Take g 10 m s−2).
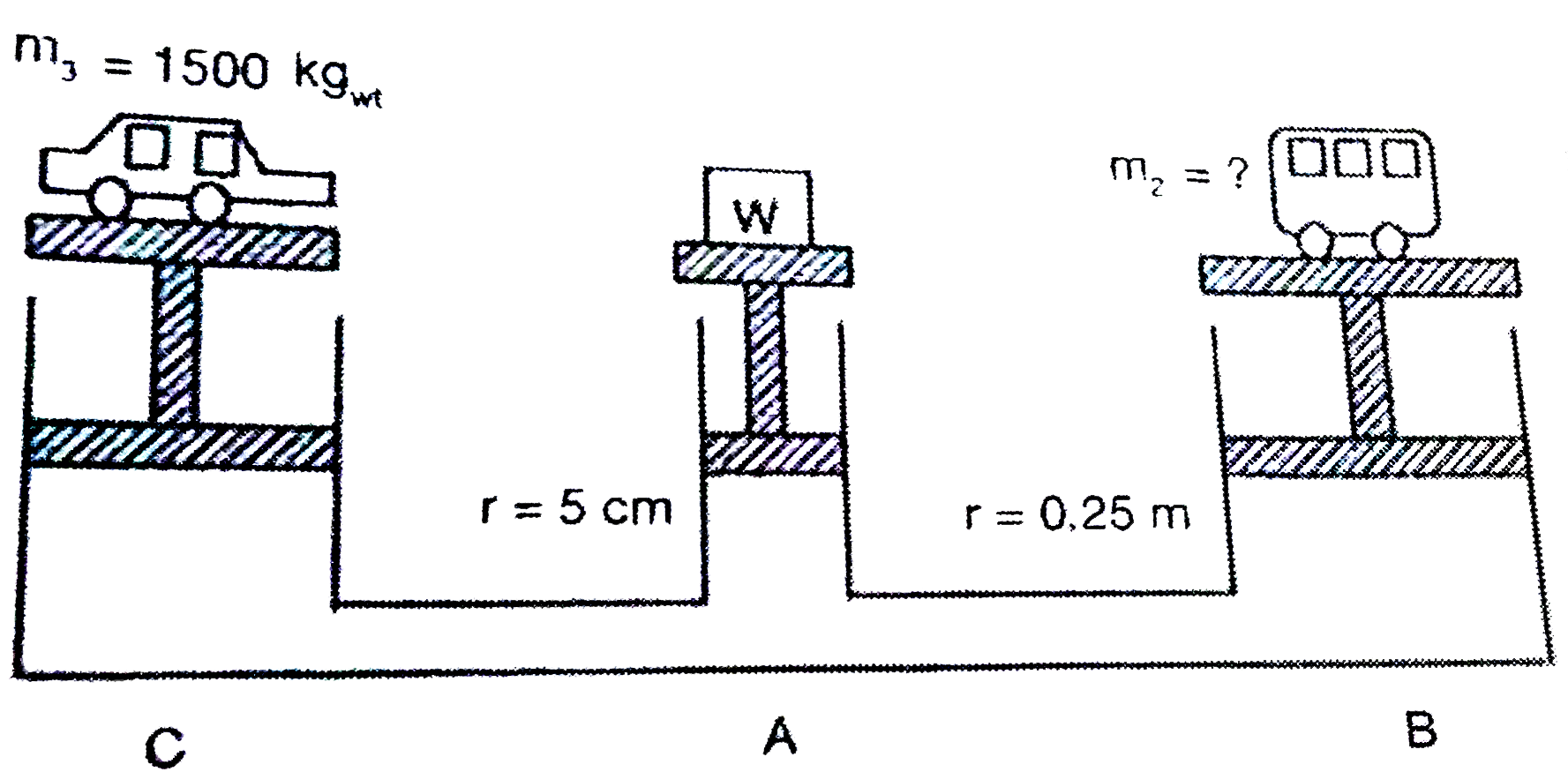
View SolutionA cylinder fitted with a spring loaded piston, shown in the figure, contains 0.01(m)3 gas at a pressure of 105Pa. The cross-sectional area of the piston is 0.05m2. Initially, the spring does not touch the piston but atmospheric pressure of 105Pa acts on the piston. The gas is slowly heated till the volume is increased to three times the original value. If the forcé constant of the spring is 25kNm, calculate the work done (in kJ ) by the gas.
'(##CEN_KSR_PHY_JEE_C15_E01_031_Q19##)'
View SolutionA pump in the basement of a garage is used to poerate a hyfraulin hack on the firest floor. The areas of the of the two piston is 3 m higher than the other one . How great a load can be lifted by a force of 10 kg applied to the lower piston ?
View Solution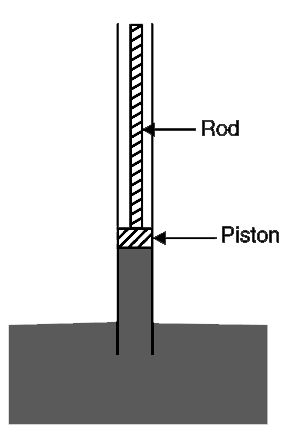
A tightly fitted piston can slide along the inner wall of a long cylindrical pipe. With the piston at the lower end of the pipe, the lower end of the pipe is dipped into a large tank, filled with water. Now the piston is pulled up with the help of the rod attached to it. water rises in the pipe along with the piston. Why? To what maximum height water can be raised in the pipe using this method? What will be the answer to your question if water is replaced with mercuty? Atmosphere pressure is Patm=1.01×105Pa.
View Solution
