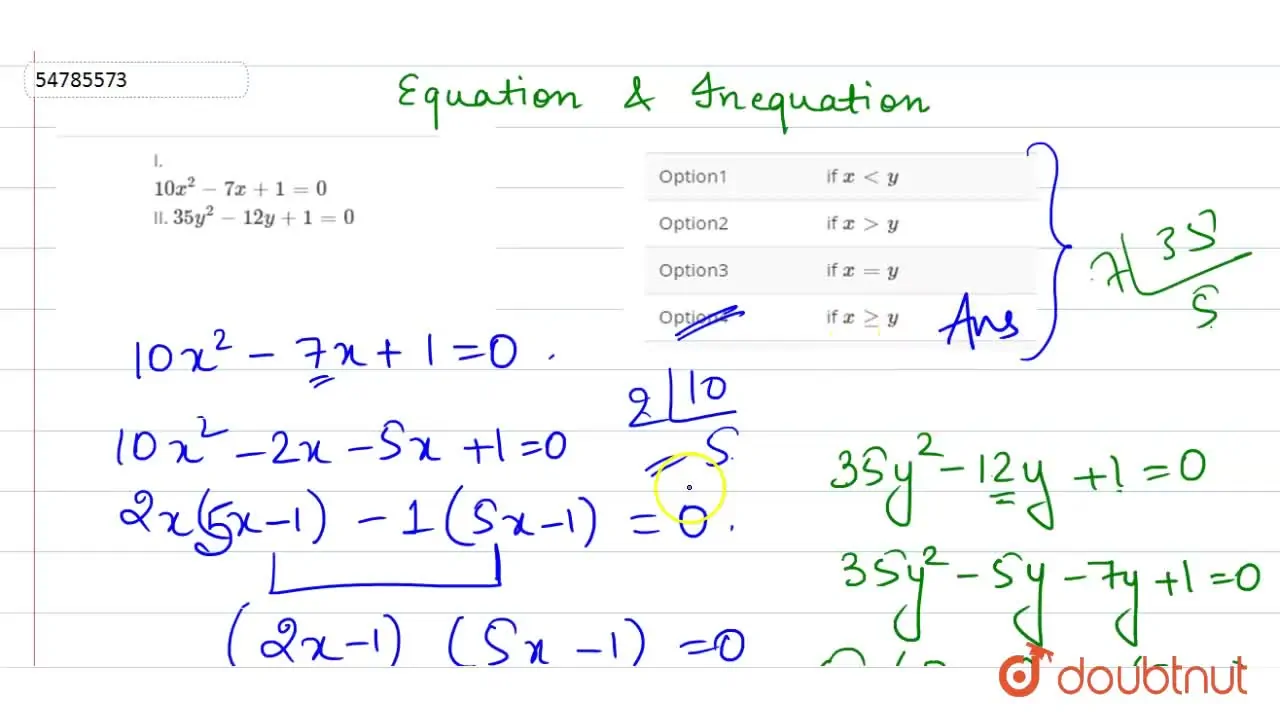
I. 10x2−7x+1=0
II. 35y2−12y+1=0
A
if x<y
B
if x>y
C
if x=y
D
if x≥y
Video Solution
Text Solution
The correct Answer is:D
I. 10x2−7x+1=0
or,10x2−5x−2x+1=0
or, (2x−1)(5x−1)=0
or,x=1/2,1/5
II. 35y2−12y+1=0
or,35y2−7y−5y+1=0
or,(5y−1)(7y−1)=0
or, y=1/5,1/7
Hence, x≥y
|
Updated on:21/07/2023
Related Playlists
Similar Questions
I.x2−7x+12=0 II.3y2−11y+10=0
View SolutionI.x2−7x+10=0 II.Y2−12y+35=0
View SolutionI. 3x2−7x+2=0
II. 2y2−11y+15=0View SolutionI.12x2−x−1=0
II. 20y2−41y+20=0View SolutionI.X2−8x+15=0 II.Y2−12y+36=0
View SolutionI. 12x2+17x+6=0 II.6y2+5y+1=0
View SolutionI12x2−x−1=0 II.20y2−41y+20=0
View SolutionI. 4x2−13x−12=0
II. y2−7y−60=0View SolutionI.x2−5x−14=0 II.Y2+7y+10=0
View SolutionI. 2x2+17x+35=0
II. 8y2−14y−15=0View Solution