Question

If the integers m and n are chosen at random between 1 and 100, then the probability that a number of the form $7^m+7^n$ is divisible by 5, equals (a) $\frac{1}{4}$ (b) $\frac{1}{7}$ (c) $\frac{1}{8}$ (d) $\frac{1}{49}$

Options

A. `(1)/(4)`
B. `(1)/(7)`
C. `(1)/(8)`
D. `(1)/(49)`

Answer

A

Explanation

`7^1=7,7^2=49,7^3 = 343,7^4=2401…`
Therefore for `7^r, r in N` the number ends at unit place 7,9,3,1,7…
`therefore 7^m+7^n` will be divisible by 5 if it end at 5 or 0.
But it cannot end at 5
Also for end at 5.
For this m and n should be as follows

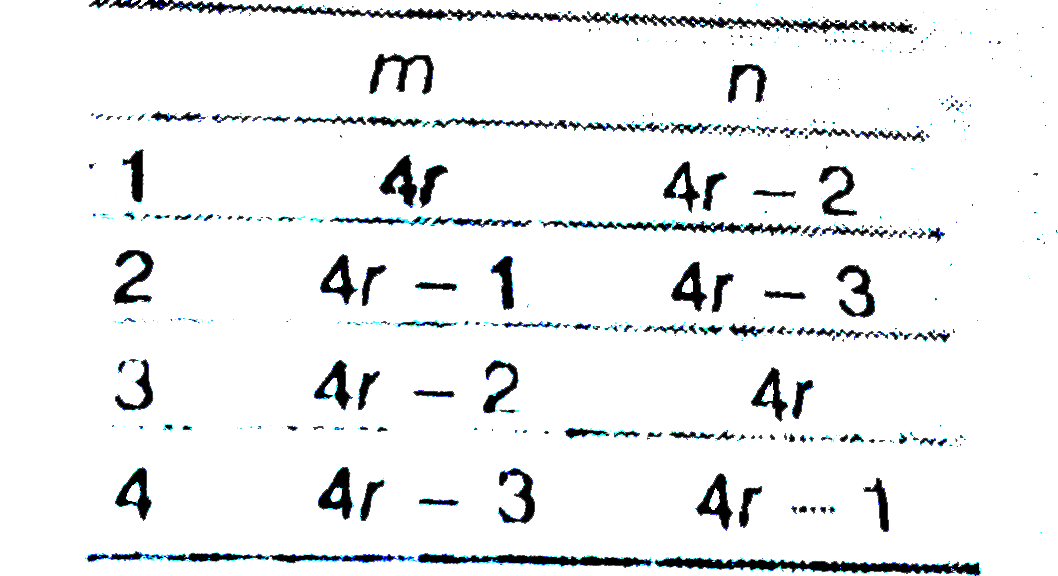
For any given value of m, there will be be 25 values of n Hence , the probability of the required event is
`(100xx 25)/(100xx100)=1/4`
Note:Power of prime numbers have cycclic numbers in their unit place.