Show that the area of the triangle on the Argand diagram formed by the

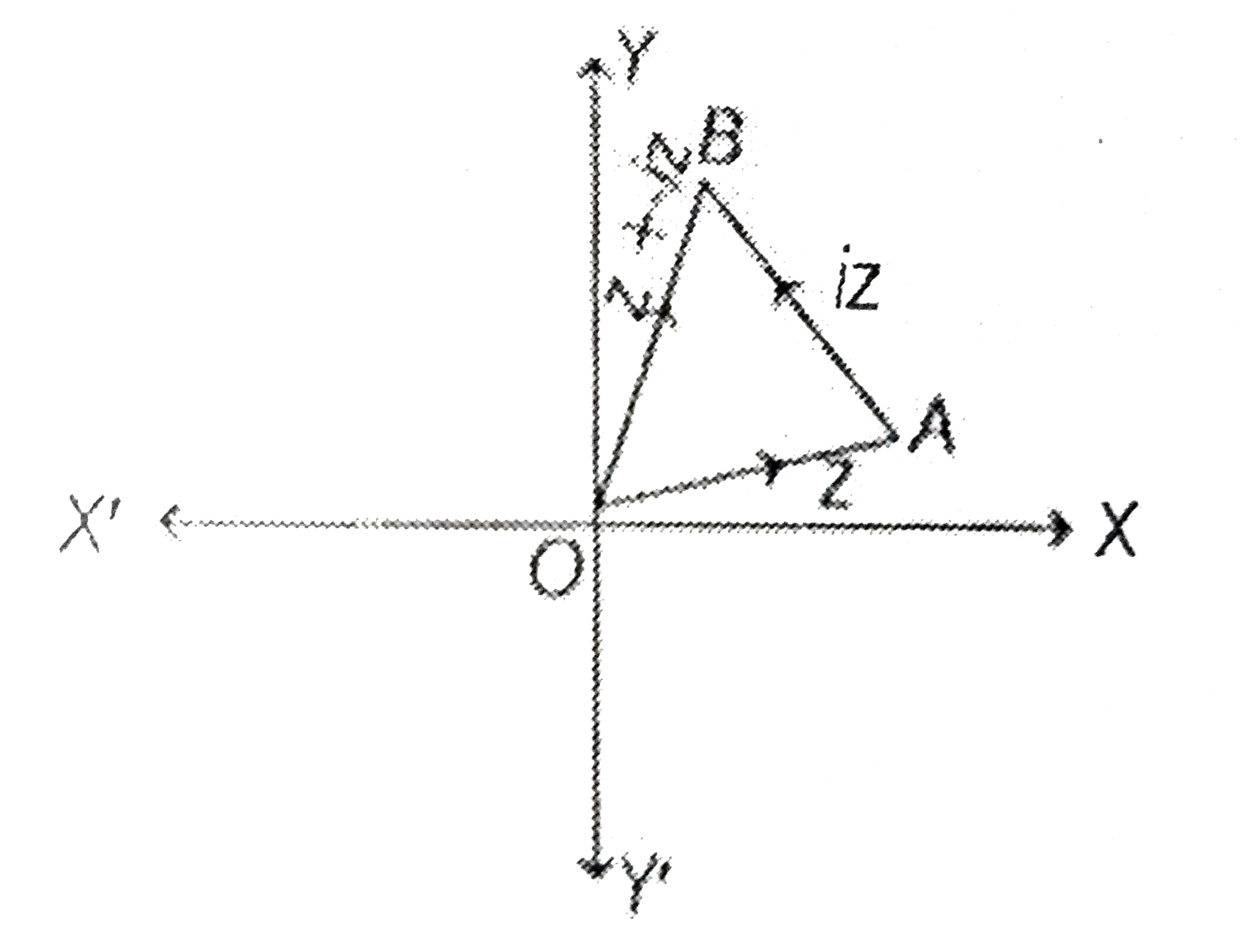
Area of the triangle in the argand diagram formed by the complex numbers z, iz, z +iz , where z = x+iy , is
A|z|
B ${| z |}^{2}$
C $2 {| z |}^{2}$
D $\frac{1}{2} {| z |}^{2}$
Area of the triangle in the argand diagram formed by the complex numbers z, iz, z +iz , where z = x+iy , is
A $| z |$
B ${| z |}^{2}$
C $2 {| z |}^{2}$
D $\frac{1}{2} {| z |}^{2}$
The area of the triangle formed by the complex numbers z, iz, z + iz in the Argand plane is
A $\frac{1}{2} {| z |}^{2}$
B $∣ ∣ z^{2} ∣ ∣$
C $2 ∣ ∣ z^{2} ∣ ∣$
DNone of these

Show that the area of the triangle on the Argand diagram formed by the complex number $z, i z a n d z + i z$ is $\frac{1}{2} {| z |}^{2}$