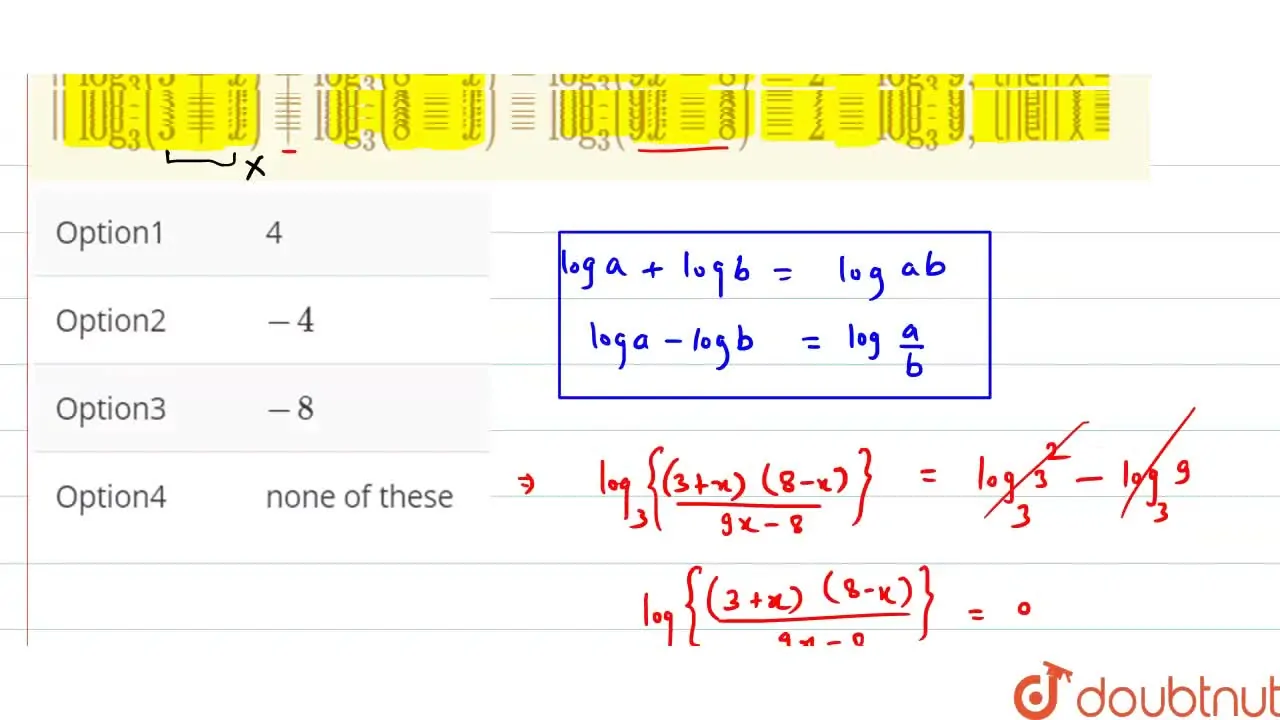
If log3(3+x)+log3(8−x)−log3(9x−8)=2−log39, then x =
A
4
B
−4
C
−8
D
none of these
Video Solution
Text Solution
The correct Answer is:A
|
Answer
Step by step video, text & image solution for If log_(3) ( 3 + x) + log_(3) (8 - x) - log_(3) ( 9x - 8) = 2 - log_(3) 9, then x = by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Related Playlists
- BOOK - MTG-WBJEECHAPTER - LOGARITHMS EXERCISE - WB JEE / WORKOUT (CATEGORY 3 : ONE OR MORE THAN ONE OPTION CORRECT TYPE )10videos
LOGARITHMS
- BOOK - MTG-WBJEECHAPTER - LOGARITHMS EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS10videos
LOGARITHMS
- BOOK - MTG-WBJEECHAPTER - LOGARITHMS EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS10videos
LOGARITHMS
- BOOK - MTG-WBJEECHAPTER - LIMITS AND CONTINUITYEXERCISE - WE JEE PREVIOUS YEARS QUESTIONS (CATEGORY 3 : ONE OR MORE THAN ONE OPTION CORRECT TYPE)2videos
LIMITS AND CONTINUITY
- BOOK - MTG-WBJEECHAPTER - MATRICES AND DETERMINANTS EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (CATEGORY 3 : ONE OR MORE THAN ONE OPTION CORRECT TYPE )3videos
MATRICES AND DETERMINANTS
Similar Questions
if log3(x−5)+log3(x+2)=log38 then x = ______
View SolutionIf log2x×log3x=log2x+log3x, then find x .
View SolutionIf log3x+log9x2+log27x3=9, then x =
View SolutionIf xlog3x=9, then x can be
View Solution(iii) log_(3)(3^(x)-8)=2-x
View SolutionSolve log3(3x−8)=2−x
View Solution√log3(3x2).log9(81x)=log9x3
View SolutionIf log9x+log4y=72 and log9x−log8y=−32, then x+y equals
View SolutionIf log4x+log8x2+log16x3=232, then logx8=
View Solutionlog3(5+x)+log88=22
View Solution