Question

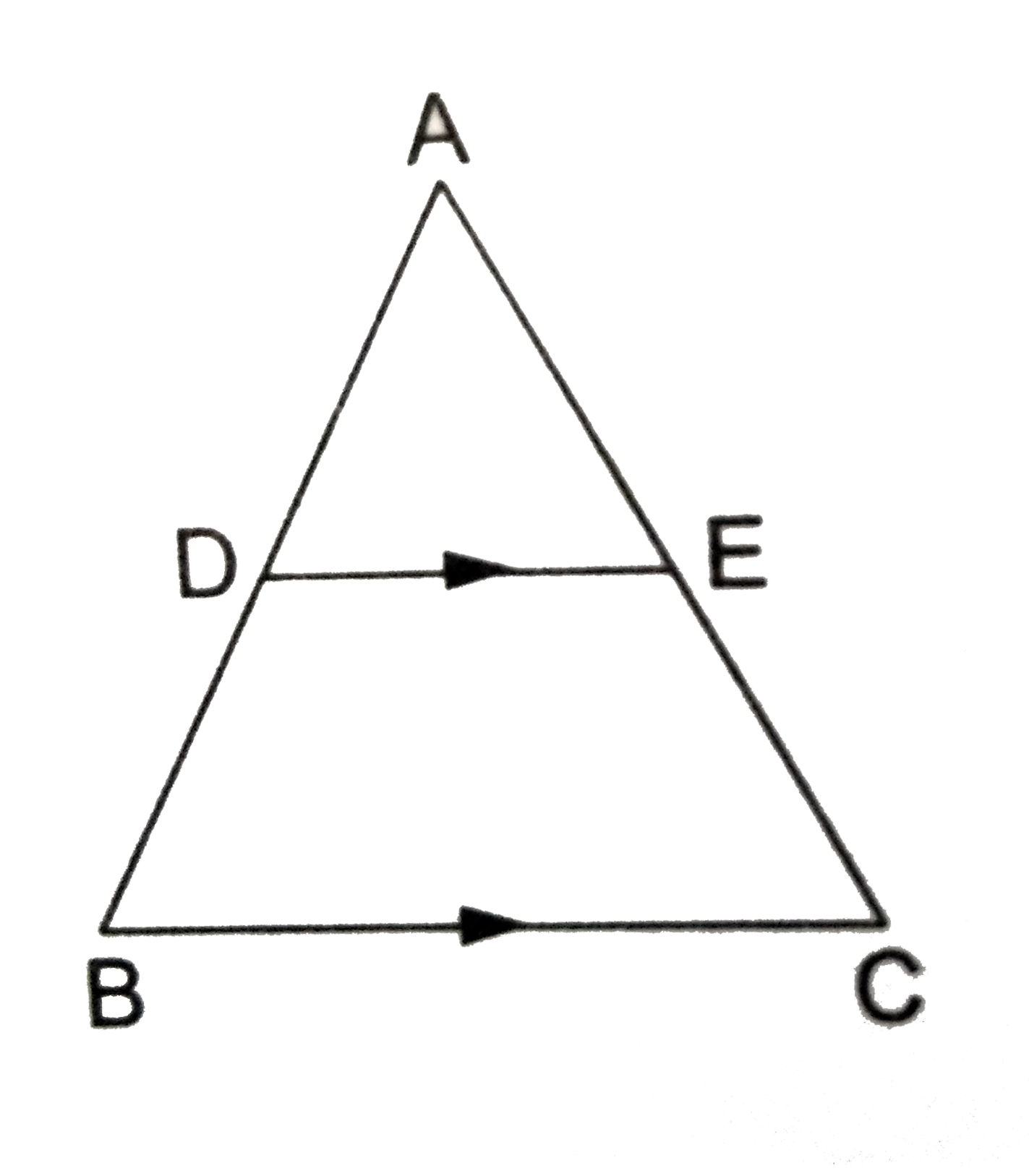
In $\Delta ABC,DE\left|\right|BC$. If AD= 2.4 cm, AE= 3.2 cm, DE= 2 cm and BC= 5 cm, find BD and CE.

Answer

BD=3.6 cm,CE=4.8 cm

Explanation

To solve the problem, we will use the properties of similar triangles since DE is parallel to BC. Let's break down the solution step by step.

Step 1: Understand the given information
We have triangle ABC with DE parallel to BC. The lengths provided are:
- AD = 2.4 cm
- AE = 3.2 cm
- DE = 2 cm
- BC = 5 cm

We need to find the lengths of BD and CE.

Step 2: Set up the proportion using similar triangles
Since DE is parallel to BC, triangles ADE and ABC are similar. Therefore, we can set up the following proportion:

\( \frac{AD}{AB} = \frac{DE}{BC} \)

Let BD = x and CE = y. Then, we can express AB and AC in terms of x and y:
- AB = AD + BD = 2.4 + x
- AC = AE + CE = 3.2 + y

Step 3: Substitute the known values into the proportion
Substituting the known values into the proportion gives us:

\( \frac{2.4}{2.4 + x} = \frac{2}{5} \)

Step 4: Cross-multiply to solve for x
Cross-multiplying yields:

\( 2.4 \cdot 5 = 2 \cdot (2.4 + x) \)

This simplifies to:

\( 12 = 4.8 + 2x \)

Step 5: Solve for x
Now, isolate x:

\( 12 - 4.8 = 2x \)
\( 7.2 = 2x \)
\( x = \frac{7.2}{2} = 3.6 \text{ cm} \)

So, BD = 3.6 cm.

Step 6: Find CE using another proportion
Now, we can use the other proportion to find CE:

\( \frac{AE}{AC} = \frac{DE}{BC} \)

Substituting the known values gives us:

\( \frac{3.2}{3.2 + y} = \frac{2}{5} \)

Step 7: Cross-multiply to solve for y
Cross-multiplying yields:

\( 3.2 \cdot 5 = 2 \cdot (3.2 + y) \)

This simplifies to:

\( 16 = 6.4 + 2y \)

Step 8: Solve for y
Now, isolate y:

\( 16 - 6.4 = 2y \)
\( 9.6 = 2y \)
\( y = \frac{9.6}{2} = 4.8 \text{ cm} \)

So, CE = 4.8 cm.

Final Answer
Thus, the lengths are:
- BD = 3.6 cm
- CE = 4.8 cm

---