A radioactive material of half-life T was kept in a nuclear reactor at two different instants. The quantity kept second time was twice of the kept first time. If now their present activities are A1 and A2 respectively, then their age difference equals
T1n2(1n2A1A2)
T(1nA1A2)
T1n2(1nA22A1)
T(1nA22A1)
The correct Answer is:A
Here in this case, A1=λN0e−λt1
or t1=1λ1n(λN0A1) ...(i)
Again, A2=(λ)(2N0)e−λt2
∴ t2=1λ1n(2λN0A2) ...(ii)
From eqns. (i) and (ii),
t2−t1=1λ1n(2A1A2)=T1n(2)1n(2A1A2)[∵1λ=T1n2]
Topper's Solved these Questions
- NUCLEAR PHYSICBOOK - MTG-WBJEECHAPTER - NUCLEAR PHYSICEXERCISE - WB JEE WORKOUT (CATEGORY 3: One or More than One Option Correct Type )
10 Videos
- NUCLEAR PHYSICBOOK - MTG-WBJEECHAPTER - NUCLEAR PHYSICEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS ( CATEGORY 1 : Single Option Correct Type )
1 Video
- NUCLEAR PHYSICBOOK - MTG-WBJEECHAPTER - NUCLEAR PHYSICEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS
5 Videos
- MOTION OF CENTRE OF MASS, CONNECTED SYSTEMS, FRICTIONBOOK - MTG-WBJEECHAPTER - MOTION OF CENTRE OF MASS, CONNECTED SYSTEMS, FRICTIONEXERCISE - WB-JEE Previous years questions
17 Videos
- OSCILLATIONS AND WAVESBOOK - MTG-WBJEECHAPTER - OSCILLATIONS AND WAVESEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (CATEGORY 3: ONE OR MORE THAN ONE OPTION CORRECT TYPE (2 MARKS))
2 Videos
Similar Questions
A radioactive substance has half-life T12 and number of radioactive nuclides present initially was N0. What is the number of radioactive nuclides at time t ?
View SolutionIn a radioactive decay processthe activity is defined as A = - (dN)/dtwhereN(t)istheνmberofradioactiveνc≤iattimet.Tworadioactivesources,S_1andS_2havesameactivityattimet=0.Atalatertime,theactivitiesofS_1andS_2areA_1andA_2,respectively.WhenS_1andS_2havejustcomp≤tedtheir3rdand7thhalf−lives,respectively,theratioA_1/A_2 is.
View Solution
Knowledge Check
Two electrons are moving with the same speed, v One electron enters at different instants , the quantity produced second time was twic of that produced first time. If now their present acitivies are A1 and A2 respectively then their age difference equals
Aλ1=λ2Bλ1>λ2Cλ1<λ2Dλ1>λ2 or λ1<λ2A radioactive nucleus can decay by two different processes. The half lives of the first and second decay processes are 5×103 and 105 years respectively. Then the effective half -life of the nucleus is
A105×105 yearsB4762 yearsC104 yearsD47.6 yrsThe decay constant of a radioactive isotope is λ. If A1 and A2 are its activities at time t1 and t2 respectively, then the number of nuclei which have decayed the time (t1−t2)
AA1t1−A2t2BA1−A2C(A1−A2)/λDλ(A1−A2)
Two different radioactive elements with half lives T1andT2 have N1andN2 (undecayed) atoms respectively present at a given instant. Determine the ratio of their activities at this instant.
View SolutionThe uranium disintegrates at a rate proportional to the amount present at any instant. If m1andm2 gms of uranium are present at time t1andt2 respectively, then half life of uranium is
View SolutionTwo radioactive samples of different elements (half-lives t1 and t2 respectively) have same number of nuclei at t=0. The time after which their activities are same is
View SolutionThe count rate of 10g of radioactive material was measured at different times and times has been shown in the figure. The half-life of material and the total counts (approximately) in the first half life period, respectively are.
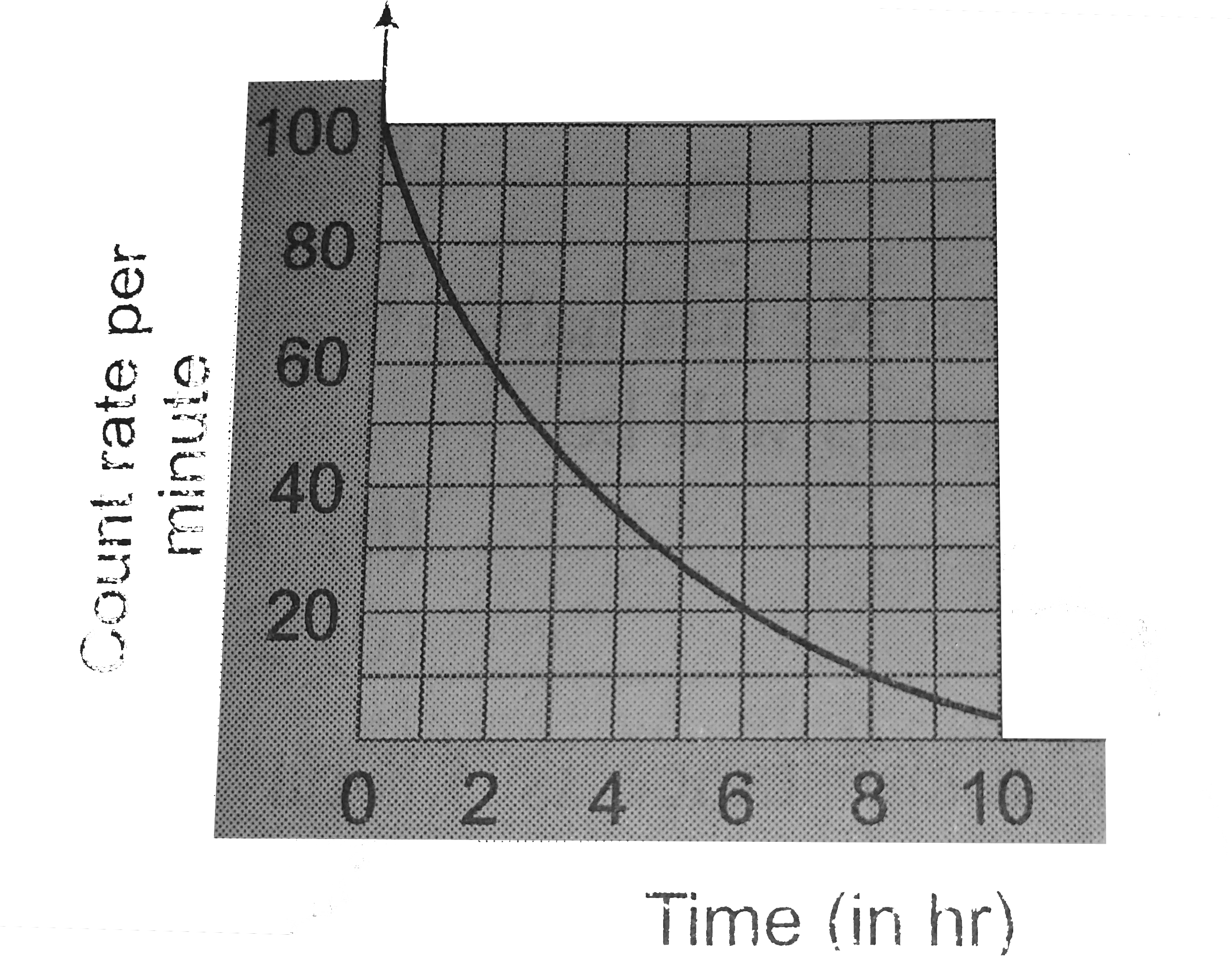 .
.
View SolutionA radioactive nucleus can decay by two different processes . The half life for the first process is t1 and that for the second process is t2. If effective half life is t, then
View Solution
