Three cells of emf, ε1=1.5V,ε2=2.0Vandε3=3.0V having internal resistance r1=0.30Ω,r2=0.4Ωandr3=0.6Ω respectively are connected in parallel. Find out the equivalent emf and the equivalent resistance of a cell which can replace this combination.
To solve the problem of finding the equivalent emf and the equivalent resistance of three cells connected in parallel, we will follow these steps:
Step 1: Write down the given values
We have three cells with the following parameters:
- Cell 1: ϵ1=1.5V, r1=0.30Ω
- Cell 2: ϵ2=2.0V, r2=0.40Ω
- Cell 3: ϵ3=3.0V, r3=0.60Ω
Step 2: Calculate the equivalent emf (ϵnet)
The formula for the equivalent emf of cells in parallel is given by:
ϵnet=ϵ1/r1+ϵ2/r2+ϵ3/r31/r1+1/r2+1/r3
Substituting the values:
ϵnet=(1.5/0.30)+(2.0/0.40)+(3.0/0.60)(1/0.30)+(1/0.40)+(1/0.60)
Calculating the numerator:
1.50.30=5,2.00.40=5,3.00.60=5
Numerator=5+5+5=15
Calculating the denominator:
10.30=103,10.40=52,10.60=53
Finding a common denominator (which is 6):
103=206,52=156,53=106
Denominator=20+15+106=456
Now substituting back into the formula:
ϵnet=15456=15×645=2V
Step 3: Calculate the equivalent resistance (Req)
The equivalent resistance for resistors in parallel is calculated using:
1Req=1r1+1r2+1r3
Substituting the values:
1Req=10.30+10.40+10.60
Calculating each term:
10.30=103,10.40=52,10.60=53
Finding a common denominator (which is 6):
103=206,52=156,53=106
1Req=20+15+106=456
Thus,
Req=645=215Ω
Final Results
- Equivalent emf (ϵnet) = 2 V
- Equivalent resistance (Req) = 215Ω
We have three cells with the following parameters:
- Cell 1: ϵ1=1.5V, r1=0.30Ω
- Cell 2: ϵ2=2.0V, r2=0.40Ω
- Cell 3: ϵ3=3.0V, r3=0.60Ω
The formula for the equivalent emf of cells in parallel is given by:
Numerator=5+5+5=15
Denominator=20+15+106=456
The equivalent resistance for resistors in parallel is calculated using:
1Req=20+15+106=456
- Equivalent emf (ϵnet) = 2 V
- Equivalent resistance (Req) = 215Ω
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - Objective Type Questions (MULTIPLE CHOICE QUESTIONS) (A. MULTIPLE CHOICE QUESTIONS WITH ONLY ONE CORRECT ANSWER)
36 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - Objective Type Questions (MULTIPLE CHOICE QUESTIONS) (B. MULTIPLE CHOICE QUESTION FOR COMPETITIVE EXAMINATIONS)
20 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - REVISION EXERCISES (LONG ANSWER QUESTIONS)
10 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
Three cells of emfs ɛ1=1.5V,ɛ2=2.0vandɛ3=3.0V having internal resistances r1=0.3Ωr2=0.4Ωandr3=0.6Ω respectively are connected in parallel . Find out the equivalent emf and the equivalent resistance of a cell which can replace this combination.
View SolutionTwo cells of emfs 1.5 V and 2.0 V having internal resistances 0.2Ω and 0.3Ω respectively are connected in parallel. Calculate the emf and internal resistance of the equivalent cell.
View Solution
Knowledge Check
Two resistor of resistance R1=(6±0.09)Ω and R2=(3±0.09)Ω are connected in parallel the equivalent resistance R with error (in Ω)
AR=(2±0.04)BR=(2±0.05)CR=(9±0.18)DR=(2±0.18)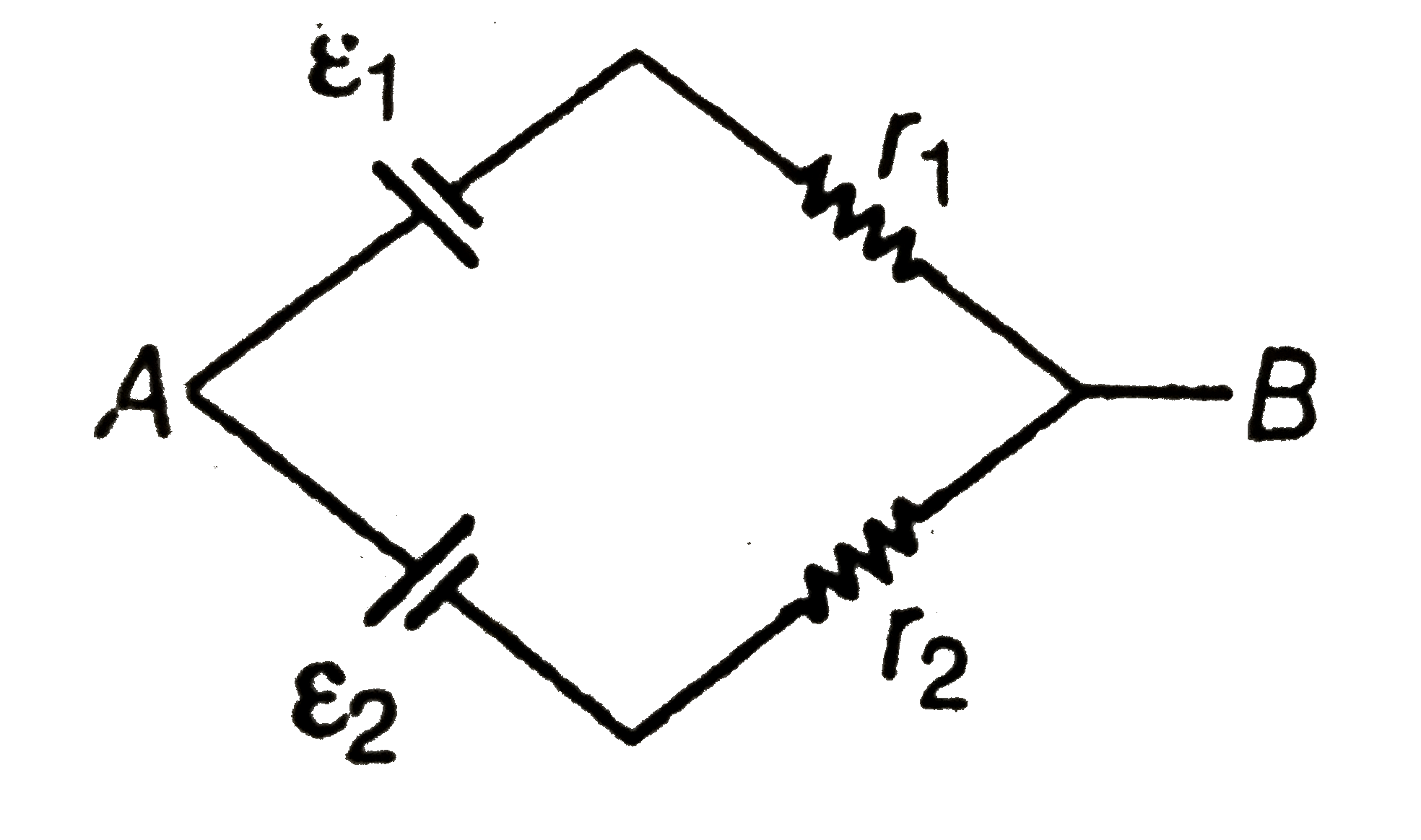
Two batteries of emf ε1andε2(ε2>ε1) and internal resistance r1andr2 respectively are connected in parallel as shown in figure.AThe equivalent emf εeq of the two cells is between ε1andε2, i.e., ε1<εeq<ε2Bthe equivalent emf εeq is smaller than ε1CThe εeq is given by εeq=ε1+ε2 always.Dεeq is independent of internal resistance r1andr2.
Two cells of emfs 1.5 V and 2.0v having internal resistance 0.2Σ and 0.3 Σ respectively are connected in parallel . Calculate the emf and internal resistance of the equivalent cell .
View SolutionTwo cells of emf ε1 and ε2 having internal resistances r1 and r2 respectively are connected in parallel as shown in Fig. Deduce the expressions for the equivalent emf and equivalent internal resistance of a cell which can replace the combination between the pointsB1 and B2 .

View Solution(a) Two cells of emf ε1 and ε2 having internal resistances r1 andr2 respectively are connected in parallel as shown in Fig. Deduce the expressions for the equivalent emf and equivalent internal resistance of a cell which can replace the combination between the points B1 and B2 . (b) Calculate the current shown by the ammeter in the circuit diagram given below
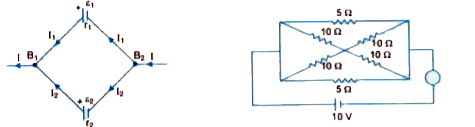
View SolutionTwo cells of emfs 1.5 V and 2.0v having internal resistance 0.2Σ and 0.3 Σ respectively are connected in parallel . Calculate the emf and internal resistance of the equivalent cell .
View SolutionTwo resistances R1=100±3ΩandR2=200±4Ω are connected in series . Find the equivalent resistance of the series combination.
View SolutionTwo cells of emf 2ε and ε and internal resistance 2r and r respectively, are connected in parallel. Obtain the expressions for the equivalent emf and the internal resistance of the combination.
View Solution
