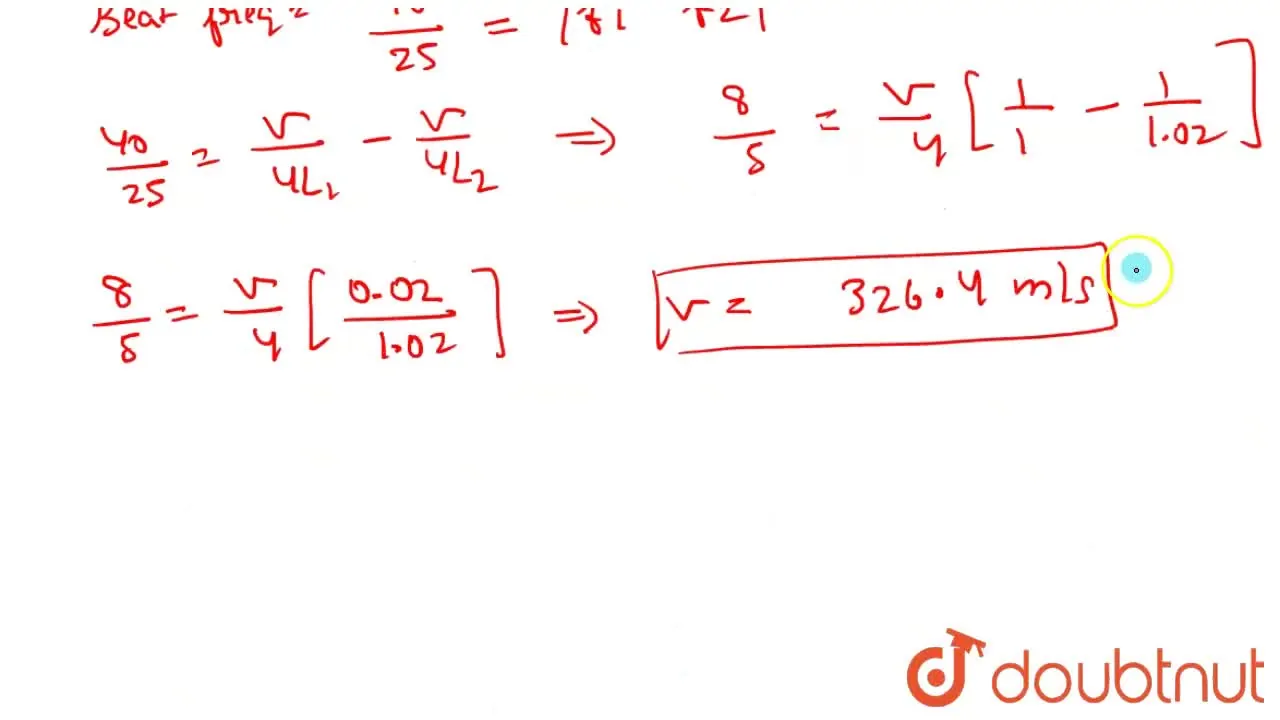
Calculate the velocity of sound when two resonance tubes 100 cm and 102 cm give 40 beats in 25 seconds sounding with fundametnal mode.
L1 = 100 cm, L2 = 102 cm
= 1m, L2 = 1.02 m
Beat frequency =v1−v2
4025=V4L1−V4L2
=V4(1L1−1L2)
4025=V4(11−11.02)
4025=V4×0.021.02
4025×4×1.020.02=V
V = 326.4 m/s
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - MULTIPLE CHOICE QUESTIONS88videos
WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - ASSERTION REASON TYPE QUESTIONS11videos
WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - HIGH ORDER THINKING SKILLS & ADVANCED LEVEL15videos
WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST15videos
UNITS AND MEASUREMENT
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Chapter Practice Test16videos
WORK, ENERGY AND POWER
Similar Questions
Two air columns of resonance appatus, 100cm and 101 cm long give 17 beats in 20 second, when each is sounding its fundamental mode. Calculate the velocity of sound.
View SolutionTwo air columns of resonance tubes of lengths 110 cm and 112 cm give 25 beats in 20 seconds. If each air column is sounding its fundamental mode, calculate the velocity of sound.
View SolutionCalculate the velocity of sound in a gas in which two sound waves of length 100cm, and 101 cm. produced 24 beats in 6 seconds.
View SolutionCalculate the velocity of sound in a gas in which two waves of wavelength 50cm and 50.5cm produce 6 beats per second.
View SolutionTwo sources of sound are in resonance when
View SolutionCalculate the speed of sound in a gas in two sound waves of wavelenths 1.00 m and 1.01 m that produce 24 beats in 6 seconds.
View SolutionTwo sound waves of wavelengths 5 m and 6 m formed 30 beats in 3 seconds. The velocity of sound is
View SolutionCalculate the speed of sound in a gas in which two waves of wavelenghts 50cmand50.5cm produce 6beats/s.
View SolutionTwo closed organ pipes of length 100 cm and 101 cm product 16 beats is 20 sec, When each pipe is sounded in its fundamental mode calculate the velocity of sound .
View SolutionTwo waves of wavelengths 99 cm and 100 cm produce 4 beats per second. Velocity of sound in the medium is
View Solution