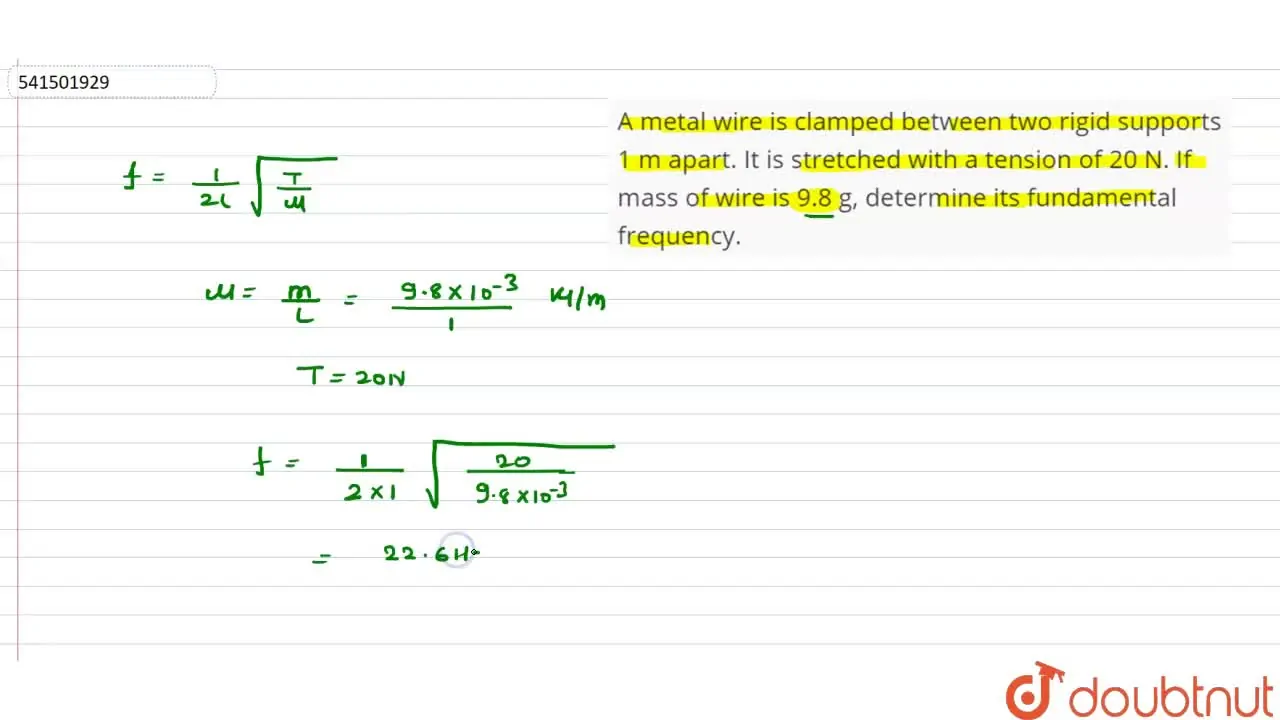
A metal wire is clamped between two rigid supports 1 m apart. It is stretched with a tension of 20 N. If mass of wire is 9.8 g, determine its fundamental frequency.
Given, L = 1 m
T = 20 N
Mass per unit length, m=9.8g1m=9.8g/m
=9.8×10−3kg/m
∴ Fundamental frequency, v=12L√Tm
=12×1√209.8×10−3=22.6 Hz
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - PROBLEM9videos
WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - PRACTICE PROBLEMS38videos
WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST15videos
UNITS AND MEASUREMENT
- BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Chapter Practice Test16videos
WORK, ENERGY AND POWER
Similar Questions
The successive resonating frequencies for a stretched wire are 250 Hz and 300 Hz. The wire is stretched between two rigid supports with a tension of 36 N.
If the mass per unit length of the wire is 0.01 kg, then the length of the wire isView SolutionA wire is stretched between two rigid supports vibrates in its fundamental mode with a frequency of 50 Hz. The mass of the wire is 30 g and its linear density is 4×10−2 kg m s−1. The speed of the transverse wave at the string is
View SolutionA wire stretched between two rigid supports vibrates in its fundamental mode with a frequency of 45Hz. The mass of the wire is 3.5×10−2 kg and its linear density is 4×10−2 kg/m . What is the speed of transverse wave on string and tension in the wire?
View SolutionA wire stretched between two rigid supports vibrates in its fundamental mode with a frequency of 45Hz. The mass of the wire is 3.5×10−2kg and its linear mass density is 4.0×10−2kgm−1. What is (a) the speed of a transverse wave on the string , and (b) the tension in the string?
View SolutionA wire stretched between two rigid supports vibrates in its fundamental mode with a frequency of 45Hz. The mass of the wire is 3.5×10−2kg and its linear mass density is 4.0×10−2kgm−1. What is (a) the speed of a transverse wave on the string , and (b) the tension in the string?
View SolutionA wire stretched between two rigid supports vibrates in its fundamental mode with a frequency of 45 Hz. The mass of the wire is 3.5×10−2kg and its linear mass density is 4.0×10−2kgm−1. What is the speed of a transverse wave on the wire?
View SolutionA sonometer wire of density ρ and radius r is held between two bridges at a distance L apart . Tension in the wire is T. then the fundamental frequency of the wire will be
View SolutionA sonometer wire is under a tension of 40 N and the length between the bridges is 50 cm. A metre long wire of the sonometer has a mass of 1.0 g. Determine its fundamental frequency.
View SolutionA wire having a linear density of 0.05g/cmis stretched between two rigid supports with a tension of 450N. It is observed that the wire resonates at a frequency of 420 Hz. The next higher frequency at which the same wire resonates is 490 Hz. Find the length of the wire.
View SolutionA steel piano wire 0.5 m long has a total mass of 0.01 kg and is stretched with a tension of 800 N. The frequency, when it vibrates in its fundamental mode, is
View Solution