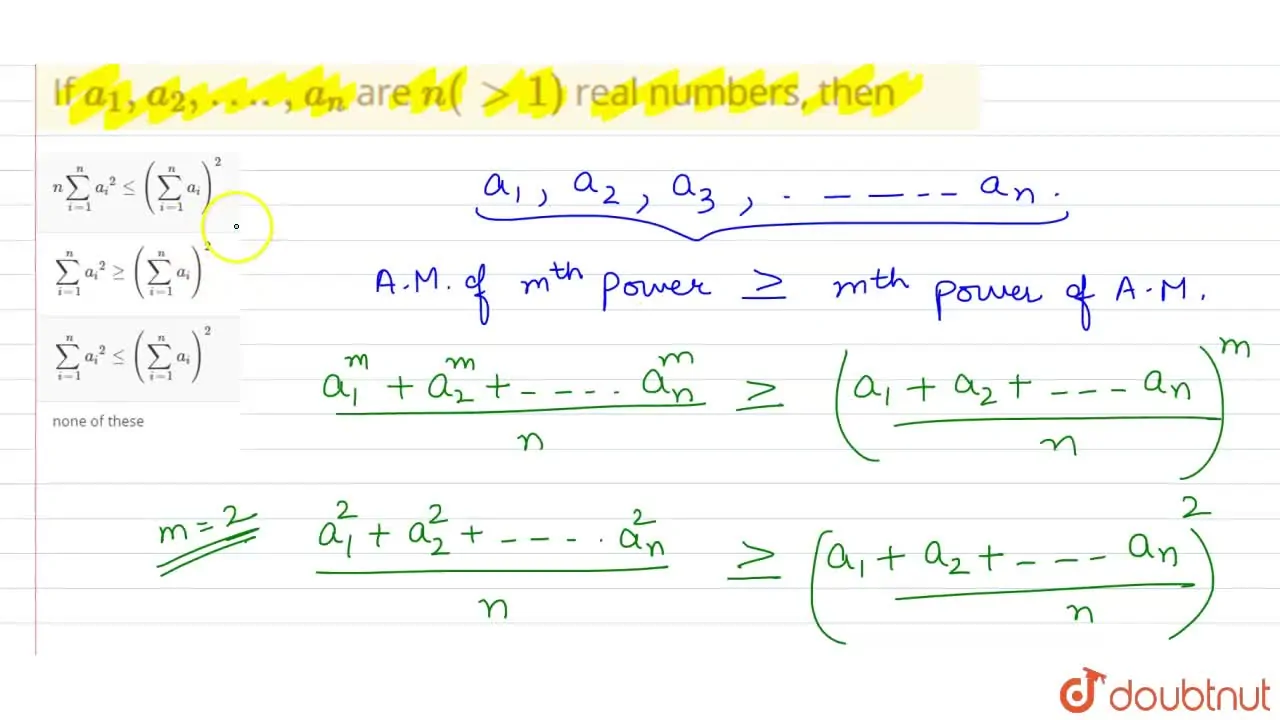
If $a_{1}, a_{2}, \dots ., a_{n}$ are $n (> 1)$ real numbers, then

$n n \sum i = 1 a_{i}^{2} \leq {(n \sum i = 1 a_{i})}_{i}^{2}$

$n \sum i = 1 a_{i}^{2} \geq {(n \sum i = 1 a_{i})}_{i}^{2}$

$n \sum i = 1 a_{i}^{2} \leq {(n \sum i = 1 a_{i})}_{i}^{2}$

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Updated on:21/07/2023

If $a - 1, a_{2},, a_{n}$ are positive real numbers whose product is a fixed number $c,$ then the minimum value of $a_{1} + a_{2} + + a_{n - 1} + 2 a_{n}$ is $a_{n - 1} + 2 a_{n}$ is b. $(n + 1) c^{1 / n}$ $2 n c^{1 / n}$ $(n + 1) {(2 c)}^{1 / n}$
A $n {(2 c)}^{1 / n}$
B $(n + 1) c^{1 / n}$
C $2 n c^{1 / n}$
D $(n + 1) {(2 c)}^{1 / n}$
If $a_{1}, a_{2}, a_{3}, . . ., a_{n}$ are non-zero real numbers such that
$(a_{1}^{2} + a_{2}^{2} + . . . + a_{n - 1} .^{2}) (a_{2}^{2} + a_{3}^{2} + . . . + a_{n}^{2}) \leq {(a_{1} a_{2} + a_{2} a_{3} + . . . + a_{n - 1} a_{n})}_{1}^{2} then, a_{1}, a_{2}, . . . . a_{n}$ are in
AA.P.
BG.P.
CH.P.
Dnone of these
If $a_{1}, a_{2}, ..., a_{n}$ are real numbers with $a_{n} \neq 0$ and $cos α + i sin α$ is a root of $z^{n} + a_{1} z^{n - 1} + a_{2} z^{n - 2} + ... + a_{n - 1} z + a_{n} = 0$ then the sum $a_{1} cos α + a_{2} cos 2 α + a_{3} cos 3 α + ... + a_{n} cos n α$ is
A0
B1
C $- 1$
D $\frac{1}{2}$