Let f:(−1,1)→B be a function defined by f(x)=tan−1(2x)1−x2 . Then f is both one-one and onto when B is the interval. [0,π2) (b) (0,π2) (−π2,π2) (d) [−π2,π2]
(−π/2,π/2)
[−π/2,π/2]
[0,π/2]
(0,π/2)
The correct Answer is:A
We have
f(x)=tan−1(2x1−x2)=2tan−1x,ifx∈(−1,1)
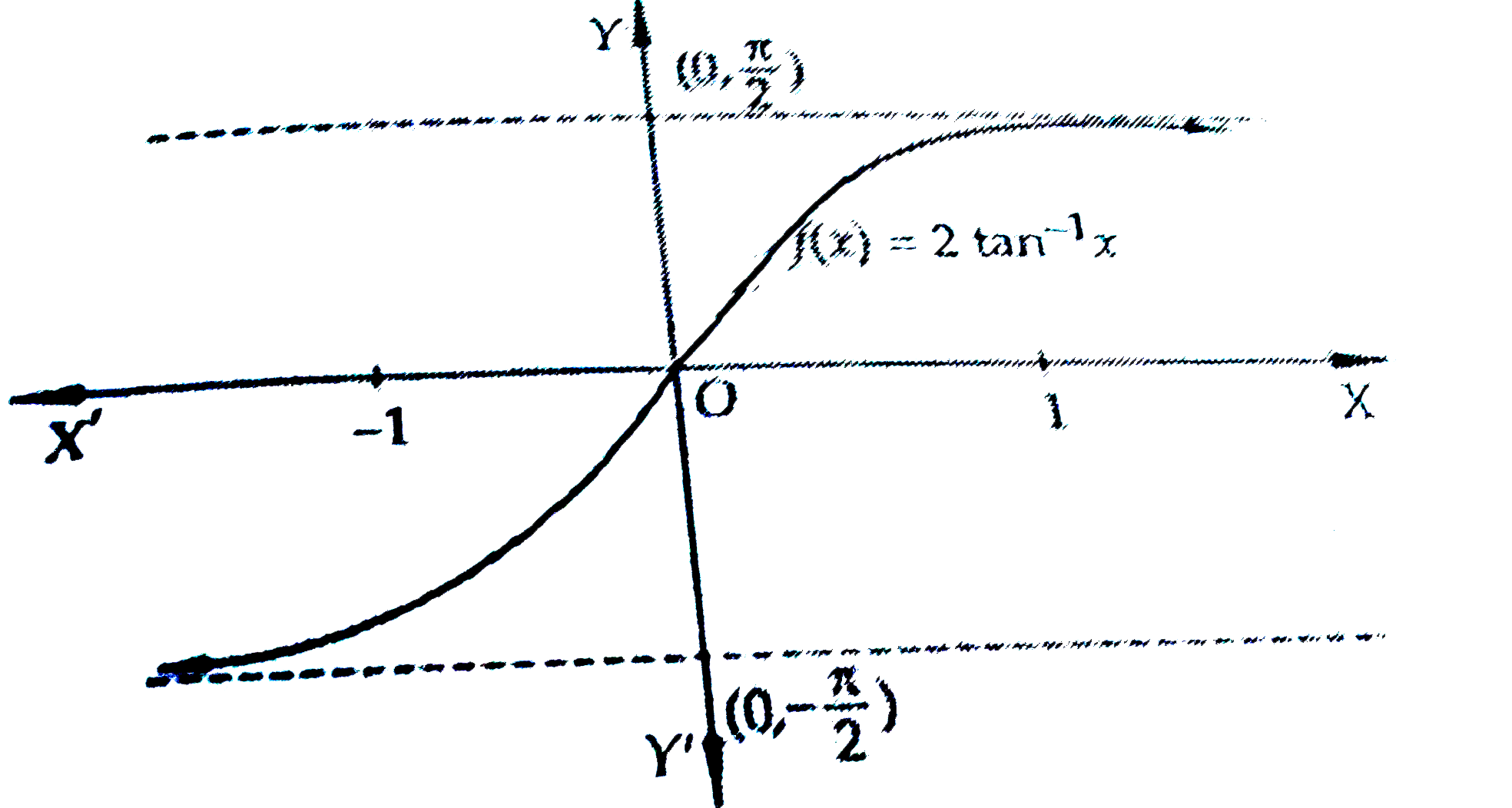
Since, f(x) is both one-one both and onto. Therefore,
B=Range (f)=(f(−1)),f(1))=(−π/2,π/2)
Topper's Solved these Questions
- FUNCTIONSBook:OBJECTIVE RD SHARMAChapter:FUNCTIONSExercise:Section II - Assertion Reason Type
Explore 10 Videos
- FUNCTIONSBook:OBJECTIVE RD SHARMAChapter:FUNCTIONSExercise:Exercise
Explore 48 Videos
- FUNCTIONSBook:OBJECTIVE RD SHARMAChapter:FUNCTIONSExercise:Chapter Test
Explore 30 Videos
- DISCRETE PROBABILITY DISTRIBUTIONSBook:OBJECTIVE RD SHARMAChapter:DISCRETE PROBABILITY DISTRIBUTIONSExercise:Exercise
Explore 40 Videos
- HYPERBOLABook:OBJECTIVE RD SHARMAChapter:HYPERBOLAExercise:Chapter Test
Explore 29 Videos
Similar Questions
Let f:(−1,1)→B be a function defined by f(x)=tan−1(2x1−x2) . Then f is both one-one and onto when B is the interval
View SolutionLet f:(−1,1)→B be a function defined by f(x)=tan−1[2x1−x2] . Then f is both one-one and onto when B is the interval. (a)[0,π2) (b) (0,π2) (c)(−π2,π2) (d) [−π2,π2]
View Solution
Knowledge Check
If f:(−1,1)→B be a function defined by f(x)=tan−1(2x1−x2) , then f is both one-one and onto when B is the interval :
A[−π2,π2]B(−π2,π2)C[0,π2]D[0,π2)Let f:R→B, be a function defined f(x)=tan−1.2x√3(1+x2), then f is both one - one and onto when B, is the interval
A(0,x6)B[0,π6)C[−π6,π6]D(−π6,π6)Let f:R→[0,π2) be a function defined by f(x)=tan−1(x2+x+a). If f is onto, then a is equal to
A0B1C1/2D1/4
If y=tan−1x+tan−1(1x)+sec−1x, then y lies in the interval (a)[π2,π)∪[π,3π2) (b) [π2,3π2] (c)(0,π) (d) [0,π2)∪[π2,π)
View SolutionOne of the root equation cosx−x+12=0 lies in the interval (0,π2) (b) (−π2,0) (c) (π2,π) (d) (π,3π2)
View SolutionShow that f(x)=tan−1(sinx+cosx) is a decreasing function on the interval on (π/4, π/2) .
View SolutionIf f(x)=12x−1, then on the interval [0,π]
View SolutionIf f(x)=12x−1, then on the interval [0,π]
View Solution
