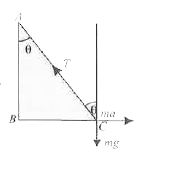
A plumb line gets inclined to vertical at angle θ when the carriage travels with an acceleration a. The plumb line is suspended from the roof of the carriage. The acceleration a is given by
a=gtanθ
a=gsinθ
a=gcosθ
a=gsecθ
The correct Answer is:A
Resolve tension in the plumb line into two rectangular components and equate the forces.
ma = Tsinθ
mg=Tcosθ
∴ag=tanθ⇒a=gtanθ
Topper's Solved these Questions
- LAWS OF MOTION BOOK - MTG-WBJEECHAPTER - LAWS OF MOTION EXERCISE - WB JEE WORKOUT (CATEGORY 2: SINGLE OPTION CORRECT TYPE (2 MARKS))
15 Videos
- LAWS OF MOTION BOOK - MTG-WBJEECHAPTER - LAWS OF MOTION EXERCISE - WB JEE WORKOUT (CATEGORY 3: ONE OR MORE THAN ONE OPTION CORRECT TYPE (2 MARKS))
10 Videos
- KINETIC THEORY OF GASES BOOK - MTG-WBJEECHAPTER - KINETIC THEORY OF GASES EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (MCQ)
7 Videos
- MAGNETIC EFFECT OF CURRENTBOOK - MTG-WBJEECHAPTER - MAGNETIC EFFECT OF CURRENTEXERCISE - WB JEE Previous Years Questions
22 Videos
Similar Questions
A car is moving on a plane inclined at 30∘ to the horizontal with an acceleration of 10m/s2 parallel to the plane upward. A bob is suspended by a string from the roof. The angle in degrees which the string makes with the vertical is: (Assume that the bob does not move relative to car) [g=10m/s2]
View SolutionA projectile is fired from horizontal at an angle θ . What are the horizontal and vertical acceleration at the highest point ?
View Solution
Knowledge Check
A simple pendulum with a bob of mass m is suspended from the roof of a car moving with horizontal acceleration a
AThe string makes an angle of tan−1(a/g) with the verticalBThe string makes an angle of sin−1(ag) with the verticalCThe tension in the string is m√a2+g2DThe tension in the string is m√g2−a2A trolley moves in horizontal direction with an acceleration a. A simple pendulum of length l is suspended from the roof of the trolley. The time period of the pendulum will be
AinfinityBzeroC2π√l(g+a)D2π√l√a2+g2A simple pendulum with a bob of mass m is suspended from the roof of a car moving with a horizontal acceleration a.
AThe string makes an angle of tan−1(a/g) with the verticalBThe string makes an angle of tan−1(1−ag) with the verticalCThe tension in the string is m√a2+g2DThe tension in the string is m√g2−a2
A train travelling on one of two intersecting railway lines, subtends at a certain station on the other line, an/ angle α when the front of the carriage reaches the junction and an angle β when the end of the carriage reaches it. Then, the two lines are inclined to each other at an angle theta, show that 2cotθ=cotα−cotβcotα+cotβ
View SolutionA train travelling on one of two intersecting railway lines, subtends at a certain station on the other line, an angle α when the front of the carriage reaches the junction, and an angle β when the end of the carriage reaches it. Prove that the two lines are inclined to each other at an angle θ determined by 2cotθ=cotα−cotβ.
View SolutionWhen a body is placed on a rough plane inclined at an angle θ to the horizontal, its acceleration is
View SolutionA car is moving in a circular horizontal track of radius 10 m with a constant speed of 10ms−1. A plumb bob is suspended from the roof of the car by a string of length 1m. The angle made by the string with vertical is (g=10ms−2)
View SolutionA bus is moving in a circular horizontal track of radius 10 m with a constant speed 10m/s. A plumb bob is suspended from the roof of length 1.0m. The angle made by the rod with the track is (take g=10m/s2)
View Solution
