ΔABD is a right triangle, right-angled at A and AC⊥BD. Prove that AB2=BC×BD.
We know that:
If a perpendicular is drawn from the vertex of the right angle of triangle to the hypotenuse then the triangles on both sides hypotenuse then the triangles on both sides of the perpendicular are similar to the whole triangle and also to each other.
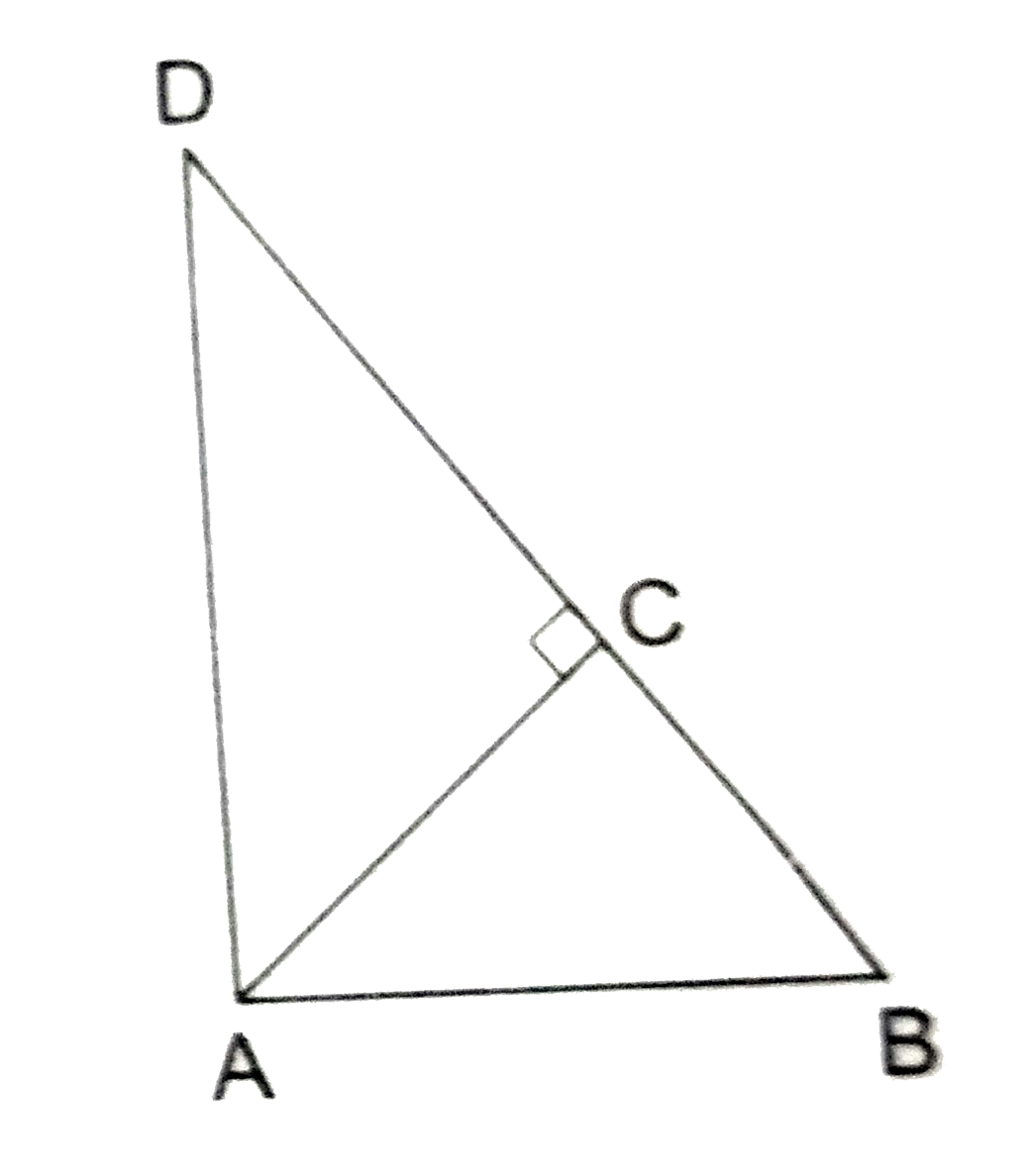
∴ΔABC~ΔDBA
ΔABC~ΔDAC
ΔDBA~ΔDAC.
(i)ΔABC~ΔDBA
⇒ABDB=BCBA⇒ABBD=BCAB⇒BC⋅BD.
(ii) ΔABC~ΔDAC
⇒ACDC=BCAC⇒AC2=BC⋅DC.
(iii)ΔDBA~ΔDAC
⇒ADCD=BDAD⇒AD2=BD⋅CD
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLE
4 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A
1 Video
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
ΔABD is a right triangle, right-angled at A and AC⊥BD. Prove that AB2=BC×BD.
View SolutionIn the figure given below ABC is a triangle right angled at A and AC ⊥ BD . Show that
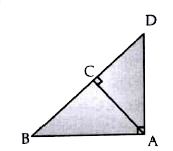
AB2=BC×BD
View SolutionABC is a right triangle right angled at C and AC = √3BC . Prove that ∠ABC=60∘ .
View SolutionABC is a right triangle right-angled at CandAC=√3BC . Prove that ∠ABC=600.
View SolutionABC is an isosceles right triangle, right-angled at C . Prove that: AB2=2AC2.
View SolutionABC is an isosceles right triangle right-angled at C . Prove that AB2=2AC2 .
View SolutionIn the figure given below ABC is a triangle right angled at A and AC ⊥ BD . Show that
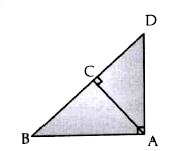
AD2=BD×DC
View SolutionIn the figure , ABD is a triangle right angled at A and AC ⊥ Bd . Show that
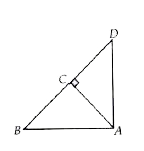
AD2=BD⋅CD
View SolutionABC is an isosceles triangle right-angled at C. Prove that AB2=2AC2.
View SolutionABC is a isosceles right angled triangle, right angled at C. prove that AB2=2AC2
View Solution