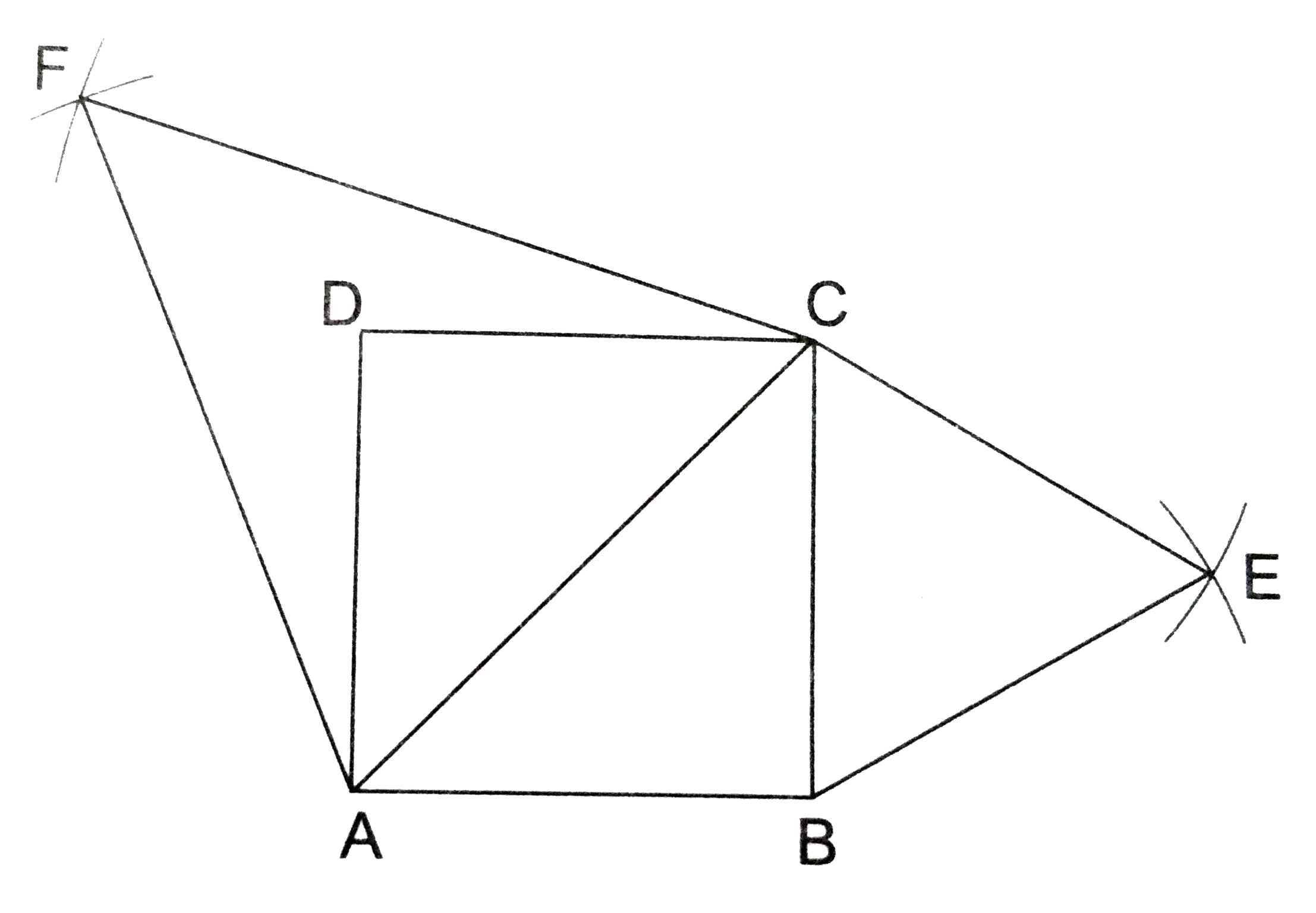
Prove that the area of an equilateral triangle described on one side of a square is equal to half the area of the equilateral triangle described on one of its diagonals.

Video Solution

Text Solution

Verified by Experts

GIVEN A squre ABCD and equilteral $Δ B C E and A C F$ have been descibed on side BC and diagonal AC respectively.
TO PROOVE $a r (Δ B C E) = \frac{1}{2} a r (Δ A C F)$ .
PROOF Since each of the $Δ B C E and Δ A C F$ is an equilateral triangle, so each angle of each one of them is $60^{\circ}$ . So, the triangles are equiangular, and hence similar.
$∴ Δ B C E ~ Δ A C E$ .
$∴ \frac{a r (Δ B C E)}{a r (Δ A C F)} = \frac{B C^{2}}{A C^{2}} = \frac{B C^{2}}{2 {(B C)}^{2}} [∴ A C = \sqrt{2} B C]$
$= \frac{1}{2}$
Hence, $a r (Δ B C E) = \frac{1}{2} \times a r (Δ A C F)$

|

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Fill in the blanks .
(i) In two similar triangles , ABC and PQR , if their corresponding altitudes AD and PS are in the ratio 4 : 9 , then $a r (Δ A B C) : a r (Δ P Q R) = ¯ ¯¯¯¯ ¯ (P)$ .
(ii) Area of an equilateral triangle described on the side of a square is $¯ ¯¯¯¯ ¯ (O)$ the area of equilateral triangle described on its diagonal .
(iii) The altitude of an equilateral triangle with side 'a' equals oberline((R ))
A $\begin{matrix} P & Q & R 16 : 81 & Twice & \frac{a}{2} \end{matrix}$
B $\begin{matrix} P & Q & R 4 : 9 & Half & 2 a \end{matrix}$
C $\begin{matrix} P & Q & R 16 : 81 & Half & \frac{\sqrt{3 a}}{2} \end{matrix}$
D $\begin{matrix} P & Q & R 4 : 9 & Twice & \frac{\sqrt{3 a}}{2} \end{matrix}$
Question 2 - Select One
What is the area of an equilateral triangle with side $2 c m ?$
A $4 \sqrt{3} c m^{2}$
B $3 \sqrt{3} c m^{2}$
C $6 c m^{2}$
D $\sqrt{3} c m^{2}$

RS AGGARWAL-TRIANGLES-Test Yourself

Home
Ask Doubt
Profile