In a $△ A B C$ P and Q are points on AB and AC respectively and PQ||BC. Prove that the median AD bisects PQ.

Video Solution

Text Solution

Verified by Experts

GIVEN A $Δ A B C$ in which P and Q are points on AB and AC respectively such that $P Q ∣ ∣ B C and A D$ is the median, cutting $P Q$ at E.
TO PROVE $P E = E Q$ .

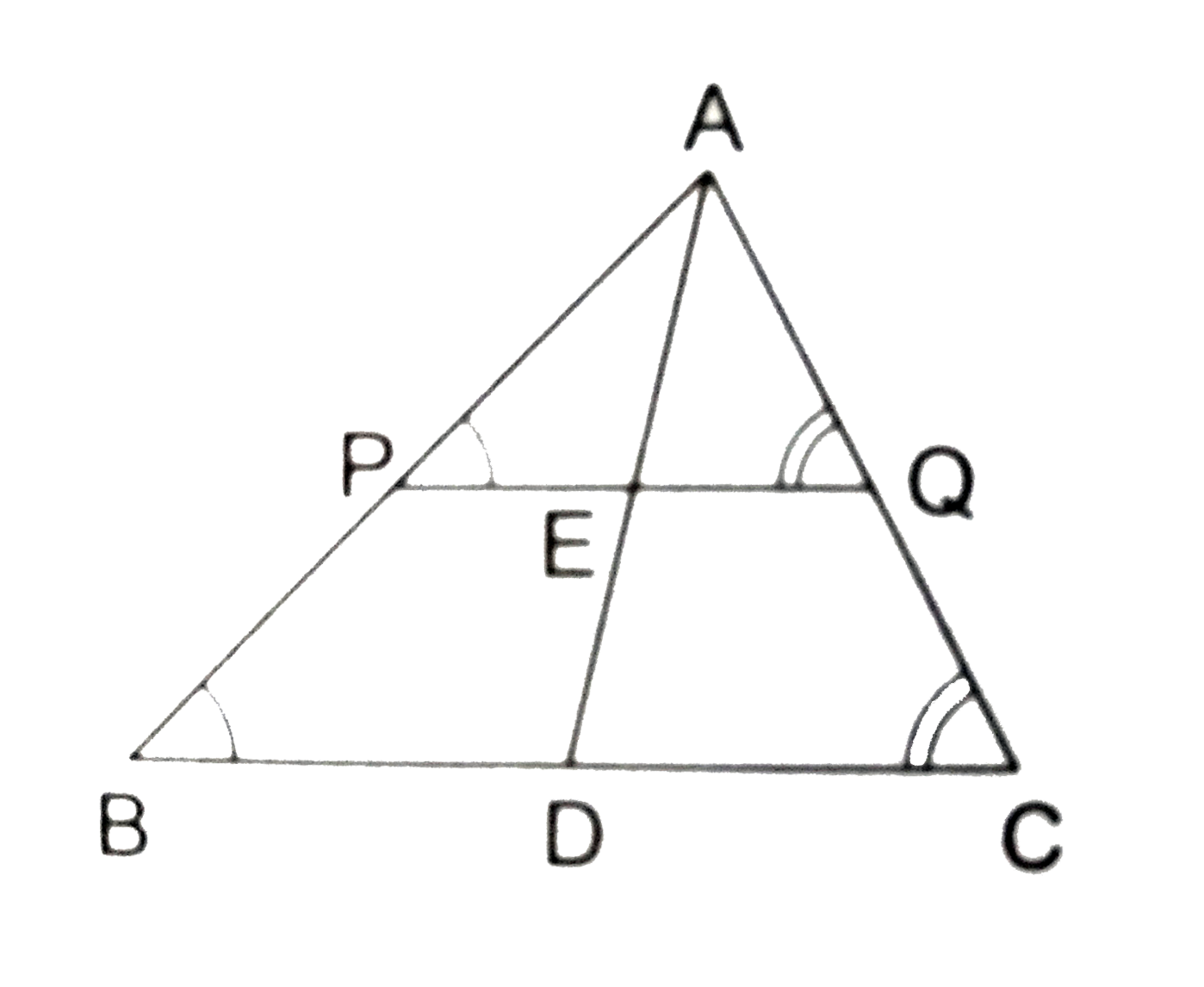
PROOF In $Δ A P E and Δ A B D$ , we have
$∠ P A E = ∠ B A D$ [ common]
$∠ A P E = ∠ A B D$ [ corrsponding $∠$ ]
$∴ Δ A P E ~ Δ A B D$ [ buy AA- similarity]
But, in similar triangles, the corresponding sides are proportional.
$∴ \frac{A E}{A D} = \frac{P E}{B D}$
In $Δ A E Q and Δ A D C$ , we have
$∠ Q A E = ∠ C A D$ [ common]
$∠ A Q E = ∠ A C D$ [ corresponding angles]
$∴ Δ A E Q ~ Δ A D C$ [ by AA- similarity]
But, in similar triangles, the correponding sides are proportional.
$∴ \frac{A E}{A D} = \frac{E Q}{D C} ... . (i i)$
From (i) and (ii), we get $\frac{P E}{B D} = \frac{E Q}{D C} [each equal to \frac{A E}{A D}]$
But, $B D = D C [∴ A D is the median]$
$∴ P E = E Q$

Updated on:21/07/2023

In a rectangle ABCD, P,Q are the mid-points of BC and AD respectively and R is any point on PQ, then $Δ A R B$ equals :
A1/2( $□ A B C D$ )
B1/3( $□ A B C D$ )
C1/4( $□ A B C D$ )
Dnone of these
In a rectangle ABCD, P,Q are the mid-points of BC and AD respectively and R is any point on PQ, then $Δ A R B$ equals :
A $\frac{1}{2} (□ A B C D)$
B $\frac{1}{3} (□ A B C D)$
C $\frac{1}{4} (□ A B C D)$
Dnone of these
ABC is an equilateral triangle. P and Q are two points on $¯ ¯¯¯¯ ¯ A B$ and $¯ ¯¯¯¯ ¯ A C$ respectively such that $¯ ¯¯¯¯ ¯ P Q ∣ ∣ ¯ ¯¯¯¯ ¯ B C$ . If $¯ ¯¯¯¯ ¯ P Q = 5 c m$ , then area of $Δ A P Q$ is :
A $\frac{25}{4}$ sq. cm
B $\frac{25}{\sqrt{3}}$ sq. cm
C $\frac{25 \sqrt{3}}{4}$ sq. cm
D $25 \sqrt{3}$ sq. cm