In triangle ABC, if AD⊥BC and AD2=BD⋅DC. Then find the angle BAC
Video Solution
Text Solution
GIVEN AΔABC in which AD⊥BCandAD2=BD⋅CD.
PROOVE ∠BAC=90∘
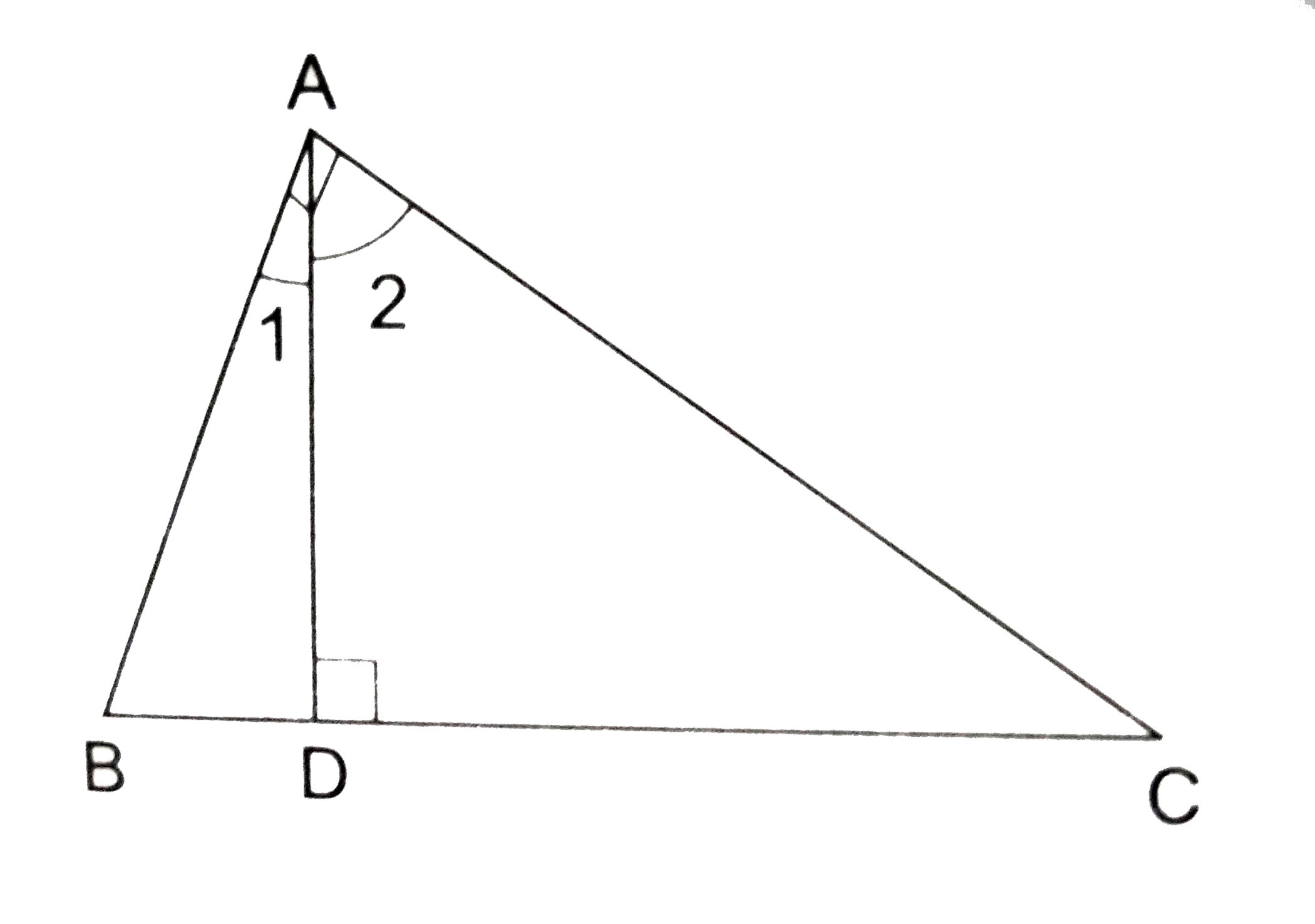
PROOF AD2=BD⋅CD⇒BDAD=ADCD
Now, in ΔDBAandΔDAC, we have
∠BDA=∠ADC=90∘andBDAD=ADCD
∴ΔDBA~ΔDAC [ by SAS- similarity]
∴∠B=∠2and∠1=∠C
∴∠1+∠2=∠B+∠C⇒∠A=∠B+∠C
⇒2∠A=∠A+∠B+∠C=180∘
⇒∠A=∠BAC=90∘
|
Topper's Solved these Questions
- TRIANGLESBook:RS AGGARWALChapter:TRIANGLESExercise:SOLVED EXAMPLE
Explore 4 Videos
- TRIANGLESBook:RS AGGARWALChapter:TRIANGLESExercise:EXERCISE 7 A
Explore 1 Video
- T-RATIOS OF SOME PARTICULAR ANGLESBook:RS AGGARWALChapter:T-RATIOS OF SOME PARTICULAR ANGLESExercise:Exercise 11
Explore 27 Videos
- TRIGONOMETRIC IDENTITIESBook:RS AGGARWALChapter:TRIGONOMETRIC IDENTITIESExercise:Test Yourself
Explore 20 Videos
Similar Questions
Knowledge Check
In ΔABC,ADI_BCandAD2=BD,DC. The measure of ∠BAC is:
A60∘B75∘C90∘D45∘A cyclic quadrailatral ABCD is such that AB=BC, AD=Dc AC⊥BD,∠CAD=θ Then the angle ∠ABC
AθBθ2C2θD3θ
In ABC , if AD⊥BC and AD2=BD×DC , prove that ∠BAC=90o .
View SolutionIn a triangle aBC ,∠B=2∠C.AD and BE bisectors of ∠BACand∠ABC. If AB=CD . Find ∠BAC
View SolutionIn Fig 2.11 AB = BC and AD = BD = DC.
The number of isoscles triangle in the figure is
View SolutionIn △ABC AB = 7 cm, BC =10cm, and AC = 8 cm. If AD is the angle bisector of ∠BAC, where D is a point on BC, then BD is equal to:
यदि त्रिभुज ABC में, AB = 7 सेमी, BC = 10 सेमी तथा AC =8 सेमी है | यदि AD कोण BAC का समद्विभाजक है, जहाँ D, BC पर स्थित एक बिंदु है, तो BD का मान किसके बराबर है ?
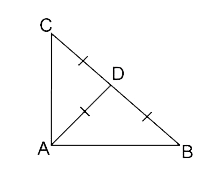
View SolutionIn the given if AD=BD=CD , Find ∠BAC
View Solution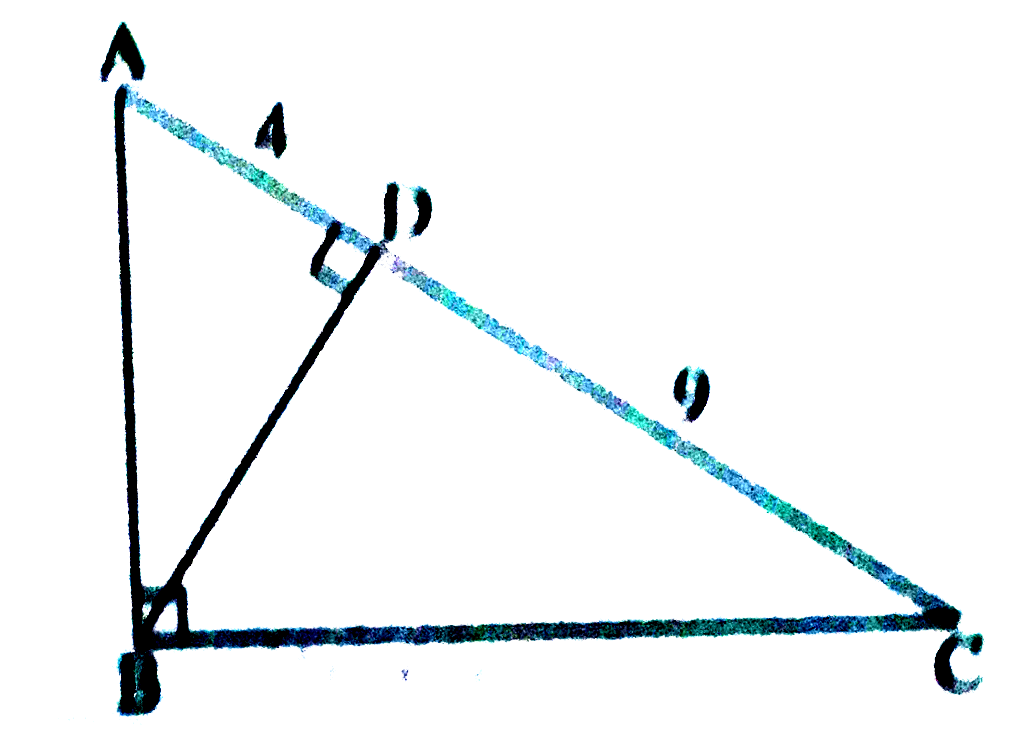
In right -angled △ABC,BD⊥AC.
If AD =4 DC =9 then find BD.
View Solution