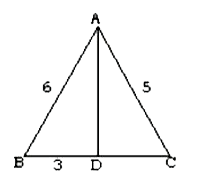
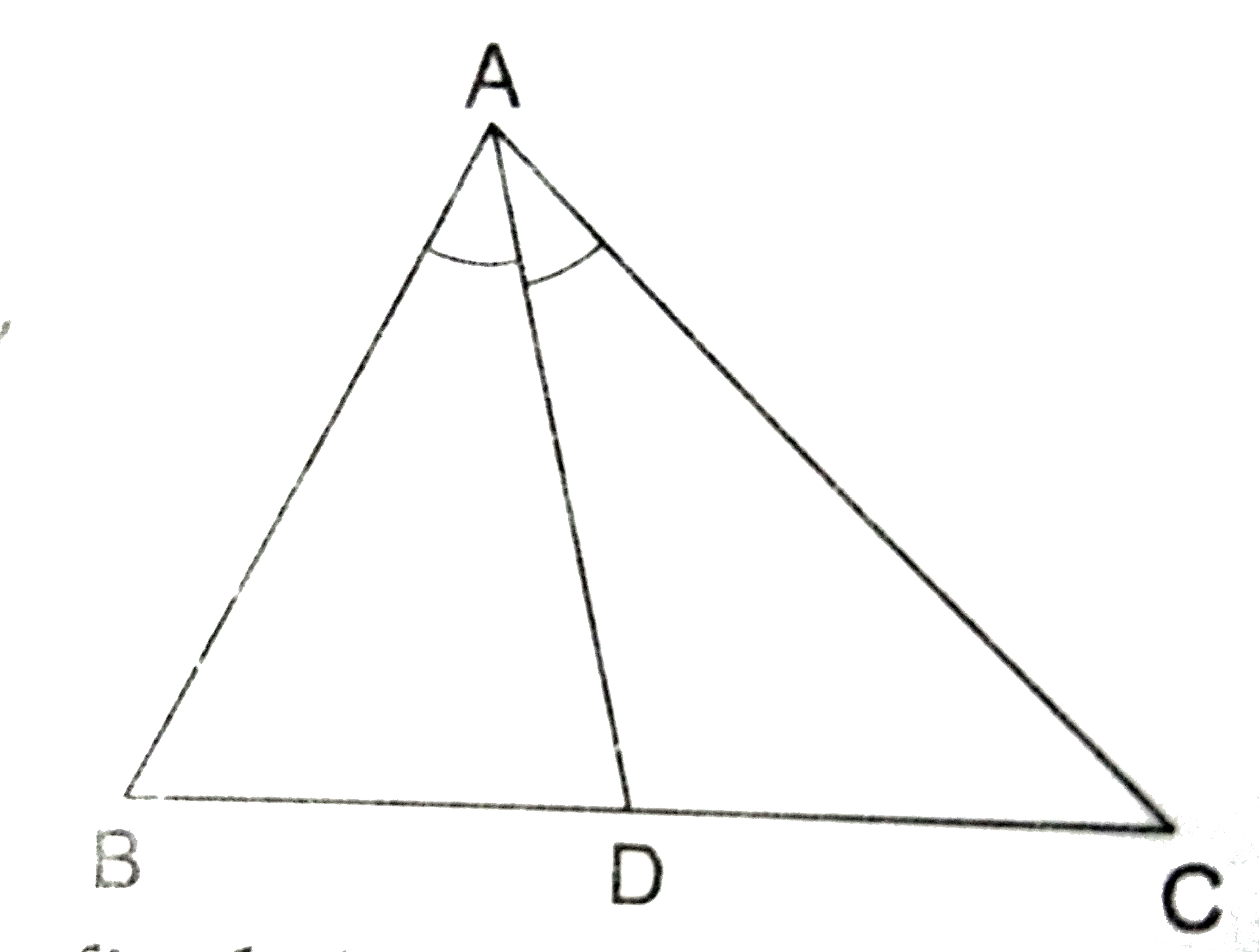
In the given figure, AD is the bisector of ∠BAC. If AB =10 cm , AC=6 cm and BC=12 cm, find BD and DC .
Video Solution
Text Solution
Let BD= x cm. then.
DC=(BC−BD)=(12−x)cm.
In ΔABC,AD is the bisector of ∠BAC.
So, by angle-bisector theorem, we have
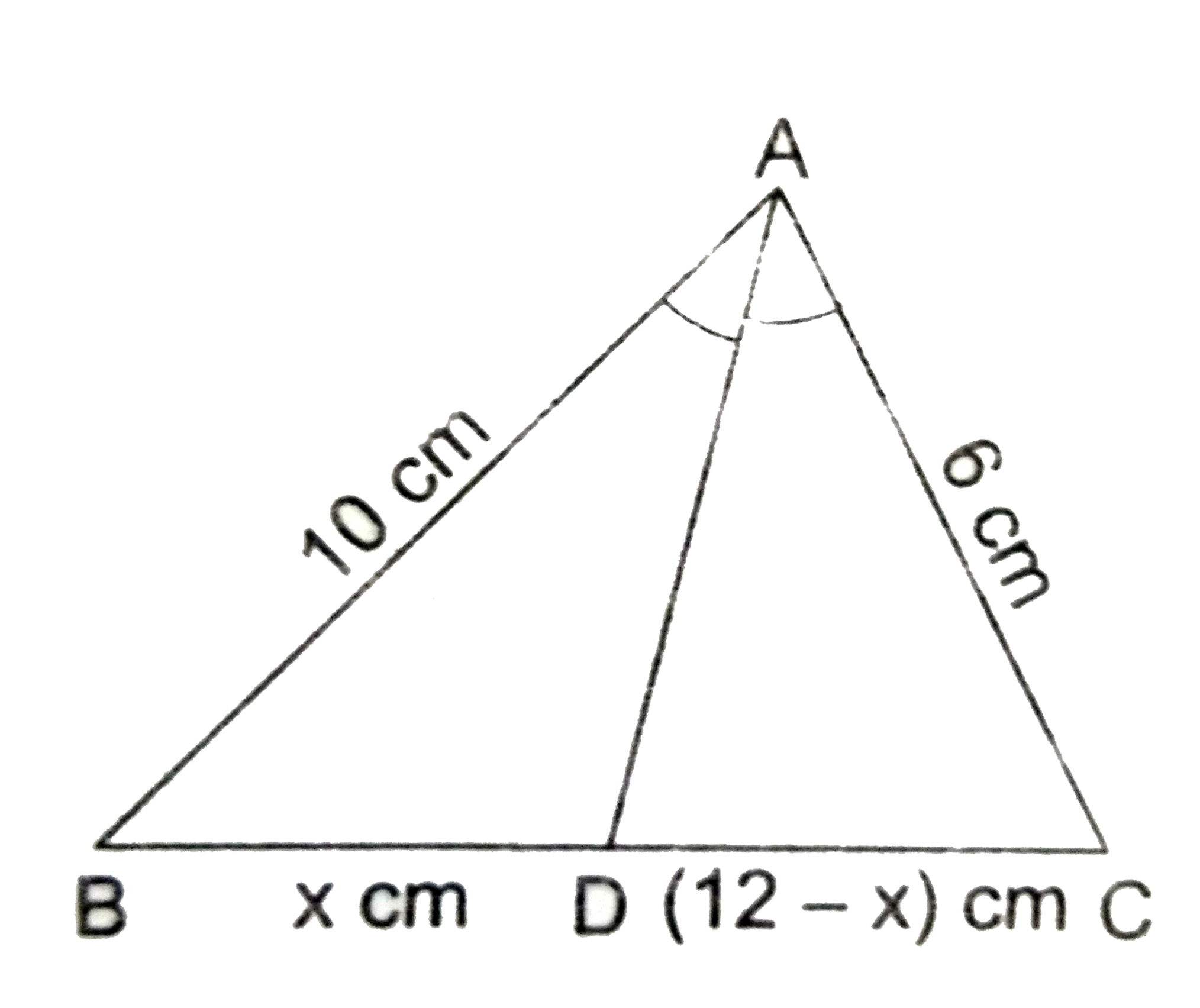
BDDC=ABAC⇒x12−x=106
⇒6x=10(12−x)
⇒16x=120⇒x=7.5
Hence, BD=7.5cm,andDC=(12−7.5)cm,=4.5cm
|
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLE
4 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A
1 Videos
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
Knowledge Check
In the given figure , Ad is the bisector of ∠BAC. If AB = 10 cm , AC = 14 cm and BC = 8 cm , find BD and DC respectively .
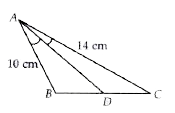 A4.7cm,3.3cmB3.3cm,4.7cmC4.3cm,3,5cmD3.5cm,4.3cm
A4.7cm,3.3cmB3.3cm,4.7cmC4.3cm,3,5cmD3.5cm,4.3cmIn the given figure , if AD is the bisector of ∠BAC . If AB=10 cm , AC = 14 cm and BC = 6 cm , then find BD and DC .
 A2.5 cm , 3.5 cmB3 cm , 3 cmC3.5 cm , 4.5 cmD4 cm , 2 cm
A2.5 cm , 3.5 cmB3 cm , 3 cmC3.5 cm , 4.5 cmD4 cm , 2 cmIn figure AD is the bisector of ∠BAC. If BD=2cm,CD=3cmandAB=5cm. Find AC :
 A6 cmB7.5 cmC10 cmD15 cm
A6 cmB7.5 cmC10 cmD15 cm
In the figure, AD is the bisector of angle BAC. Prove that AB>BD.
View SolutionIn the figure , AD is bisector of ˆA . If BC = 10 cm , BD = 6 cm , and AC = 6 cm, find AB.
View SolutionIn Fig. 4.42, AD is the bisector of ∠BAC . If AB=10cm . AC=14cm and BC=6cm , find BD and DC . (FIGURE)
View SolutionIn a ΔABC,AD is the bisector of ∠A.
(i) If AB=6.4cm,AC=8cmandBD=5.6cm, find DC.
(ii) If AB=10cm,AC=14cmandBC=6cm, find BD and DC.
(iii) If AB=5.6cm,BD=3.2cm,andBC=6cm, find AC.
(iv) If AB=5.6cm,AC=4cm,andDC3cm, find BC.
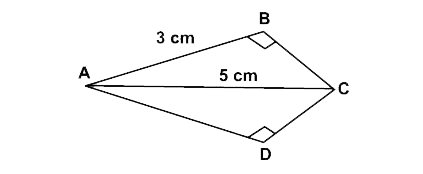
View SolutionIn the given figure AC is bisector of ∠BAD, AB=3cm,AC=5cm, then AD= ______
View Solution