Let f:[0, ∞)→R be a continuous function such that f(x)=1−2x+∫x0ex−tf(t)dt for all x∈[0, ∞) . Then, which of the following statement(s) is (are) TRUE? The curve y=f(x) passes through the point (1, 2) (b) The curve y=f(x) passes through the point (2, −1) (c) The area of the region {(x, y)∈[0, 1]×R:f(x)≤y≤√1−x2} is π−24 (d) The area of the region {(x, y)∈[0, 1]×R:f(x)≤y≤√1−x2} is π−14
(a) The curve y=f(x) passes through the point (1,2)
(b) The curve y=f(x) passes through the point (2, -1)
(c) the area of the region
{(x,y)∈[0,1]×R:f(x)≤y≤√1−x2}isπ−24
(d) The area of the region
{(x,y)∈[0,1]×R:f(x)≤y≤√1−x2}isπ−14
The correct Answer is:(b,c)
we have ,
f(x)=1−2x+∫x0ex−tf(t)dt
On multiplying e^(-x) both sides, we get
e−xf(x)=e−x−2xe−x+∫x0e−tf(t)dt
On differentiating both side w.r.t.x, we get
e−xf'(x)−e−xf(x)=−e−x−2xe−x+2xe−x+e−xf(x)
⇒f'(x)−2f(x)=2x−3
[dividing both sides by e^(-x)]
Let f(x)=y
⇒f'(x)=dydx
∴dydx−2y=2x−3
which is linear differential equation of the form
dydx+Py=Q.Here,P=−2andQ=2x−3.
Now, IF=e∫Pdx=e∫−2dx=e−2x
∴ Solution of the given differential equation is
y⋅e−2x=∫(2x−3)e−2xdx+C
y⋅e−2x=−(2x−3)⋅e−2x2+2∫e−2x2dx+C
[by using integration by parts]
⇒y⋅e−2x=−(2x−3)e−2x2−e−2x2+C
⇒y=(1−x)+Ce2x
On putting x = 0 anfd y = 1, we get
1=1+C⇒C=0
∴y=1−x
y=1-x passes through (2,-1)
Now, area of region bounded by curve y=√1−x2 and
y=1-x is shows as
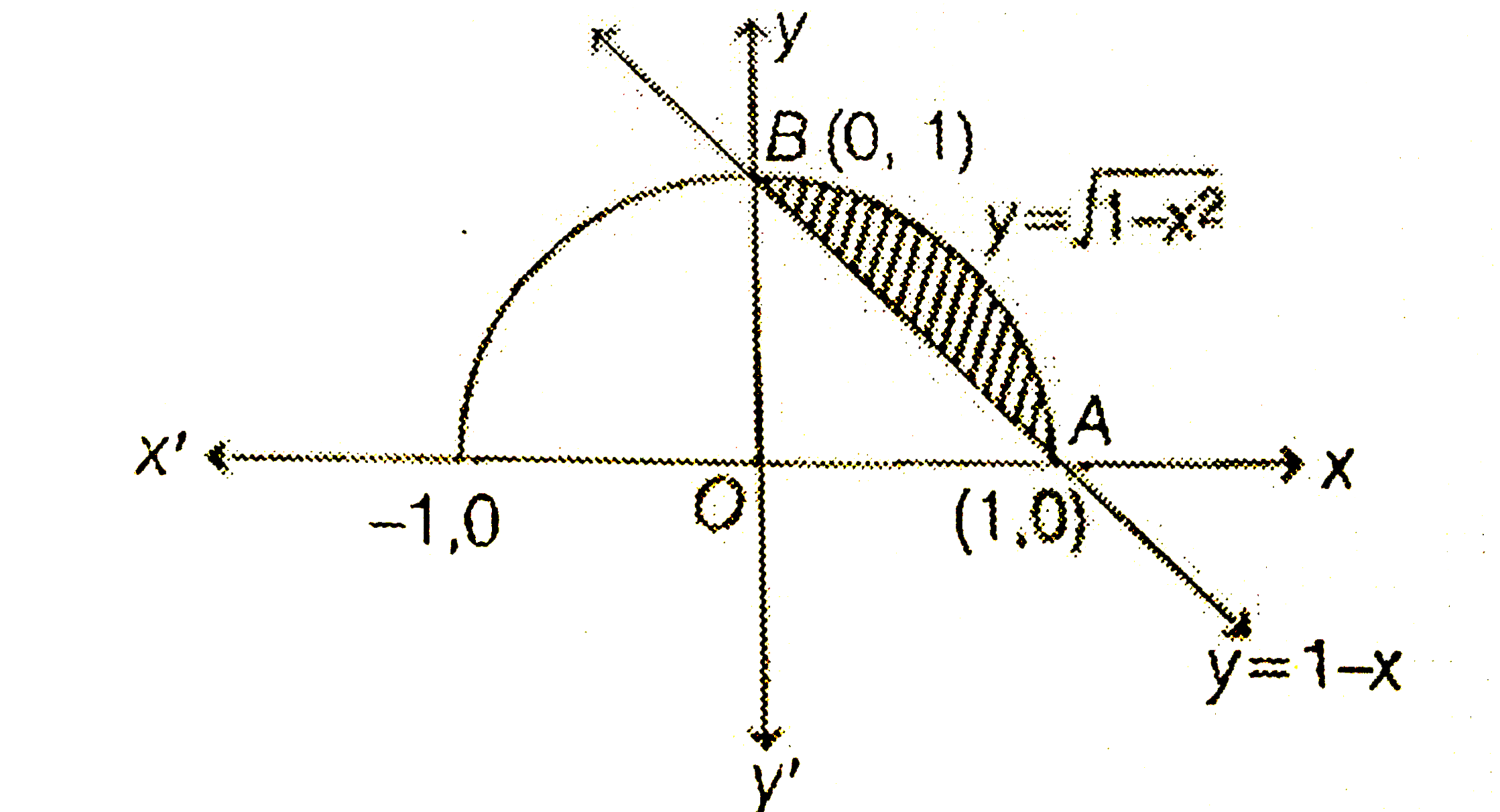
∴ Area of shaded region
= Area of 1st quadrant of a
ciralc-Area of ΔOAB
=π4(1)2−12×1×1
=π4−12=π−24
Hencee, options b and c are correct.
Topper's Solved these Questions
- DIFFERENTIAL EQUATIONS BOOK - IIT JEE PREVIOUS YEARCHAPTER - DIFFERENTIAL EQUATIONS EXERCISE - TOPIC 1 SOLUTION OF DIFFERENTIAL EQUATIONS BY VARIABLE <br> SEPARATION METHOD <br>OBJECTIVE QUESTIONS II (ONE OR MORE THAN ONE CORRECT OPTION)
7 Videos
- DIFFERENTIAL EQUATIONS BOOK - IIT JEE PREVIOUS YEARCHAPTER - DIFFERENTIAL EQUATIONS EXERCISE - TOPIC 1 SOLUTION OF DIFFERENTIAL EQUATIONS BY VARIABLE <br> SEPARATION METHOD <br> NUMERICAL VALUE
2 Videos
- DIFFERENTIAL EQUATIONS BOOK - IIT JEE PREVIOUS YEARCHAPTER - DIFFERENTIAL EQUATIONS EXERCISE - TOPIC 3 APPLICATIONS OF HOMOGENEOUS DIFFERENTIAL EQUATIONS <br> ANALYTICAL & DESCRIPTIVE QUESTIONS
7 Videos
- DEFINITE INTEGRATIONBOOK - IIT JEE PREVIOUS YEARCHAPTER - DEFINITE INTEGRATIONEXERCISE - LIMITS AS THE SUM
6 Videos
- ELLIPSEBOOK - IIT JEE PREVIOUS YEARCHAPTER - ELLIPSEEXERCISE - EQUATION OF CHORD OF CONTACT, CHORD BISECTED AT A GIVEN POINT & DIAMETER
3 Videos
Similar Questions
Knowledge Check
Let f:[0,∞)→R be a continuous function such that f(x)=1−2x+∫x0ex−tf(t)dt for all x∈[0,∞). Then, which of the following statements(s) is (are)) TRUE?
AThe curve y=f(x) passes through the point (1,2)BThe curve y=f(x) passes through the point (2,-1)CThe area of the region {(x,y)∈[0,1]×R:f(x)≤y≤√1−x2} is π−24DThe area of the region {(x,y)∈[0,1]×R:f(x)≤y≤√1−x2} is π−14If f (x) is the anti-derivative of tan−1√x such that the curve y = f(x) passes through the point (0,2) then f(x) =
Axtan−1√x−√x+tan−1√x+2Bxtan−1√x+√x+tan−1√x+2Cxtan−1√x+2DNone of theseThe slope of the tangent to a cruve y=f(x) at (x,f(x)0 is 2x+1.If the curve passes through the point (1,2) then the area of the region bounded by the curve,the x-axis and the lien x=1 is
A56 sq. unitsB65 sq.unitsC16 sq. unitsD6 sq. units
If f'(x)=x−1, the equation of a curve y=f(x) passing through the point (1, 0) is given by
View SolutionLet f(x) be a continuous function such that f(a−x)+f(x)=0 for all x∈[0,a]. Then ∫a0dx1+ef(x)= (A) a (B) a2 (C) 12f(a) (D) none of these
View SolutionLet f be a continuous function on (0,∞) and satisfying f(x)=(logex)2−∫e1f(t)tdt for all x≥1, then f(e) equals
View SolutionLet f(x) be continuous function such that f(0)=1,f(x)−f(x7)=x7∀x∈R. The area bounded by the curve y=f(x) and the coordinate axes is
View SolutionLet f :(0,∞) R be a continuous, strictly increasing function such that f3(x)=∫x0t f2(t)dt. If a normal is drawn to the curve y = f(x) with gradient −12 , then find the intercept made by it on the y-axis is 5 (b) 7 (c) 9 (d) 11
View Solution