Let p(x)=x3−x+1andg(x)=2−3x, Check whether p(x) is a multiple of g (x) or not .
g(x)=0⇒2−3x=0⇒3x=2⇒x=23.
By the remainder theorem , we know that when p (x) is divided by (2−3x) then the remainder is p(23).
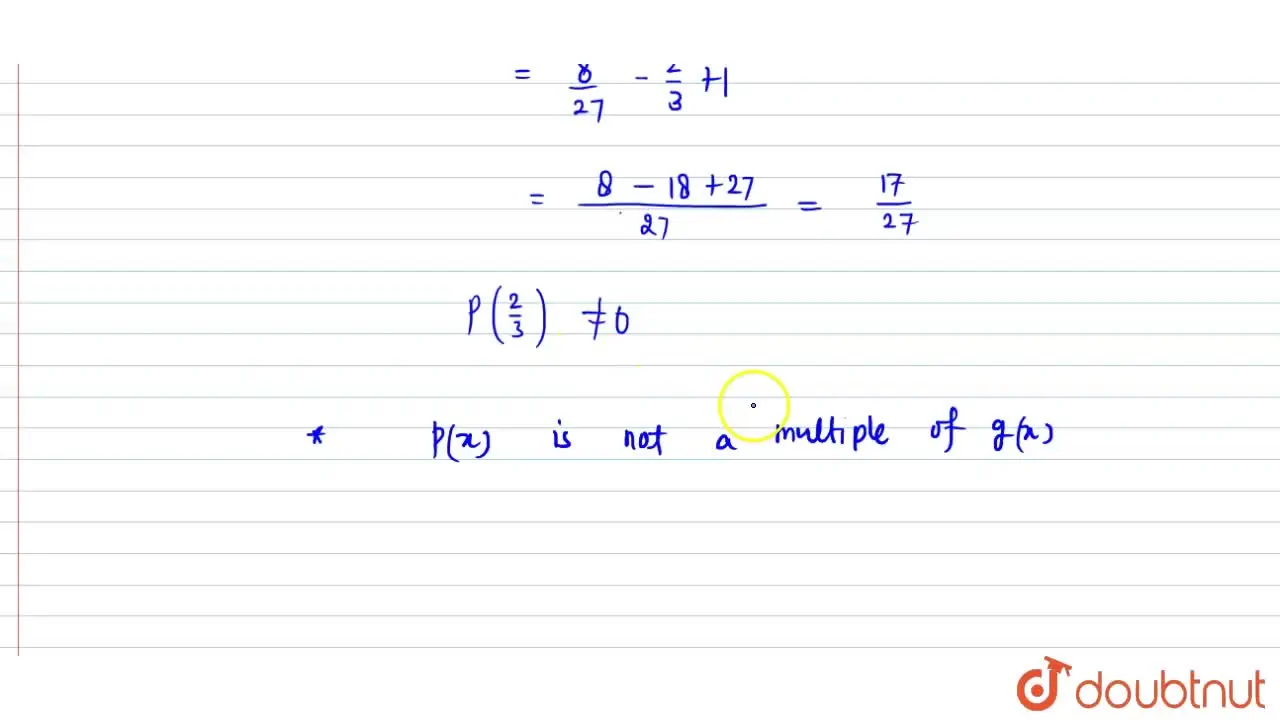
And , p(23)={(23)3−(23)+1}=(827−23+1)
=(8−18+2727)=1727≠0.
Thus , when p (x) is divided by g (x) , the remainder is nonzero. Hence , p(x) is not a multiple of g (x).
Related Playlists
- BOOK - RS AGGARWALCHAPTER - POLYNOMIALSEXERCISE - Exercise 2A6videos
POLYNOMIALS
- BOOK - RS AGGARWALCHAPTER - POLYNOMIALSEXERCISE - Exercise 2B9videos
POLYNOMIALS
- BOOK - RS AGGARWALCHAPTER - NUMBER SYSTEMSEXERCISE - Very Short Answer Questions23videos
NUMBER SYSTEMS
- BOOK - RS AGGARWALCHAPTER - PRESENTATION OF DATA IN TABULAR FORMEXERCISE - Multiple Choice Questions (Mcq)9videos
PRESENTATION OF DATA IN TABULAR FORM
Similar Questions
If p(x)=x3−5x2+4x−3andg(x)=x−2 show that p(x) is not a multiple of g(x).
View SolutionIf p(x)=8x3−6x2−4x+3andg(x)=x3−14 then check whether g (x) is a factor of p(x) or not.
View SolutionIf p(x)=2x3−11x2−4x+5andg(x)=2x+1, show that g(x) is not a factor of p(x).
View SolutionLet p(x)=x4−3x2+2x+5. Find the remainder when p(x) is divided by (x−1).
View SolutionIf: f(x)=xx+1andg(x)=x1−x, then: (f∘g)(x)=
View SolutionIf the function f(x)=x3+ex/2andg(x)=f−1(x), then the value of g'(1) is
View SolutionLet fori 1,2,3,pi(x) be a polynomial ofdegree 2 in x1pi(x) and pi(x) be the first and second order derivatives of pi(x) respectively.Let, A(x)=⎡⎢⎣p1(x)p'1(x)p''1(x)p2(x)p'2(x)p''2(x)p3(x)p'3(x)p''3(x)⎤⎥⎦and B(x)=[A(x)]TA(x), then Determinant of B(x): (A) Is a Polynomial of degree 6 (B) Is a Polynomial of degree 4 (C) Is a Polynomial of degree 2 (D) Does not depend on x
View SolutionCheck whether q(x) is a multiple of r(x) or not.
Where q(x)=2x3−11x2−4x+5,r(x)=2x+1View SolutionLet P(x)=x7−3x5+x3−7x2+5 and q(x)=x−2. The remainder if p(x) is divided by q(x) is
View SolutionCheck whether x=−1 is a zero of the polynomial p(x)=x3−3x+1
View Solution