Three equal circles, each of radius 6 cm, touch one another as shown in the figure. Find the area enclosed between them.
[Take π=3.14 and √3=1.732]
![Three equal circles, each of radius 6 cm, touch one another as shown in the figure. Find the area enclosed between them. [Take pi=3.14 and sqrt(3)=1.732]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/RSA_MATH_X_C16_E01_039_Q01.png)
The correct Answer is:5.76cm2
Let A,B,C be the centres of these circles.
Join AB,BC and CA.
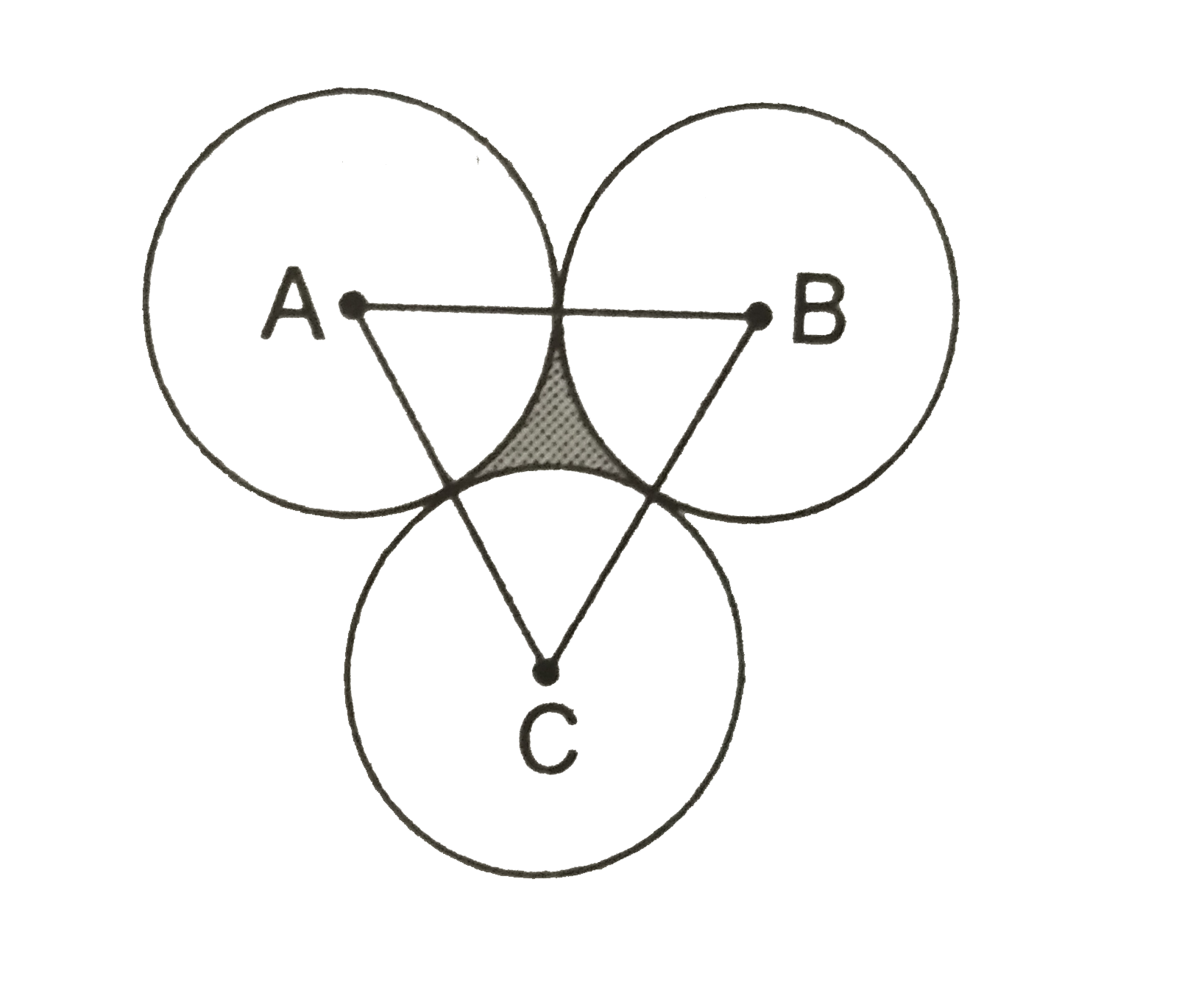
Required area = (area of the Δ ABC with each side a=12 cm)
−3(area of a sector with r=6cm,θ=60∘)
Topper's Solved these Questions
- AREA OF CIRCLE, SECTOR AND SEGMENT Book:RS AGGARWALChapter:AREA OF CIRCLE, SECTOR AND SEGMENT Exercise:EXERCISE
Explore 1 Video
- AREA OF CIRCLE, SECTOR AND SEGMENT Book:RS AGGARWALChapter:AREA OF CIRCLE, SECTOR AND SEGMENT Exercise:Exercise 16B
Explore 51 Videos
- AREA OF CIRCLE, SECTOR AND SEGMENT Book:RS AGGARWALChapter:AREA OF CIRCLE, SECTOR AND SEGMENT Exercise:SOLVED EXAMPLE_TYPE
Explore 1 Video
- ARITHMETIC PROGRESSIONBook:RS AGGARWALChapter:ARITHMETIC PROGRESSIONExercise:Multiple Choice Questions (Mcq)
Explore 30 Videos
Similar Questions
Knowledge Check
Four equal circles each of radius 'a' units touch one another. The area enclose between them (π=227), in square units, is
A3a2B6a27C41a27Da27Three equal circles of unit radius touch each other. Then, the area of the circles is
Aπ3(2+√3)3B2π3(2+√3PP2)C6π(2+√3)2D16π(2+√3)2
Three circles each of radius 3.5 cm touch one another. The area subtended between them is
View SolutionThree congruent circles each of radius 4 cm touch one another. What is the area (in cm)2 of the portion included between them?
View SolutionThree equal circles of radius unity touches one another. Radius of the circle touching all the three circles is:
View SolutionA chord of a circle of radius 30 cm makes an angle of 60∘ at the centre of the circle. Find the areas of the major segment. [Take π=3.14 and √3=1.732]
View SolutionThree circles each of radius 7cm are drawn in such a way that each of them touches the other two. Find the area enclosed between the circles.
View SolutionA chord of a circle of radius 30 cm makes an angle of 60∘ at the centre of the circle. Find the areas of the minor and major segments. [Take π=314 and √3=1.732]
View Solution